Difference between revisions of "Arctan"
(→Properties) |
|||
| Line 3: | Line 3: | ||
<div align="center"> | <div align="center"> | ||
<gallery> | <gallery> | ||
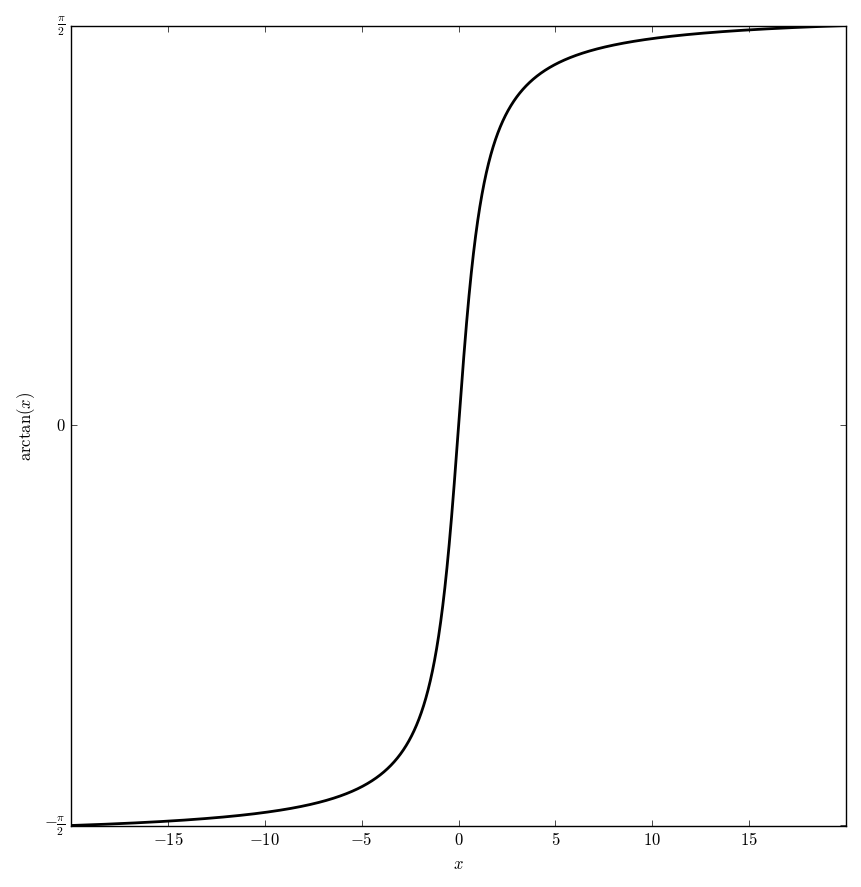
| − | File: | + | File:Arctanplot.png|Graph of $\mathrm{arctan}$ on $[-20,20]$. |
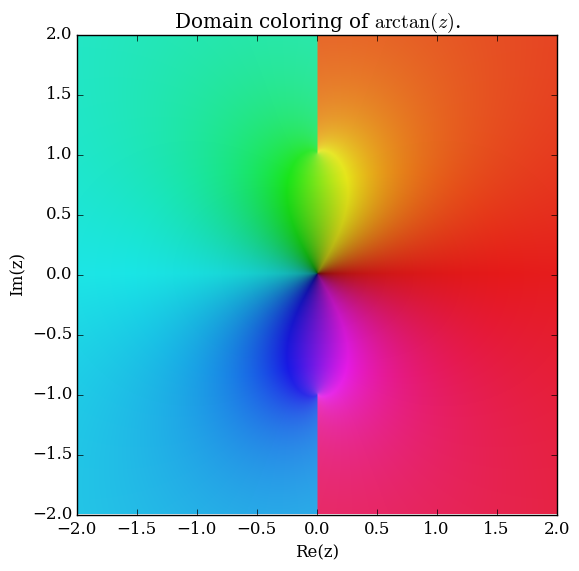
| − | File:Complexarctanplot.png|[[Domain coloring]] of $\mathrm{arctan}$ | + | File:Complexarctanplot.png|[[Domain coloring]] of $\mathrm{arctan}$. |
</gallery> | </gallery> | ||
</div> | </div> | ||
| Line 10: | Line 10: | ||
=Properties= | =Properties= | ||
{{:Derivative of arctan}} | {{:Derivative of arctan}} | ||
| − | + | {{:Antiderivative of arctan}} | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
<div class="toccolours mw-collapsible mw-collapsed"> | <div class="toccolours mw-collapsible mw-collapsed"> | ||
Revision as of 04:15, 16 May 2016
The $\mathrm{arctan}$ function is the inverse function of the tangent function.
Domain coloring of $\mathrm{arctan}$.
Contents
Properties
Theorem
The following formula holds: $$\dfrac{\mathrm{d}}{\mathrm{d}z} \mathrm{arctan}(z) = \dfrac{1}{z^2+1},$$ where $\mathrm{arctan}$ denotes the inverse tangent function.
Proof
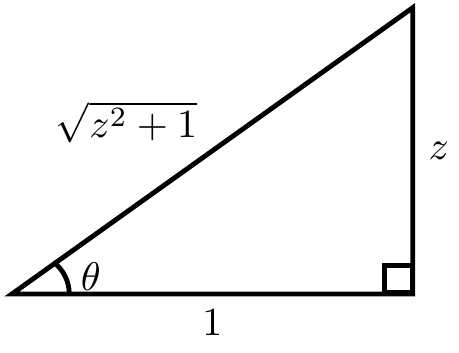
If $\theta=\mathrm{arctan}(z)$ then $\tan \theta = z$. Now implicit differentiation with respect to $z$ yields $$\sec^2(\theta)\theta'=1.$$ The following triangle shows that $\sec^2(\mathrm{arctan}(z))=z^2+1$:
Substituting back in $\theta=\mathrm{arccos(z)}$ yields the formula $$\dfrac{\mathrm{d}}{\mathrm{d}z} \mathrm{arccos(z)} = \dfrac{1}{\sec^2(\mathrm{arctan(z)})} = \dfrac{1}{z^2+1},$$ as was to be shown. █
References
Theorem
The following formula holds: $$\displaystyle\int \mathrm{arctan}(z) \mathrm{d}z = z\mathrm{arctan}(z) - \dfrac{1}{2}\log \left(z^2+1 \right)+C,$$ where $\mathrm{arctan}$ denotes the inverse tangent and $\log$ denotes the logarithm.
Proof
Let $u=\mathrm{arctan}(z)$ and $\mathrm{d}v=1$. Then $v=z$ and because of the derivative of arctan, $\mathrm{d}u=\dfrac{1}{z^2+1} \mathrm{d}z$. Therefore using integration by parts, $$\displaystyle\int \mathrm{arctan}(z) \mathrm{d}z = z \mathrm{arctan}(z) - \displaystyle\int \dfrac{z}{z^2+1} \mathrm{d}z.$$ To evaluate the remaining integral, let $w=z^2+1$ so that $\mathrm{d}w=2z \mathrm{d}z$, i.e. $\dfrac{1}{2} \mathrm{d} w = z \mathrm{d}z$ and we see using the derivative of the logarithm, $$\displaystyle\int \mathrm{arctan}(z) \mathrm{d}z = z \mathrm{arctan}(z) - \dfrac{1}{2}\displaystyle\int \dfrac{1}{w} \mathrm{d}w = z \mathrm{arctan}(z) - \dfrac{1}{2} \log(z^2+1) + C,$$ as was to be shown.
References
Proposition: $\mathrm{arctan}(z) = \mathrm{arccot}\left( \dfrac{1}{z} \right)$
Proof: █
Relationship between arctan and hypergeometric 2F1