Difference between revisions of "Sech"
| Line 5: | Line 5: | ||
<gallery> | <gallery> | ||
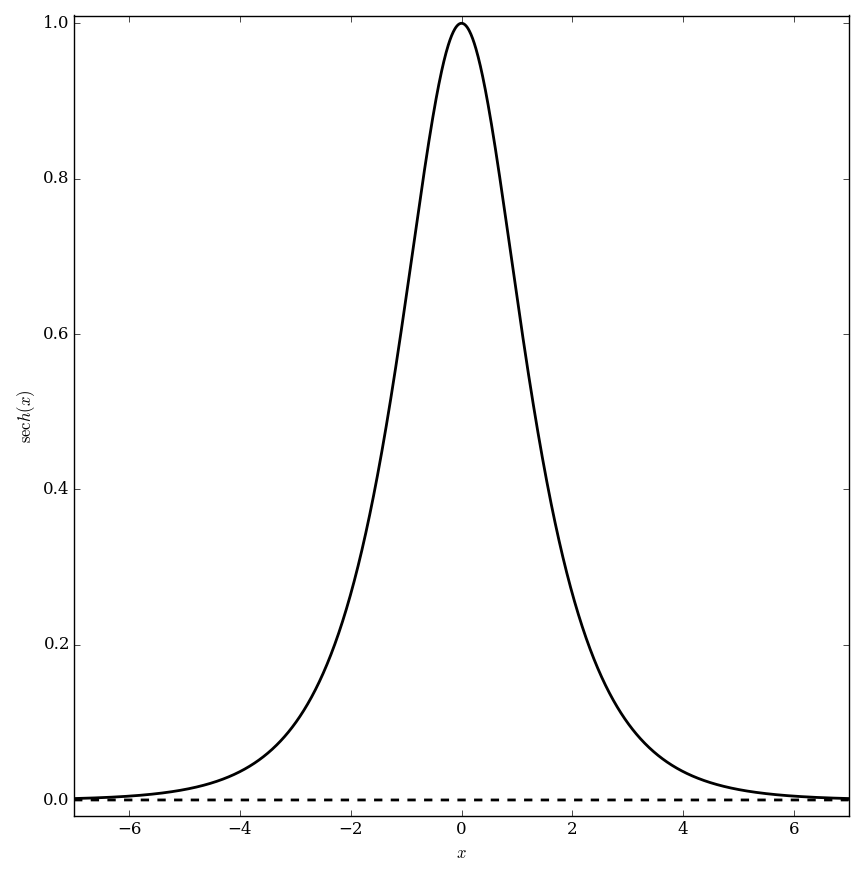
File:Sechplot.png|Graph of $\mathrm{sech}$ on $[-5,5]$. | File:Sechplot.png|Graph of $\mathrm{sech}$ on $[-5,5]$. | ||
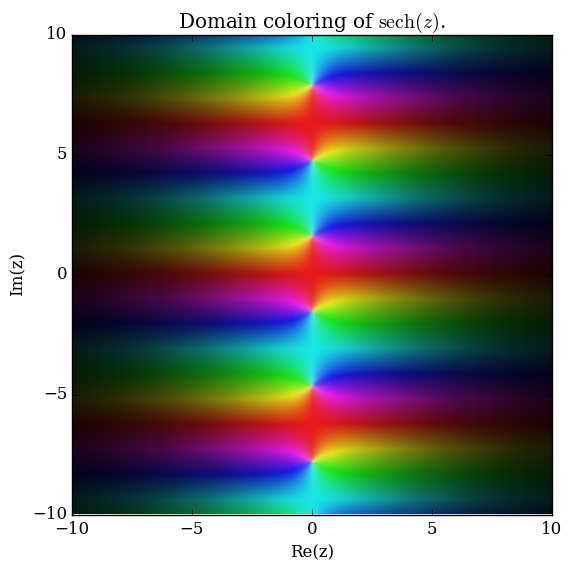
| − | File: | + | File:Complexsechplot.png|[[Domain coloring]] of [[analytic continuation]] of $\mathrm{sech}$. |
</gallery> | </gallery> | ||
</div> | </div> | ||
Revision as of 08:26, 16 May 2016
The hyperbolic secant function is defined by $$\mathrm{sech}(z)=\dfrac{1}{\cosh(z)}.$$
Domain coloring of analytic continuation of $\mathrm{sech}$.
Contents
Properties
Theorem
The following formula holds: $$\dfrac{\mathrm{d}}{\mathrm{d}z} \mathrm{sech}(z)=-\mathrm{sech}(z)\mathrm{tanh}(z),$$ where $\mathrm{sech}$ denotes the hyperbolic secant and $\mathrm{tanh}$ denotes the hyperbolic tangent.
Proof
From the definition, $$\mathrm{sech}(z) = \dfrac{1}{\mathrm{cosh}(z)}.$$ Using the quotient rule, the derivative of cosh, and the definition of $\mathrm{tanh}$, we see $$\begin{array}{ll} \dfrac{\mathrm{d}}{\mathrm{d}z} \mathrm{sech}(z) &= \dfrac{0-\sinh(z)}{\cosh(z)^2} \\ &=-\mathrm{sech}(z)\mathrm{tanh}(z), \end{array}$$ as was to be shown.
References
Theorem
The following formula holds: $$\displaystyle\int \mathrm{sech}(z) \mathrm{d}z=\arctan(\sinh(z)) + C,$$ where $\mathrm{sech}$ denotes the hyperbolic secant, $\arctan$ denotes the inverse tangent, and $\sinh$ denotes the hyperbolic sine.
Proof
References
Theorem
The following formula holds: $$\cos(\mathrm{gd}(x))=\mathrm{sech}(x),$$ where $\cos$ denotes the cosine, $\mathrm{gd}$ denotes the Gudermannian, and $\mathrm{sech}$ denotes the hyperbolic secant.
Proof
References
Theorem
The following formula holds: $$\mathrm{sech}(\mathrm{gd}^{-1}(x))=\cos(x),$$ where $\mathrm{sech}$ is the hyperbolic secant, $\mathrm{gd}^{-1}$ is the inverse Gudermannian, and $\cos$ is the cosine.