Difference between revisions of "Logarithmic integral"
From specialfunctionswiki
| Line 11: | Line 11: | ||
=Properties= | =Properties= | ||
| − | + | [[Relationship between logarithmic integral and exponential integral]]<br /> | |
| − | + | [[Prime number theorem, logarithmic integral]]<br /> | |
=See Also= | =See Also= | ||
Revision as of 08:08, 8 June 2016
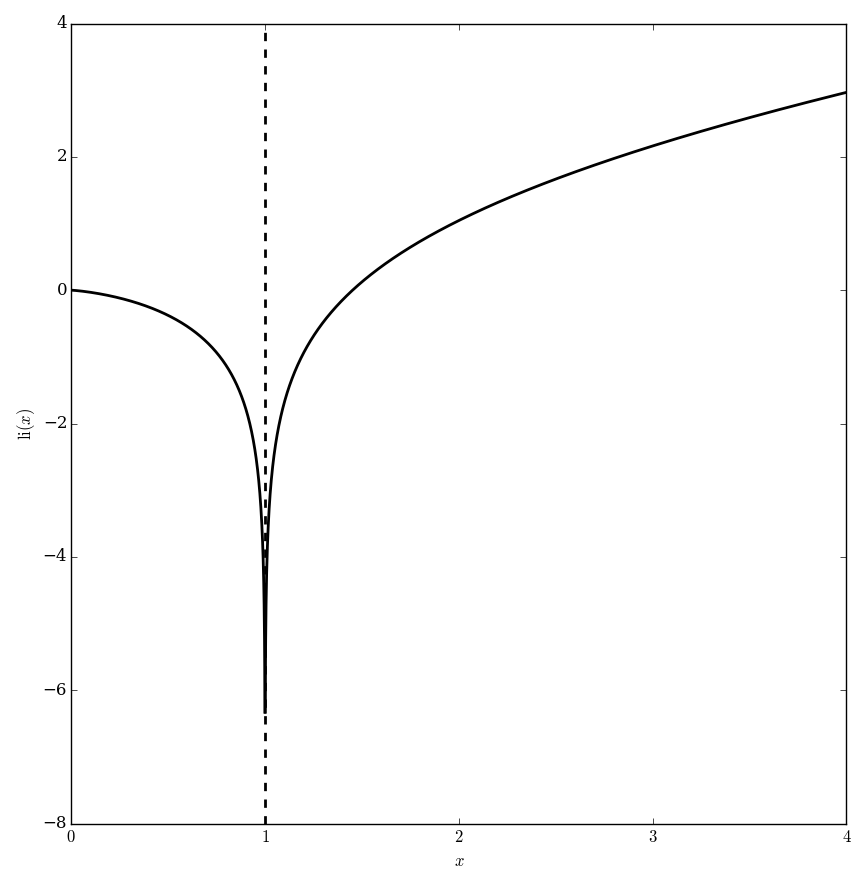
The logarithmic integral is $$\mathrm{li}(x) = \displaystyle\int_0^x \dfrac{1}{\log(t)} \mathrm{d}t,$$ where $\log$ denotes the logarithm.
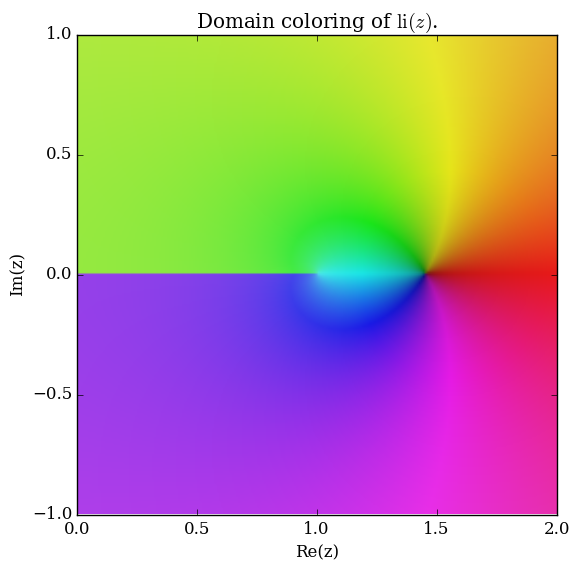
Domain coloring of $\mathrm{li}$.
Properties
Relationship between logarithmic integral and exponential integral
Prime number theorem, logarithmic integral