Difference between revisions of "Scorer Hi"
From specialfunctionswiki
(→Properties) |
|||
| (3 intermediate revisions by the same user not shown) | |||
| Line 1: | Line 1: | ||
The Scorer $\mathrm{Hi}$ function is a solution of the [[differential equation]] $y''(x)-x y(x)=\dfrac{1}{\pi}$ and may be defined by the formula | The Scorer $\mathrm{Hi}$ function is a solution of the [[differential equation]] $y''(x)-x y(x)=\dfrac{1}{\pi}$ and may be defined by the formula | ||
| − | $$\mathrm{Hi}(x)=\dfrac{1}{\pi} \displaystyle\int_0^{\infty} \exp \left( -\dfrac{t^3}{3}+xt \right) | + | $$\mathrm{Hi}(x)=\dfrac{1}{\pi} \displaystyle\int_0^{\infty} \exp \left( -\dfrac{t^3}{3}+xt \right)\mathrm{d}t.$$ |
| + | |||
| + | <div align="center"> | ||
| + | <gallery> | ||
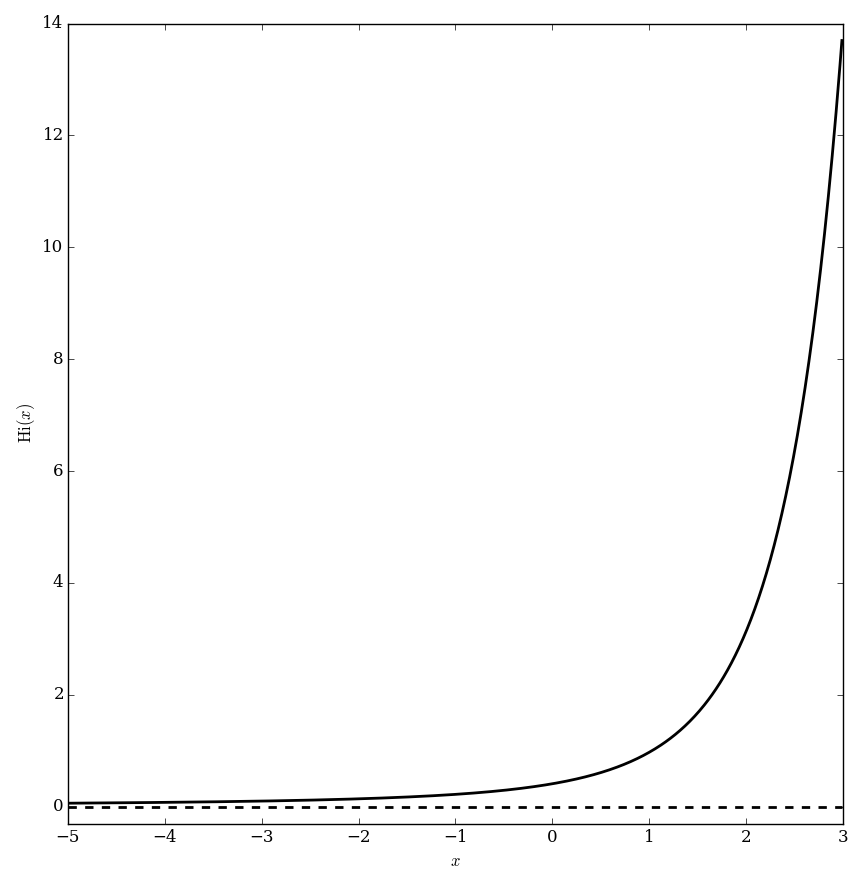
| + | File:Scorerhiplot.png|Graph of $\mathrm{Hi}$. | ||
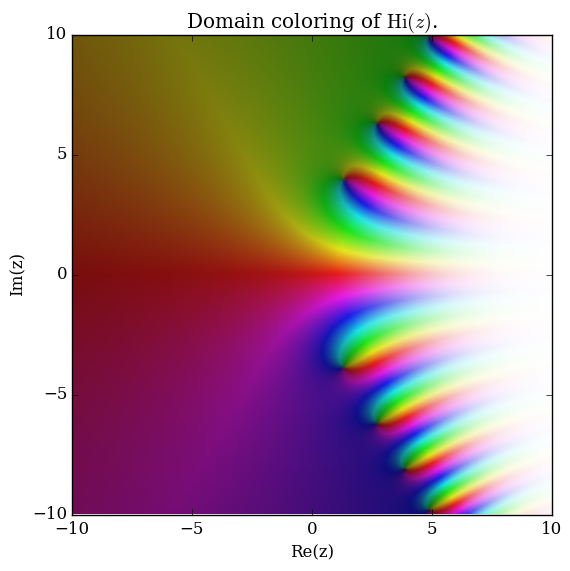
| + | File:Complexscorerhi.png|[[Domain coloring]] of $\mathrm{Hi}$. | ||
| + | </gallery> | ||
| + | </div> | ||
=Properties= | =Properties= | ||
| − | + | [[Relationship between Scorer Hi and Airy functions]]<br /> | |
=See Also= | =See Also= | ||
| Line 9: | Line 16: | ||
[[Airy Bi]]<br /> | [[Airy Bi]]<br /> | ||
[[Scorer Gi]]<br > | [[Scorer Gi]]<br > | ||
| + | |||
| + | [[Category:SpecialFunction]] | ||
Latest revision as of 23:00, 9 June 2016
The Scorer $\mathrm{Hi}$ function is a solution of the differential equation $y(x)-x y(x)=\dfrac{1}{\pi}$ and may be defined by the formula $$\mathrm{Hi}(x)=\dfrac{1}{\pi} \displaystyle\int_0^{\infty} \exp \left( -\dfrac{t^3}{3}+xt \right)\mathrm{d}t.$$
Domain coloring of $\mathrm{Hi}$.
Properties
Relationship between Scorer Hi and Airy functions