Difference between revisions of "Erfc"
From specialfunctionswiki
| (7 intermediate revisions by the same user not shown) | |||
| Line 1: | Line 1: | ||
The complementary error function $\mathrm{erfc}$ is defined by the formula | The complementary error function $\mathrm{erfc}$ is defined by the formula | ||
| − | $$\mathrm{erfc}( | + | $$\mathrm{erfc}(z)=1-\mathrm{erf}(z),$$ |
where $\mathrm{erf}$ denotes the [[error function]]. | where $\mathrm{erf}$ denotes the [[error function]]. | ||
<div align="center"> | <div align="center"> | ||
<gallery> | <gallery> | ||
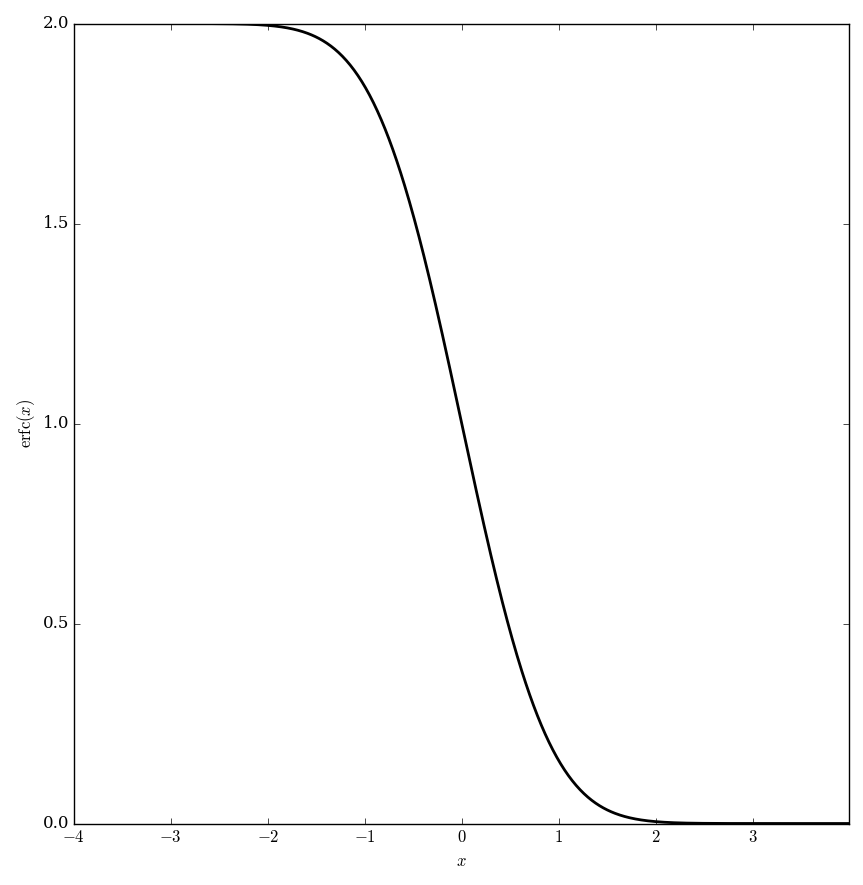
| − | File: | + | File:Erfcplot.png|Graph of $\mathrm{erfc}$. |
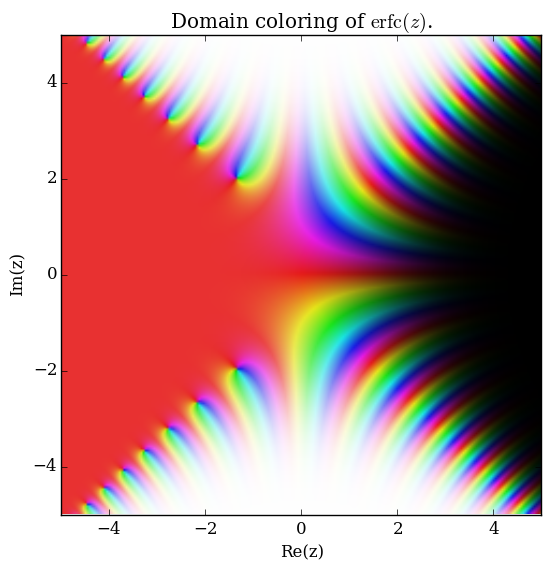
| + | File:Complexerfcplot.png|[[Domain coloring]] of $\mathrm{erfc}$. | ||
</gallery> | </gallery> | ||
</div> | </div> | ||
| + | |||
| + | ==References== | ||
| + | * {{BookReference|Handbook of mathematical functions|1964|Milton Abramowitz|author2=Irene A. Stegun|prev=Error function|next=findme}}: 7.1.2 | ||
| + | |||
| + | {{:Error functions footer}} | ||
| + | |||
| + | [[Category:SpecialFunction]] | ||
Latest revision as of 21:56, 6 July 2016
The complementary error function $\mathrm{erfc}$ is defined by the formula $$\mathrm{erfc}(z)=1-\mathrm{erf}(z),$$ where $\mathrm{erf}$ denotes the error function.
Domain coloring of $\mathrm{erfc}$.
References
- 1964: Milton Abramowitz and Irene A. Stegun: Handbook of mathematical functions ... (previous) ... (next): 7.1.2