Difference between revisions of "Arctan"
From specialfunctionswiki
| (20 intermediate revisions by the same user not shown) | |||
| Line 1: | Line 1: | ||
| + | __NOTOC__ | ||
The $\mathrm{arctan}$ function is the inverse function of the [[tangent]] function.<br /> | The $\mathrm{arctan}$ function is the inverse function of the [[tangent]] function.<br /> | ||
| − | [ | + | <div align="center"> |
| + | <gallery> | ||
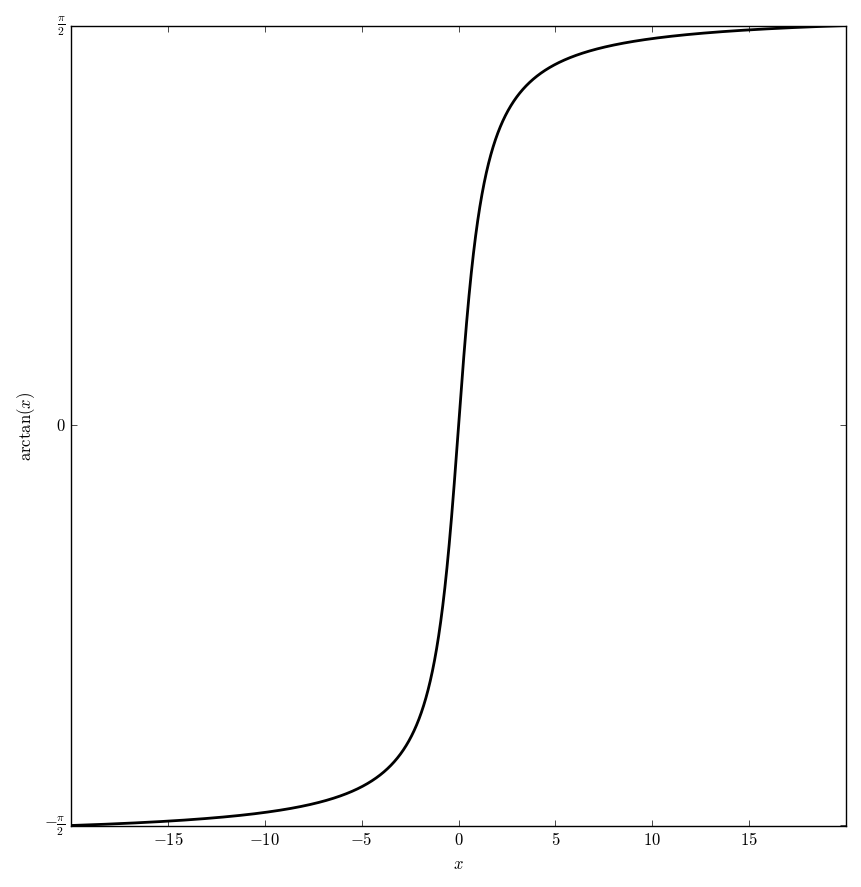
| + | File:Arctanplot.png|Graph of $\mathrm{arctan}$ on $[-20,20]$. | ||
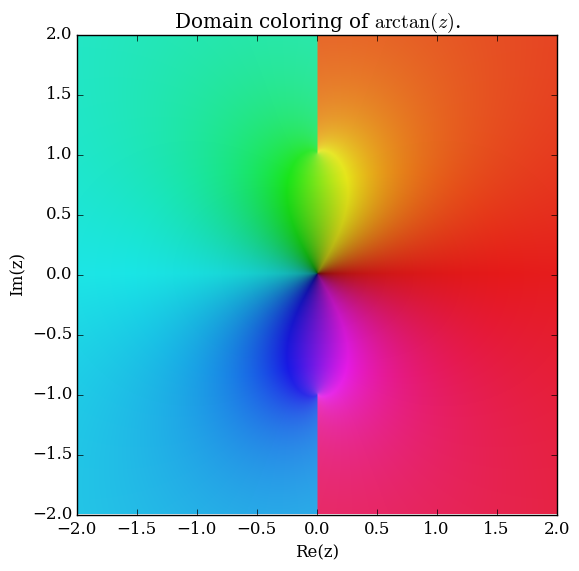
| + | File:Complexarctanplot.png|[[Domain coloring]] of $\mathrm{arctan}$. | ||
| + | </gallery> | ||
| + | </div> | ||
| − | [[ | + | =Properties= |
| + | [[Derivative of arctan]]<br /> | ||
| + | [[Antiderivative of arctan]]<br /> | ||
| + | [[Relationship between arctan and arccot]]<br /> | ||
| + | [[2F1(1/2,1;3/2;-z^2)=arctan(z)/z]]<br /> | ||
| − | = | + | =References= |
| − | + | [http://mathworld.wolfram.com/InverseTangent.html Weisstein, Eric W. "Inverse Tangent." From MathWorld--A Wolfram Web Resource. http://mathworld.wolfram.com/InverseTangent.html] | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | + | =See Also= | |
| − | < | + | [[Tangent]] <br /> |
| − | + | [[Tanh]] <br /> | |
| − | + | [[Arctanh]] | |
| − | < | ||
| − | |||
| − | |||
| − | + | {{:Inverse trigonometric functions footer}} | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | + | [[Category:SpecialFunction]] | |
| − | [ | ||
Latest revision as of 02:46, 16 September 2016
The $\mathrm{arctan}$ function is the inverse function of the tangent function.
Graph of $\mathrm{arctan}$ on $[-20,20]$.
Domain coloring of $\mathrm{arctan}$.
Properties
Derivative of arctan
Antiderivative of arctan
Relationship between arctan and arccot
2F1(1/2,1;3/2;-z^2)=arctan(z)/z
