Difference between revisions of "Thomae function"
(→Videos) |
|||
| Line 38: | Line 38: | ||
=Videos= | =Videos= | ||
| − | [https://www.youtube.com/watch?v= | + | [https://www.youtube.com/watch?v=Xu5Y6DqzN7Q Thomae Function by Bret Benesh (11 January 2012)]<br /> |
| − | [https://www.youtube.com/watch?v= | + | [https://www.youtube.com/watch?v=HeIU5lLtHyQ Thomae Function by Douglas Harder (19 April 2012)]<br /> |
=See also= | =See also= | ||
Revision as of 22:53, 3 November 2016
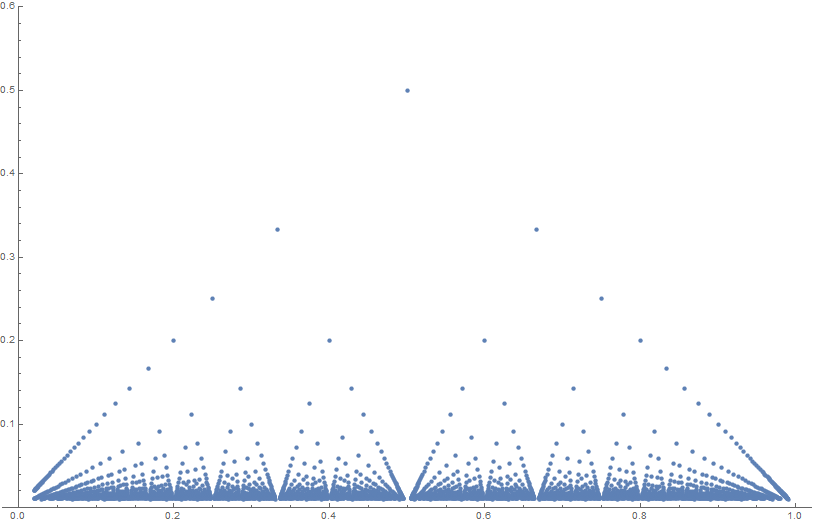
Thomae's function (sometimes called the popcorn function, raindrop function, Stars over Babylon) is given by the formula $$f(x) =\begin{cases} 1 & \text{if } x= 0 \\ \tfrac1{q} & \text{if } x = \tfrac{p}{q}\\ 0 & \text{if } x \in \mathbb{R}-\mathbb{Q}. \end{cases}$$
Properties
Theorem: The Thomae function is continuous at all irrational numbers and discontinuous at all rational numbers.
Proof: █
Theorem: The Thomae function has a (strict) local maximum at each rational number.
Proof: █
Theorem: The Thomae function $f(x)$ is Riemann integrable and $$\displaystyle\int_0^1 f(x) \mathrm{d}x = 0.$$
Proof: █
Videos
Thomae Function by Bret Benesh (11 January 2012)
Thomae Function by Douglas Harder (19 April 2012)
See also
Modifications of Thomae's Function and Differentiability