Difference between revisions of "Logarithmic integral"
From specialfunctionswiki
| Line 20: | Line 20: | ||
* {{BookReference|Handbook of mathematical functions|1964|Milton Abramowitz|author2=Irene A. Stegun|prev=Exponential integral Ei|next=Exponential integral E}}: $5.1.3$ | * {{BookReference|Handbook of mathematical functions|1964|Milton Abramowitz|author2=Irene A. Stegun|prev=Exponential integral Ei|next=Exponential integral E}}: $5.1.3$ | ||
| + | {{:Logarithm and friends footer}} | ||
{{:*-integral functions footer}} | {{:*-integral functions footer}} | ||
| + | |||
[[Category:SpecialFunction]] | [[Category:SpecialFunction]] | ||
Revision as of 20:28, 25 June 2017
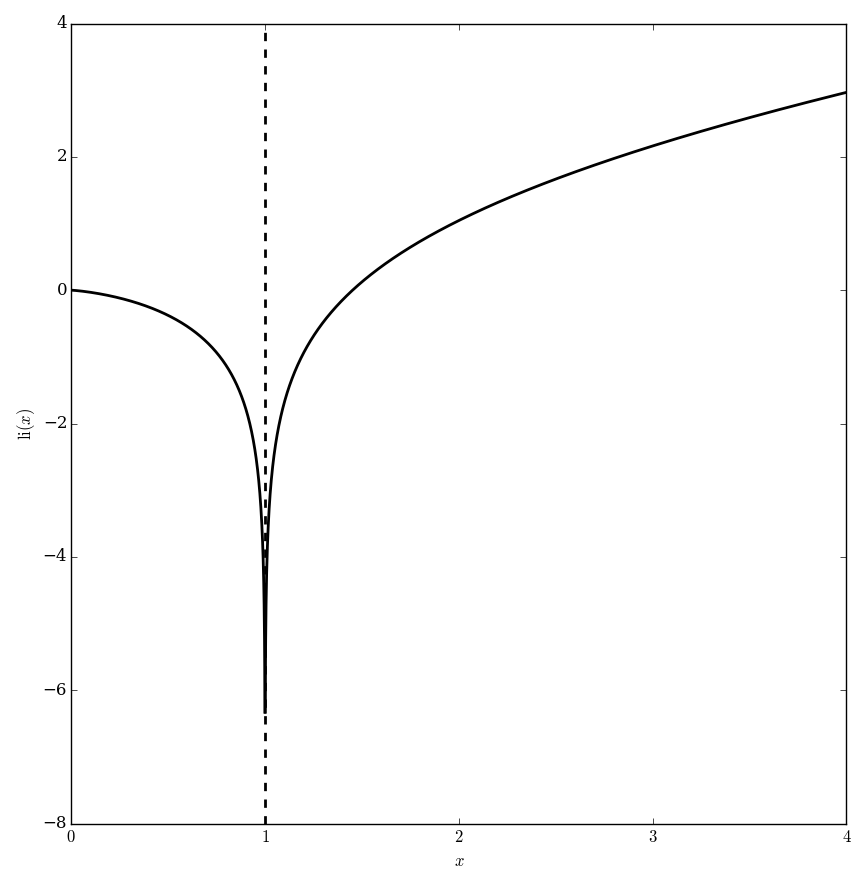
The logarithmic integral is $$\mathrm{li}(x) = \displaystyle\int_0^x \dfrac{1}{\log(t)} \mathrm{d}t,$$ where $\log$ denotes the logarithm.
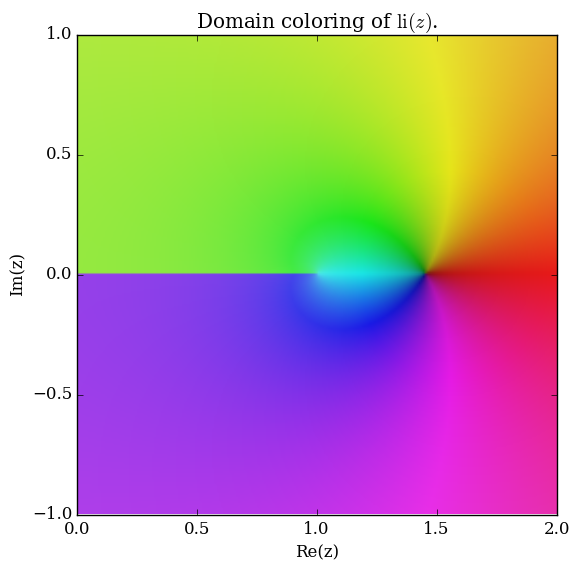
Domain coloring of $\mathrm{li}$.
Properties
Relationship between logarithmic integral and exponential integral
Prime number theorem, logarithmic integral
See Also
References
- 1964: Milton Abramowitz and Irene A. Stegun: Handbook of mathematical functions ... (previous) ... (next): $5.1.3$