Difference between revisions of "Exponential integral Ei"
From specialfunctionswiki
| (14 intermediate revisions by the same user not shown) | |||
| Line 1: | Line 1: | ||
| − | The exponential integral $\mathrm{Ei}$ is defined by | + | The exponential integral $\mathrm{Ei}$ is defined for $x>0$ by |
| − | $$\mathrm{Ei}( | + | $$\mathrm{Ei}(x) = \mathrm{PV}\int_{-\infty}^x \dfrac{e^t}{t} \mathrm{d}t,$$ |
| + | where $\mathrm{PV}$ denotes the [[Cauchy principal value]] and $e^t$ denotes the [[exponential]]. | ||
| Line 11: | Line 12: | ||
=Properties= | =Properties= | ||
| − | + | [[Ei(-x)=-Integral from -x to infinity of e^(-t)/t dt]]<br /> | |
| + | [[Relationship between logarithmic integral and exponential integral]]<br /> | ||
| + | [[Exponential integral Ei series]]<br /> | ||
| + | [[Relationship between exponential integral Ei, cosine integral, and sine integral]]<br /> | ||
| − | + | =See Also= | |
| − | + | [[Exponential integral E]] | |
| − | |||
=References= | =References= | ||
| − | + | * {{PaperReference|On certain definite integrals involving the exponential-integral|1881|James Whitbread Lee Glaisher|next=Ei(-x)=-Integral from x to infinity of e^(-t)/t dt}} | |
| + | * {{BookReference|Handbook of mathematical functions|1964|Milton Abramowitz|author2=Irene A. Stegun|prev=Ei(-x)=-Integral from -x to infinity of e^(-t)/t dt|next=Logarithmic integral}}: $5.1.2$ | ||
| + | {{:*-integral functions footer}} | ||
| − | + | [[Category:SpecialFunction]] | |
Latest revision as of 00:48, 24 March 2018
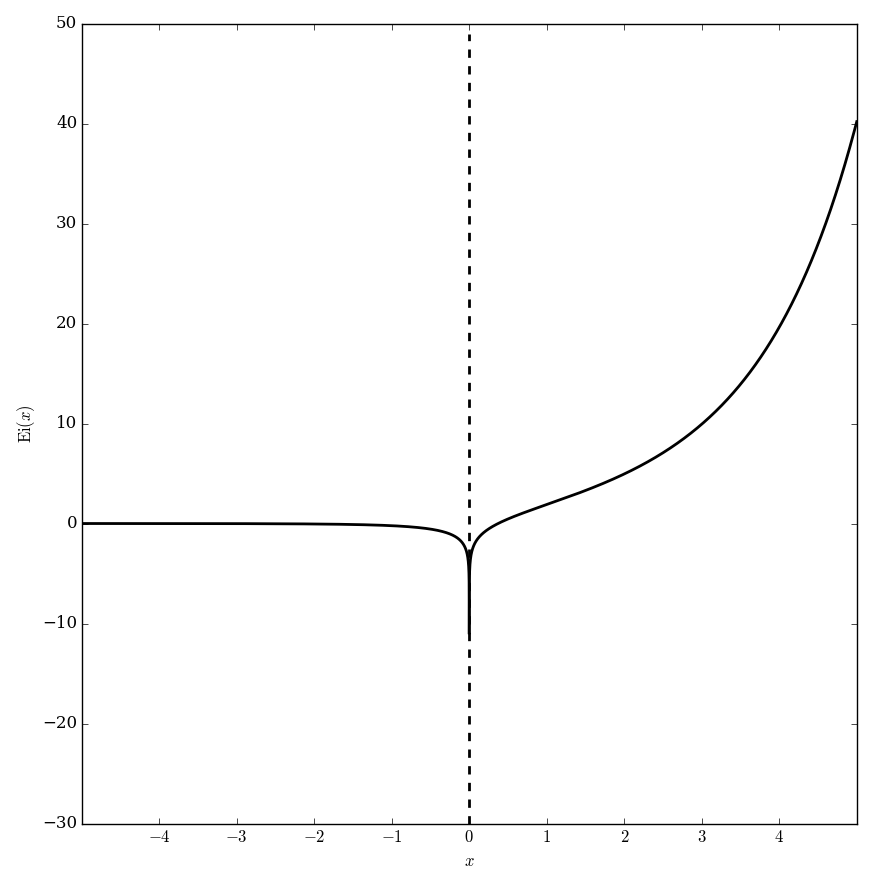
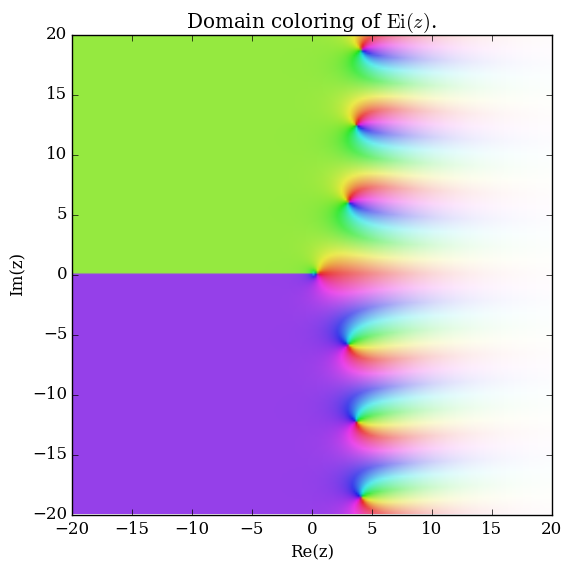
The exponential integral $\mathrm{Ei}$ is defined for $x>0$ by $$\mathrm{Ei}(x) = \mathrm{PV}\int_{-\infty}^x \dfrac{e^t}{t} \mathrm{d}t,$$ where $\mathrm{PV}$ denotes the Cauchy principal value and $e^t$ denotes the exponential.
Graph of $\mathrm{Ei}$.
Domain coloring of $\mathrm{Ei}$.
Properties
Ei(-x)=-Integral from -x to infinity of e^(-t)/t dt
Relationship between logarithmic integral and exponential integral
Exponential integral Ei series
Relationship between exponential integral Ei, cosine integral, and sine integral
See Also
References
- James Whitbread Lee Glaisher: On certain definite integrals involving the exponential-integral (1881)... (next)
- 1964: Milton Abramowitz and Irene A. Stegun: Handbook of mathematical functions ... (previous) ... (next): $5.1.2$
Exp integral $\mathrm{Ei}$
