Difference between revisions of "Spherical Bessel y"
From specialfunctionswiki
| (9 intermediate revisions by the same user not shown) | |||
| Line 1: | Line 1: | ||
| + | __NOTOC__ | ||
The spherical Bessel function of the second kind is | The spherical Bessel function of the second kind is | ||
$$y_{\nu}(z)=\sqrt{\dfrac{\pi}{2z}} Y_{\nu+\frac{1}{2}}(z),$$ | $$y_{\nu}(z)=\sqrt{\dfrac{\pi}{2z}} Y_{\nu+\frac{1}{2}}(z),$$ | ||
where $Y_{\nu}$ denotes the [[Bessel Y sub nu|Bessel function of the second kind]]. | where $Y_{\nu}$ denotes the [[Bessel Y sub nu|Bessel function of the second kind]]. | ||
| − | <center>{{:Bessel functions footer}} | + | <div align="center"> |
| + | <gallery> | ||
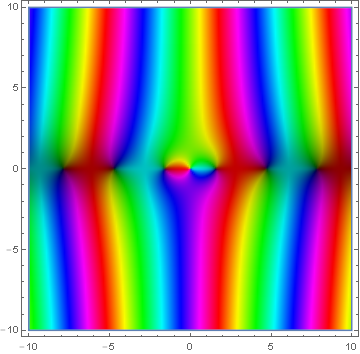
| + | File:Domcolsphericalbesselysub0.png|[[Domain coloring]] of $y_0$. | ||
| + | </gallery> | ||
| + | </div> | ||
| + | |||
| + | =Properties= | ||
| + | [[Relationship between spherical Bessel y and cosine]] | ||
| + | |||
| + | =References= | ||
| + | |||
| + | {{:Bessel functions footer}} | ||
| + | |||
| + | [[Category:SpecialFunction]] | ||
Latest revision as of 01:14, 18 July 2016
The spherical Bessel function of the second kind is $$y_{\nu}(z)=\sqrt{\dfrac{\pi}{2z}} Y_{\nu+\frac{1}{2}}(z),$$ where $Y_{\nu}$ denotes the Bessel function of the second kind.
Domain coloring of $y_0$.
Properties
Relationship between spherical Bessel y and cosine
References
Spherical Bessel $y_{\nu}$