Difference between revisions of "Jinc"
From specialfunctionswiki
| (7 intermediate revisions by the same user not shown) | |||
| Line 1: | Line 1: | ||
The $\mathrm{jinc}$ function is defined by | The $\mathrm{jinc}$ function is defined by | ||
$$\mathrm{jinc}(z)=\dfrac{J_1(z)}{z},$$ | $$\mathrm{jinc}(z)=\dfrac{J_1(z)}{z},$$ | ||
| − | where $J_1$ denotes the [[Bessel J | + | where $J_1$ denotes the [[Bessel J|Bessel function of the first kind]]. |
<div align="center"> | <div align="center"> | ||
<gallery> | <gallery> | ||
| − | File:Jincplot.png|Graph of $\mathrm{ | + | File:Jincplot.png|Graph of $\mathrm{jinc}$ on $[-1,1]$. |
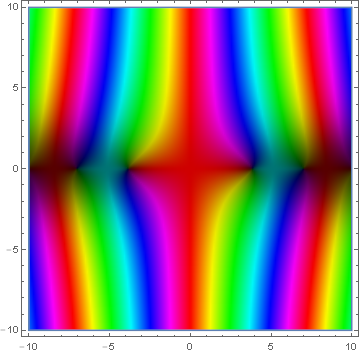
File:Domcoljinc.png|[[Domain coloring]] of $\mathrm{jinc}$. | File:Domcoljinc.png|[[Domain coloring]] of $\mathrm{jinc}$. | ||
</gallery> | </gallery> | ||
| Line 11: | Line 11: | ||
=References= | =References= | ||
[http://www.johndcook.com/blog/2012/02/01/jinc-function/]<br /> | [http://www.johndcook.com/blog/2012/02/01/jinc-function/]<br /> | ||
| + | |||
| + | =See Also= | ||
| + | [[Bessel J]] | ||
| + | |||
| + | {{:*-c functions footer}} | ||
| + | |||
| + | [[Category:SpecialFunction]] | ||
Latest revision as of 04:03, 22 September 2016
The $\mathrm{jinc}$ function is defined by $$\mathrm{jinc}(z)=\dfrac{J_1(z)}{z},$$ where $J_1$ denotes the Bessel function of the first kind.
Domain coloring of $\mathrm{jinc}$.