Difference between revisions of "Versine"
From specialfunctionswiki
| (7 intermediate revisions by the same user not shown) | |||
| Line 2: | Line 2: | ||
$$\mathrm{versin}(z)=1-\cos(z),$$ | $$\mathrm{versin}(z)=1-\cos(z),$$ | ||
where $ \cos$ denotes the [[cosine]] function. | where $ \cos$ denotes the [[cosine]] function. | ||
| + | |||
| + | <div align="center"> | ||
| + | <gallery> | ||
| + | File:Versinplot.png|Plot of $\mathrm{versin}$ on $[-10,10]$. | ||
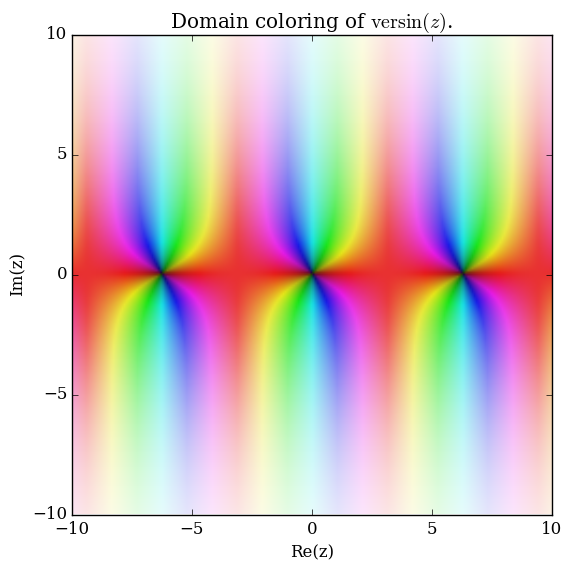
| + | File:Complexversinplot.png|[[Domain coloring]] of $\mathrm{versin}$. | ||
| + | File:Trig Functions Diagram.svg|Trig functions diagram using the unit circle. | ||
| + | </gallery> | ||
| + | </div> | ||
=Properties= | =Properties= | ||
| + | [[Derivative of versine]]<br /> | ||
| + | [[Antiderivative of versine]]<br /> | ||
=References= | =References= | ||
| − | * {{BookReference|Handbook of mathematical functions|1964|Milton Abramowitz|author2=Irene A. Stegun|prev= | + | * {{BookReference|Handbook of mathematical functions|1964|Milton Abramowitz|author2=Irene A. Stegun|prev=findme|next=Coversine}}: $4.3.147$ |
| + | |||
| + | [[Category:SpecialFunction]] | ||
| + | [[Category:Definition]] | ||
Latest revision as of 02:33, 5 January 2017
The versine function $\mathrm{versin} \colon \mathbb{C} \rightarrow \mathbb{C}$ is defined by the formula $$\mathrm{versin}(z)=1-\cos(z),$$ where $ \cos$ denotes the cosine function.
Plot of $\mathrm{versin}$ on $[-10,10]$.
Domain coloring of $\mathrm{versin}$.
Trig functions diagram using the unit circle.
Properties
Derivative of versine
Antiderivative of versine
References
- 1964: Milton Abramowitz and Irene A. Stegun: Handbook of mathematical functions ... (previous) ... (next): $4.3.147$
