Difference between revisions of "Dedekind eta"
From specialfunctionswiki
| Line 2: | Line 2: | ||
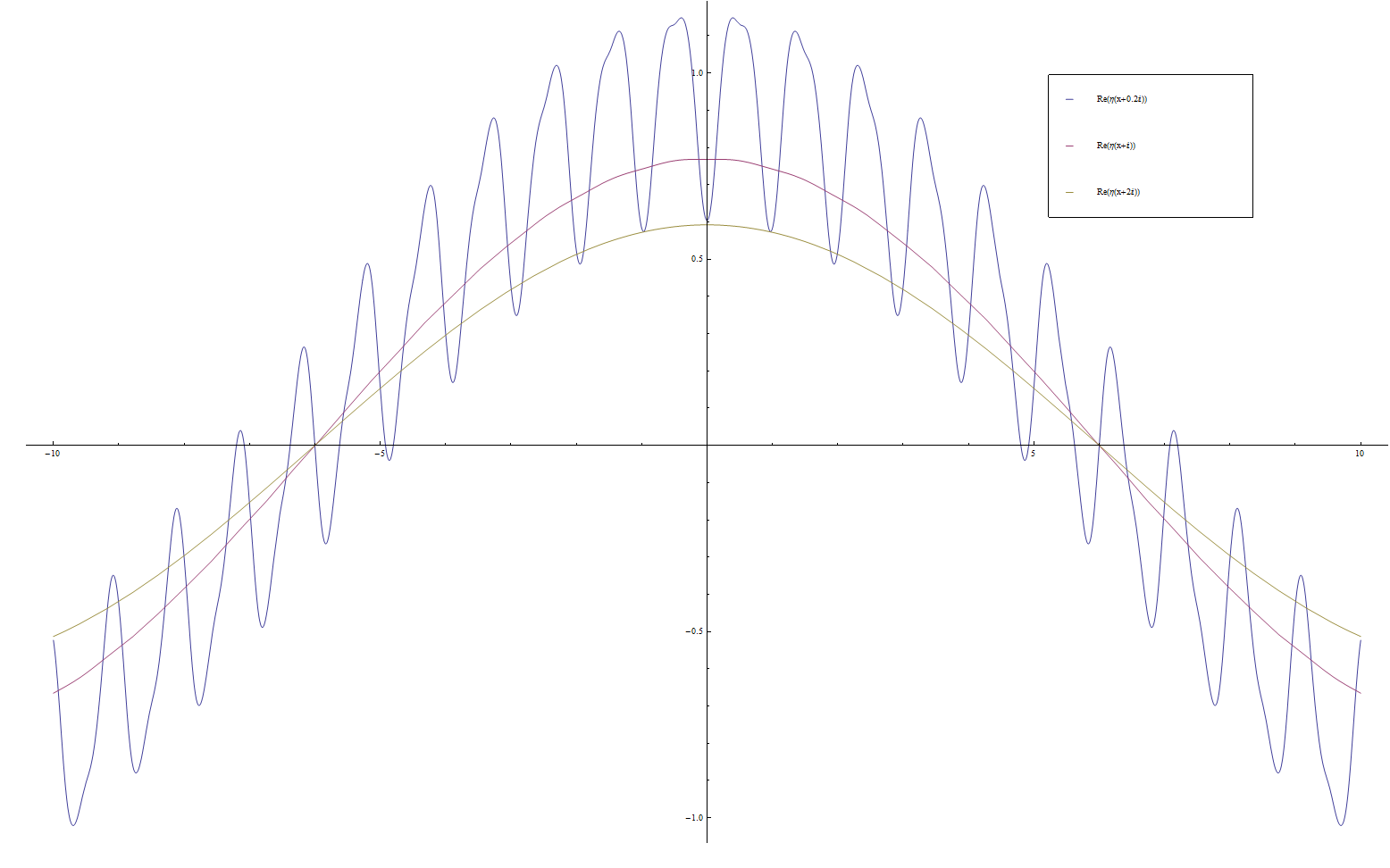
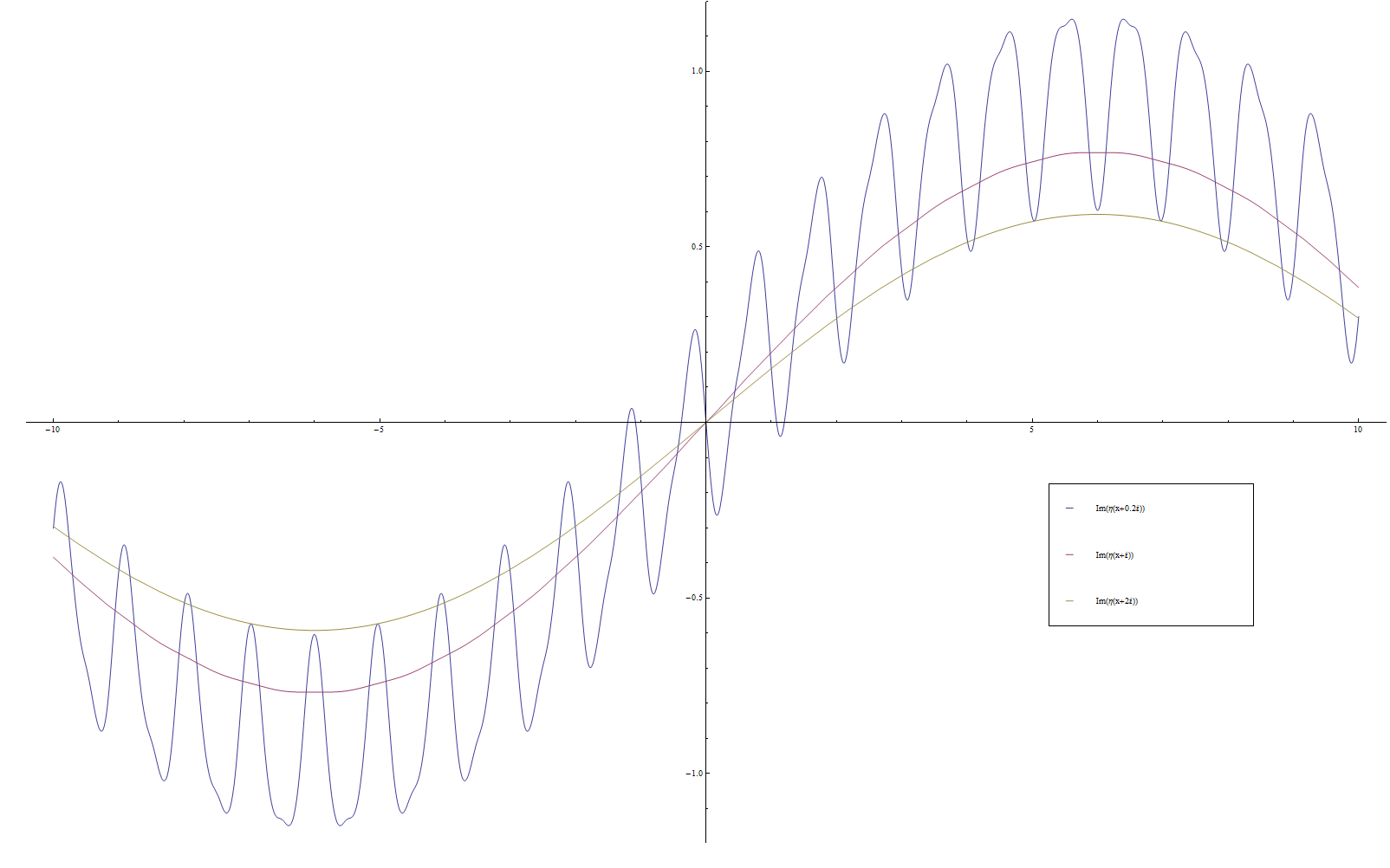
$$\eta(\tau) = e^{\frac{\pi i \tau}{12}} \displaystyle\prod_{n=1}^{\infty} (1-q^n).$$ | $$\eta(\tau) = e^{\frac{\pi i \tau}{12}} \displaystyle\prod_{n=1}^{\infty} (1-q^n).$$ | ||
| − | + | <div align="center"> | |
| − | + | <gallery> | |
| − | + | File:DedekindetaRe.png|Real part of $\eta$. | |
| + | File:DedekindetaIm.png|Imaginary part of $\eta$. | ||
| + | </gallery> | ||
| + | </div> | ||
=References= | =References= | ||
[http://eta.math.georgetown.edu/ A collection of over 6200 identities for the Dedekind Eta Function] | [http://eta.math.georgetown.edu/ A collection of over 6200 identities for the Dedekind Eta Function] | ||
Revision as of 03:23, 19 January 2015
Let $q=e^{2\pi i \tau}$. We define the Dedekind eta function by the formula $$\eta(\tau) = e^{\frac{\pi i \tau}{12}} \displaystyle\prod_{n=1}^{\infty} (1-q^n).$$
Real part of $\eta$.
Imaginary part of $\eta$.
References
A collection of over 6200 identities for the Dedekind Eta Function
