Difference between revisions of "Beta"
From specialfunctionswiki
m (Tom moved page Beta function to Beta) |
(→References) |
||
| Line 36: | Line 36: | ||
=References= | =References= | ||
Bell. Special Functions | Bell. Special Functions | ||
| + | [http://web.mst.edu/~lmhall/SPFNS/spfns.pdf Special functions by Leon Hall] | ||
Revision as of 06:56, 11 February 2015
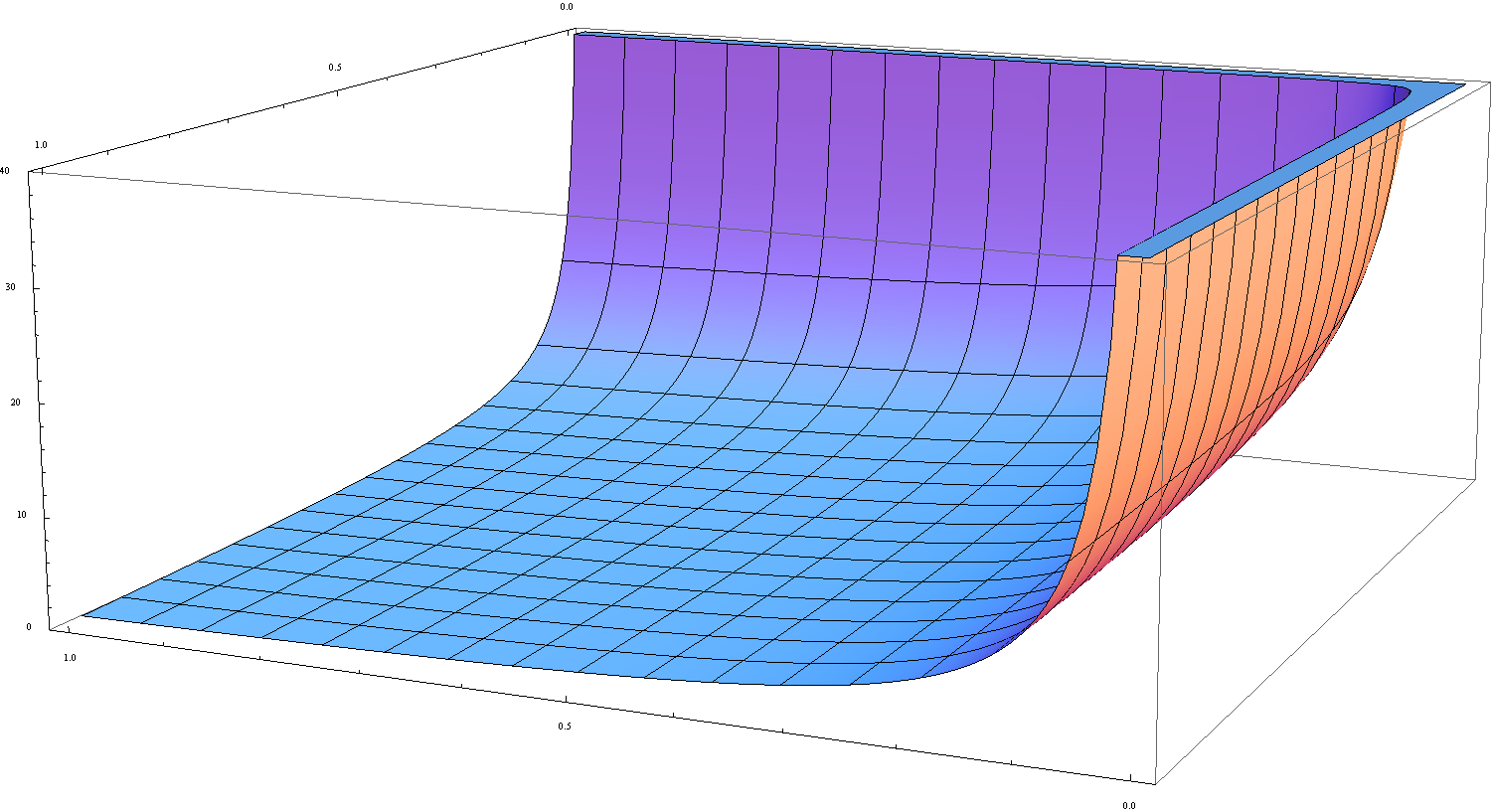
The $\beta$ function is defined by the formula $$B(x,y)=\displaystyle\int_0^1 t^{x-1}(1-t)^{y-1}dt.$$
Properties
Theorem: The following formula holds: $$B(x,y)=\dfrac{\Gamma(x)\Gamma(y)}{\Gamma(x+y)},$$ where $\Gamma$ denotes the gamma function.
Proof: █
Theorem: $B(x,y)=B(y,x)$
Proof: █
Theorem: (i) $B(x+1,y)=\dfrac{x}{x+y} B(x,y)$
(ii) $B(x,y+1)=\dfrac{y}{x+y}B(x,y)$
Proof: █
References
Bell. Special Functions Special functions by Leon Hall