Difference between revisions of "Legendre P"
From specialfunctionswiki
| Line 9: | Line 9: | ||
\end{array}$$ | \end{array}$$ | ||
| − | + | <div align="center"> | |
| + | <gallery> | ||
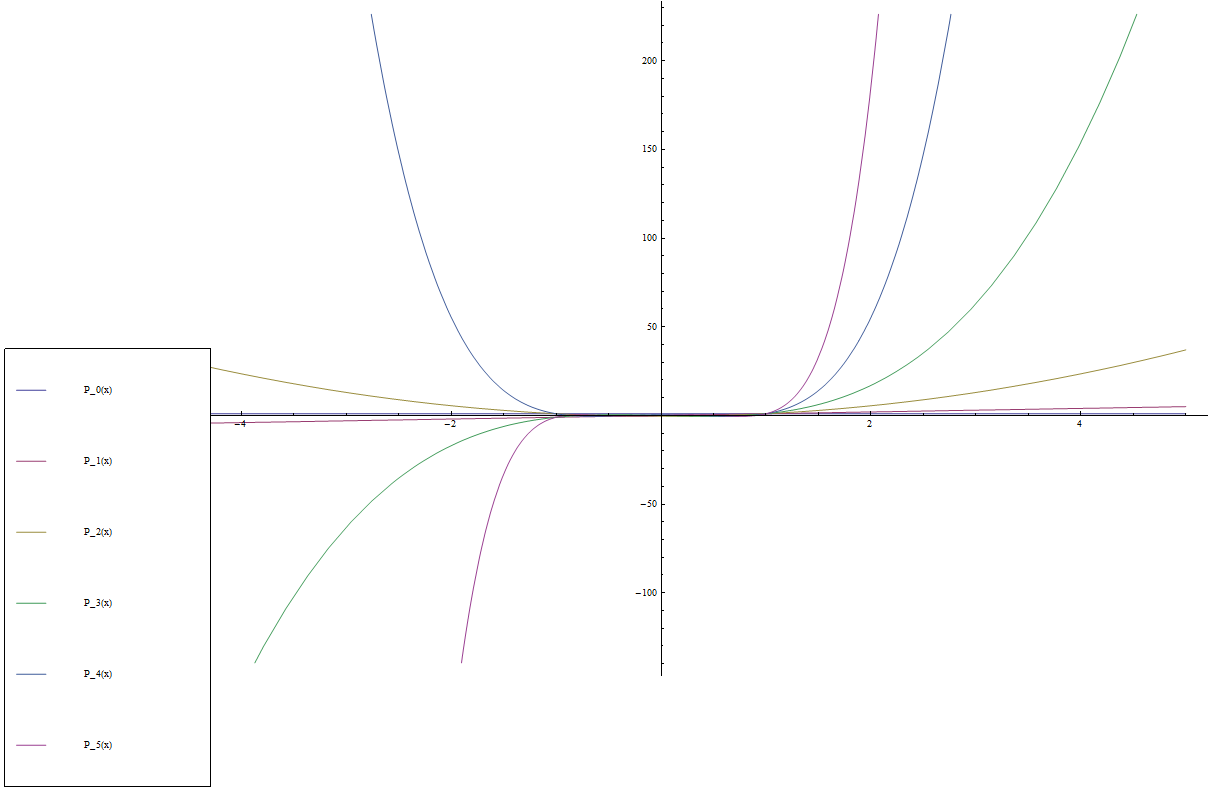
| + | File:Legendrepolynomials.png|Graph of $P_n$ on $[-4,4]$ for $n=0,1,2,3,4,5$. | ||
| + | </gallery> | ||
| + | </div> | ||
{{:Orthogonal polynomials footer}} | {{:Orthogonal polynomials footer}} | ||
Revision as of 20:18, 23 March 2015
The Legendre polynomials are orthogonal polynomials defined by the recurrence $$P_n(x) = \dfrac{1}{2^nn!}\dfrac{d^n}{dx^n}(x^2-1)^n; n=0,1,2,\ldots$$ $$\begin{array}{ll} P_0(x) &= 1 \\ P_1(x) &= x \\ P_2(x) &= \dfrac{1}{2}(3x^2-1) \\ P_3(x) &= \dfrac{1}{2}(5x^3-3x) \\ \vdots \end{array}$$
Graph of $P_n$ on $[-4,4]$ for $n=0,1,2,3,4,5$.
