Difference between revisions of "Modified Bessel I"
| Line 34: | Line 34: | ||
</div> | </div> | ||
</div> | </div> | ||
| + | |||
| + | {{:Relationship between Bessel I sub n and Bessel J sub n}} | ||
{{:Relationship between Airy Bi and modified Bessel I}} | {{:Relationship between Airy Bi and modified Bessel I}} | ||
<center>{{:Bessel functions footer}}</center> | <center>{{:Bessel functions footer}}</center> | ||
Revision as of 08:53, 16 June 2015
The modified Bessel function of the first kind is defined by $$I_{\nu}(z)=i^{-\nu}J_{\nu}(iz),$$ where $J_{\nu}$ is the Bessel function of the first kind.
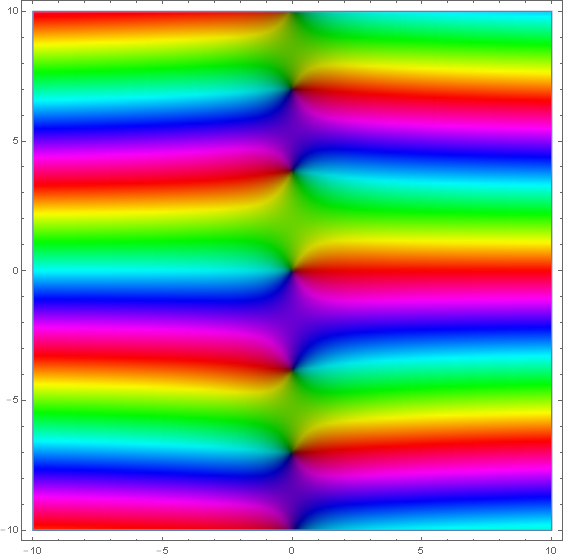
Domain coloring of analytic continuation of $I_1(z)$.
Contents
Properties
Proposition: The following formula holds: $$I_{-\frac{1}{2}}(z)=\sqrt{\dfrac{2}{\pi z}} \cosh(z).$$
Proof: █
Proposition: The following formula holds: $$I_{\frac{1}{2}}(z)=\sqrt{\dfrac{2}{\pi z}}\sinh(z).$$
Proof: █
Proposition: The following formula holds: $$I_{\nu}(z)=\displaystyle\sum_{k=0}^{\infty} J_{\nu+k}(z) \dfrac{z^k}{k!},$$ where $J_{\nu}$ denotes the Bessel function of the first kind.
Proof: █
Theorem
The following formula holds: $$\mathrm{Bi}(z)=\sqrt{\dfrac{z}{3}} \left( I_{\frac{1}{3}}\left(\frac{2}{3}x^{\frac{3}{2}} \right) + I_{-\frac{1}{3}} \left( \frac{2}{3} x^{\frac{3}{2}} \right) \right),$$ where $\mathrm{Bi}$ denotes the Airy Bi function and $I_{\nu}$ denotes the modified Bessel $I$.