Difference between revisions of "Jacobi ds"
From specialfunctionswiki
| Line 13: | Line 13: | ||
<center>{{:Jacobi elliptic functions footer}}</center> | <center>{{:Jacobi elliptic functions footer}}</center> | ||
| + | |||
| + | [[Category:SpecialFunction]] | ||
Revision as of 18:38, 24 May 2016
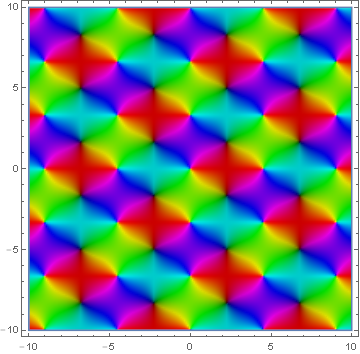
The $\mathrm{ds}$ function is defined by $$\mathrm{ds}(u)=\dfrac{\mathrm{dn}(u)}{\mathrm{sn}(u)},$$ where $\mathrm{dn}$ is the Jacobi dn function and $\mathrm{sn}$ is the Jacobi sn function.
Domain coloring of $\mathrm{ds}$ corresponding to $m=0.8$.
References
Special functions by Leon Hall