Difference between revisions of "Dirichlet beta"
From specialfunctionswiki
| Line 1: | Line 1: | ||
| + | __NOTOC__ | ||
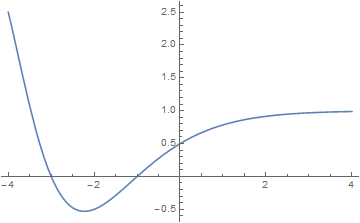
The Dirichlet $\beta$ function is defined by | The Dirichlet $\beta$ function is defined by | ||
$$\beta(x) = \displaystyle\sum_{k=0}^{\infty} (-1)^k (2k+1)^{-x} = 2^{-x} \Phi \left(-1,x,\dfrac{1}{2} \right),$$ | $$\beta(x) = \displaystyle\sum_{k=0}^{\infty} (-1)^k (2k+1)^{-x} = 2^{-x} \Phi \left(-1,x,\dfrac{1}{2} \right),$$ | ||
| Line 12: | Line 13: | ||
=Properties= | =Properties= | ||
| − | + | [[Catalan's constant using Dirichlet beta]]<br /> | |
[[Category:SpecialFunction]] | [[Category:SpecialFunction]] | ||
Revision as of 17:42, 24 June 2016
The Dirichlet $\beta$ function is defined by $$\beta(x) = \displaystyle\sum_{k=0}^{\infty} (-1)^k (2k+1)^{-x} = 2^{-x} \Phi \left(-1,x,\dfrac{1}{2} \right),$$ where $\Phi$ denotes the Lerch transcendent.
Domain coloring of analytic continuation of $\beta$.