Difference between revisions of "Bessel J"
| Line 14: | Line 14: | ||
=Properties= | =Properties= | ||
| + | [[Bessel J sub nu and Y sub nu solve Bessel's differential equation]]<br /> | ||
| + | [[Bessel J sub nu and Y sub nu solve Bessel's differential equation (constant multiple in argument)]]<br /> | ||
| + | [[Bessel J sub nu and Y sub nu solve Bessel's differential equation (monomial multiple outside,weighted monomial in argument)]]<br /> | ||
| + | [[Bessel polynomial in terms of Bessel functions]]<br /> | ||
| + | [[Bessel at n+1/2 in terms of Bessel polynomial]]<br /> | ||
| + | [[Bessel at -n-1/2 in terms of Bessel polynomial]]<br /> | ||
| + | [[Relationship between Bessel J sub nu and hypergeometric 0F1]]<br /> | ||
| + | [[Relationship between Bessel I sub n and Bessel J sub n]]<br /> | ||
| + | [[Relationship between Anger function and Bessel J sub nu]]<br /> | ||
<div class="toccolours mw-collapsible mw-collapsed"> | <div class="toccolours mw-collapsible mw-collapsed"> | ||
<strong>Theorem:</strong> If $n \in \mathbb{Z}$, then $J_{-n}(x)=(-1)^nJ_n(x)$. Moreover this means that $J_n$ and $J_{-n}$ are [[linearly dependent]]. | <strong>Theorem:</strong> If $n \in \mathbb{Z}$, then $J_{-n}(x)=(-1)^nJ_n(x)$. Moreover this means that $J_n$ and $J_{-n}$ are [[linearly dependent]]. | ||
| Line 21: | Line 30: | ||
</div> | </div> | ||
| − | |||
| − | |||
| − | |||
| − | |||
<div class="toccolours mw-collapsible mw-collapsed" style="width:800px"> | <div class="toccolours mw-collapsible mw-collapsed" style="width:800px"> | ||
| Line 68: | Line 73: | ||
=Relations to other special functions= | =Relations to other special functions= | ||
| − | + | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
=Videos= | =Videos= | ||
Revision as of 19:08, 6 June 2016
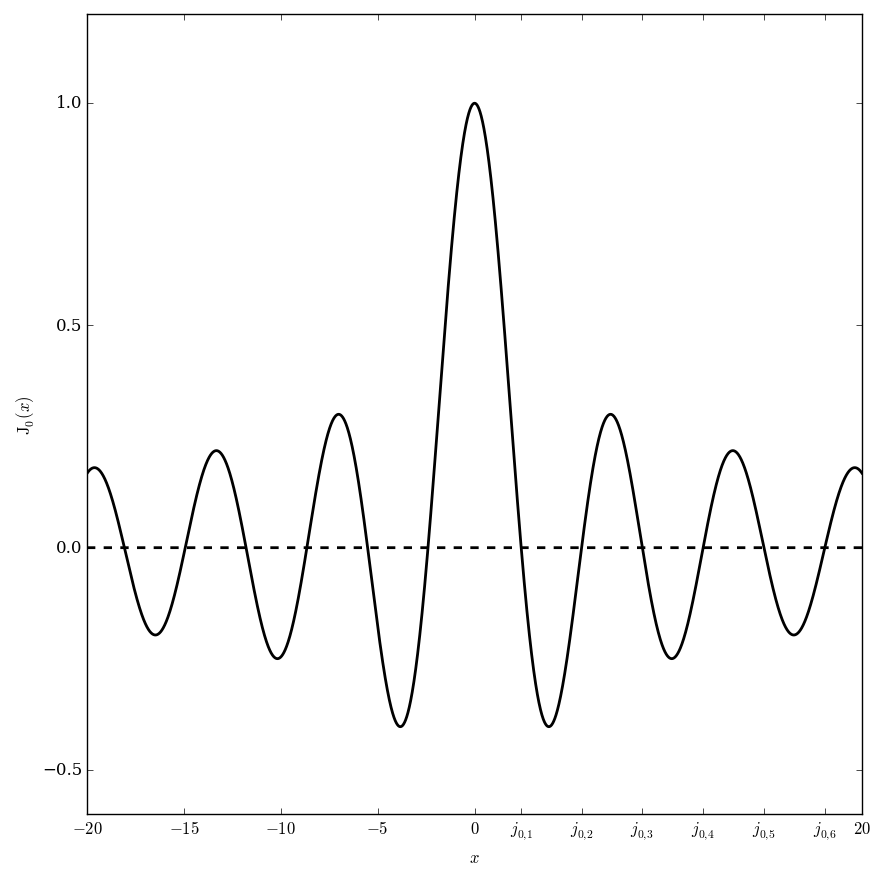
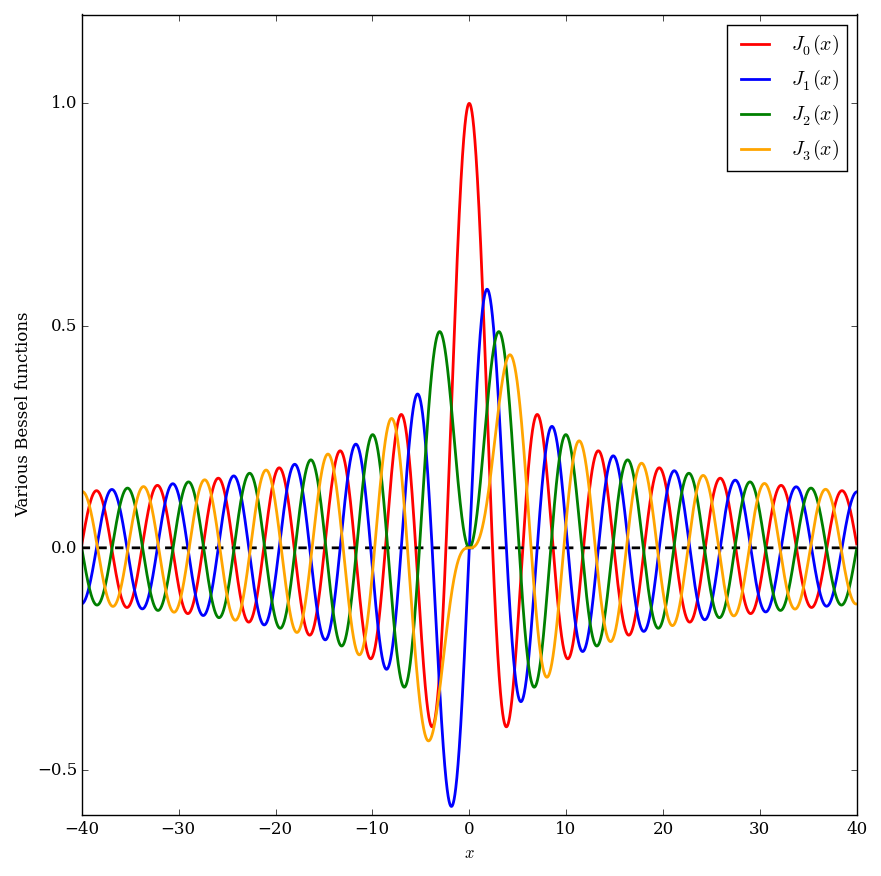
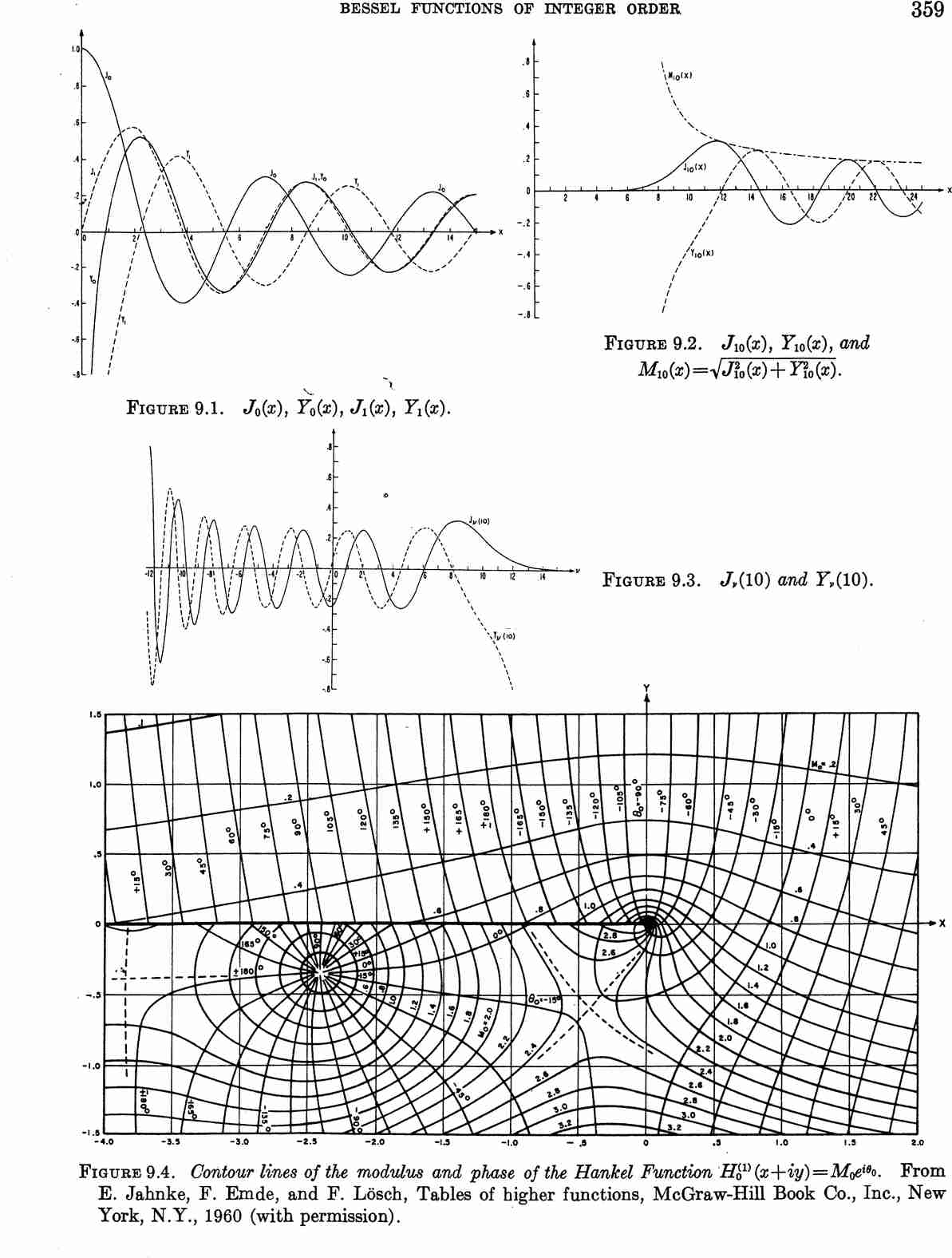
The Bessel functions of the first kind, $J_{\nu}$, have a power series expansion $$J_{\nu}(z)=\displaystyle\sum_{k=0}^{\infty} \dfrac{(-1)^k}{k! \Gamma(k+\nu+1)2^{2k+\nu}}z^{2k+\nu}.$$
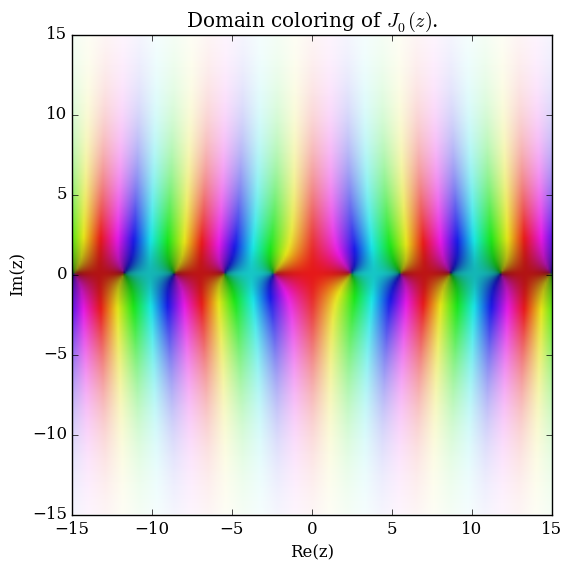
Domain coloring of $J_0$.
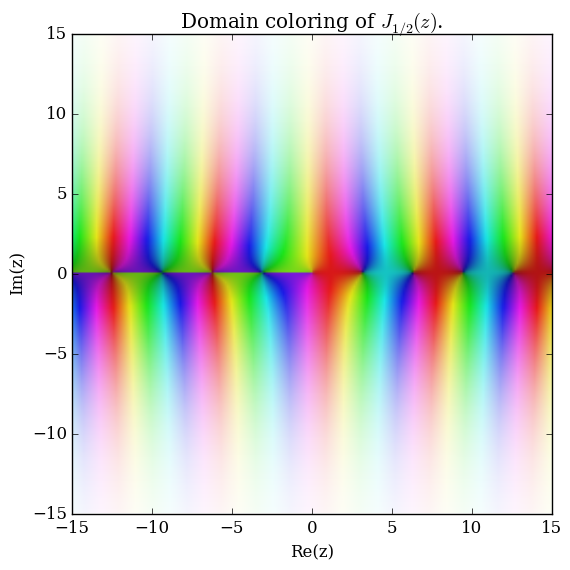
Domain coloring of $J_{\frac{1}{2}}$.
Domain coloring of $J_5$.
Bessel functions from Abramowitz&Stegun
Properties
Bessel J sub nu and Y sub nu solve Bessel's differential equation
Bessel J sub nu and Y sub nu solve Bessel's differential equation (constant multiple in argument)
Bessel J sub nu and Y sub nu solve Bessel's differential equation (monomial multiple outside,weighted monomial in argument)
Bessel polynomial in terms of Bessel functions
Bessel at n+1/2 in terms of Bessel polynomial
Bessel at -n-1/2 in terms of Bessel polynomial
Relationship between Bessel J sub nu and hypergeometric 0F1
Relationship between Bessel I sub n and Bessel J sub n
Relationship between Anger function and Bessel J sub nu
Theorem: If $n \in \mathbb{Z}$, then $J_{-n}(x)=(-1)^nJ_n(x)$. Moreover this means that $J_n$ and $J_{-n}$ are linearly dependent.
Proof: █
Theorem: The following formula holds: $$zJ_{\nu}'(z)=\nu J_{\nu}(z) - z J_{\nu+1}(z).$$
Proof: █
Theorem: The following formula holds: $$\dfrac{d}{dz}[z^{-\nu}J_{\nu}(z)] = -z^{-\nu}J_{\nu+1}(z).$$
Proof: █
Theorem: (Generating function) The following formula holds: $$\exp \left( \dfrac{1}{2} z \left( t-\dfrac{1}{t} \right) \right) = \displaystyle\sum_{k=-\infty}^{\infty} t^k J_k(z).$$
Proof: █
Theorem: The following formula holds for $n\in\mathbb{Z}$: $$J_n(z)=\dfrac{1}{\pi} \displaystyle\int_0^{\pi} \cos(n\xi-x\sin(\xi))d\xi.$$
Proof: █
Theorem: The following formula holds for $n>-\frac{1}{2}$: $$J_n(z)=\dfrac{\left(\frac{z}{2}\right)^n}{\sqrt{\pi}\Gamma(n+\frac{1}{2})} \displaystyle\int_{-1}^1 (1-t^2)^{n-\frac{1}{2}}e^{izt}dt.$$
Proof: █
Relations to other special functions
Videos
Bessel Equation and Bessel functions
Mod-1 Lec-6 Bessel Functions and Their Properties-I
Bessel's Equation by Free Academy
Taylor Series, Bessel, single Variable Calculus, Coursera.org
Ordinary Differential Equations Lecture 7—Bessel functions and the unit step function
Laplace transform of Bessel function order zero
Laplace transform: Integral over Bessel function is one
Orthogonal Properties of Bessel Function, Orthogonal Properties of Bessel Equation
Links
Addition formulas for Bessel functions
Relations between Bessel functions by John D. Cook