Difference between revisions of "Exponential integral Ei"
| Line 18: | Line 18: | ||
=References= | =References= | ||
| − | [http://dualaud.net/specialfunctionswiki/abramowitz_and_stegun-1.03/page_228.htm Exponential Integral and Related Functions] | + | [http://dualaud.net/specialfunctionswiki/abramowitz_and_stegun-1.03/page_228.htm Exponential Integral and Related Functions]<br /> |
| + | [http://gdz.sub.uni-goettingen.de/dms/load/img/?PID=PPN600494829_0018%7CLOG_0048 On certain definite integrals involving the exponential-integral - J.W.L. Glaisher] | ||
<center>{{:*-integral functions footer}}</center> | <center>{{:*-integral functions footer}}</center> | ||
[[Category:SpecialFunction]] | [[Category:SpecialFunction]] | ||
Revision as of 09:26, 1 June 2016
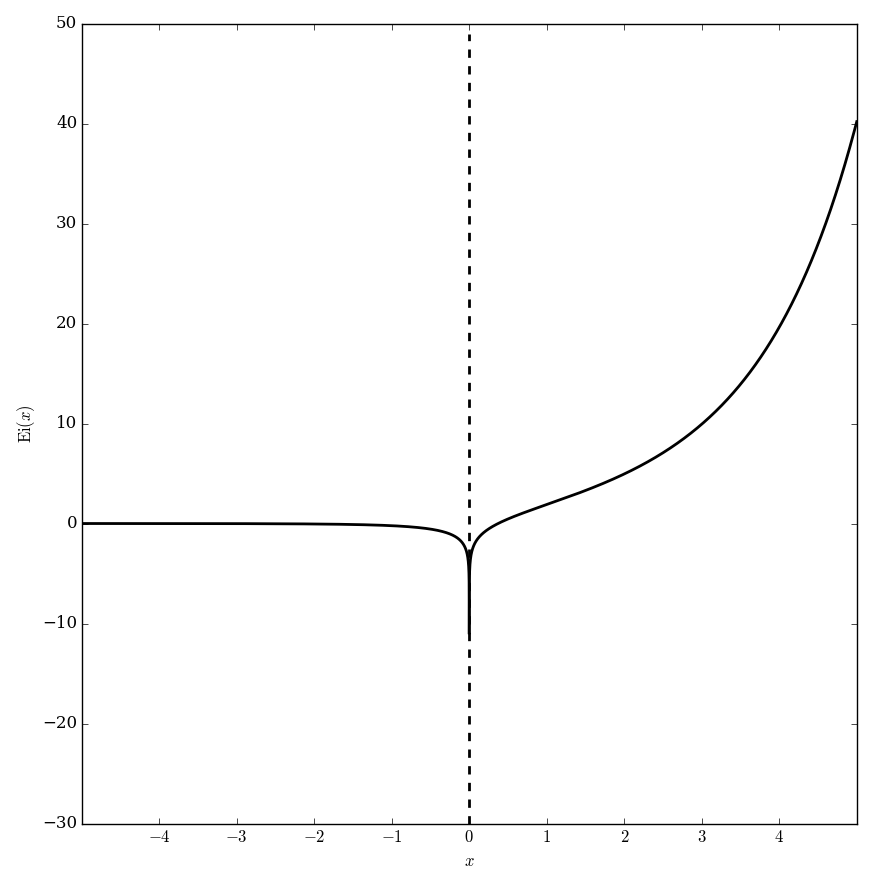
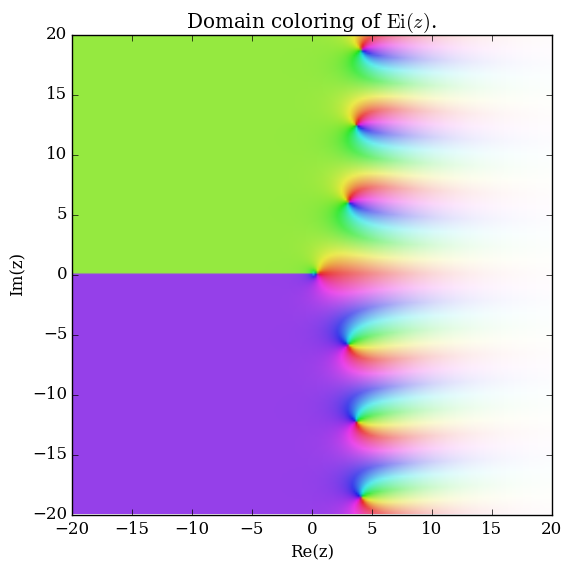
The exponential integral $\mathrm{Ei}$ is defined by $$\mathrm{Ei}(z) = \int_{-\infty}^x \dfrac{e^t}{t} \mathrm{d}t, \quad \left|\mathrm{arg}(-z) \right|<\pi.$$
Contents
Properties
Theorem
The following formula holds: $$\mathrm{li}(x)=\mathrm{Ei}( \log(x)),$$ where $\mathrm{li}$ denotes the logarithmic integral, $\mathrm{Ei}$ denotes the exponential integral Ei, and $\log$ denotes the logarithm.
Proof
References
- James Whitbread Lee Glaisher: On certain definite integrals involving the exponential-integral (1881)... (previous)... (next) (note: expresses this relationship as $\mathrm{Ei}(x)=\mathrm{li}(e^x)$)
Theorem
The following formula holds for $x>0$: $$\mathrm{Ei}(x) = \gamma + \log x + \displaystyle\sum_{k=1}^{\infty} \dfrac{x^k}{kk!},$$ where $\mathrm{Ei}$ denotes the exponential integral Ei, $\log$ denotes the logarithm, and $\gamma$ denotes the Euler-Mascheroni constant.
Proof
References
- James Whitbread Lee Glaisher: On certain definite integrals involving the exponential-integral (1881)... (previous)... (next) (note: expresses the logarithm term as $\frac{1}{4}\log(x^4)$)
Theorem
The following formula holds: $$\mathrm{Ei}(ix)=\mathrm{Ci}(x)+i\mathrm{Si}(x),$$ where $\mathrm{Ei}$ denotes the exponential integral Ei, $\mathrm{Ci}$ denotes the cosine integral, and $\mathrm{Si}$ denotes the sine integral.
Proof
References
References
Exponential Integral and Related Functions
On certain definite integrals involving the exponential-integral - J.W.L. Glaisher