Revision as of 09:26, 4 June 2016

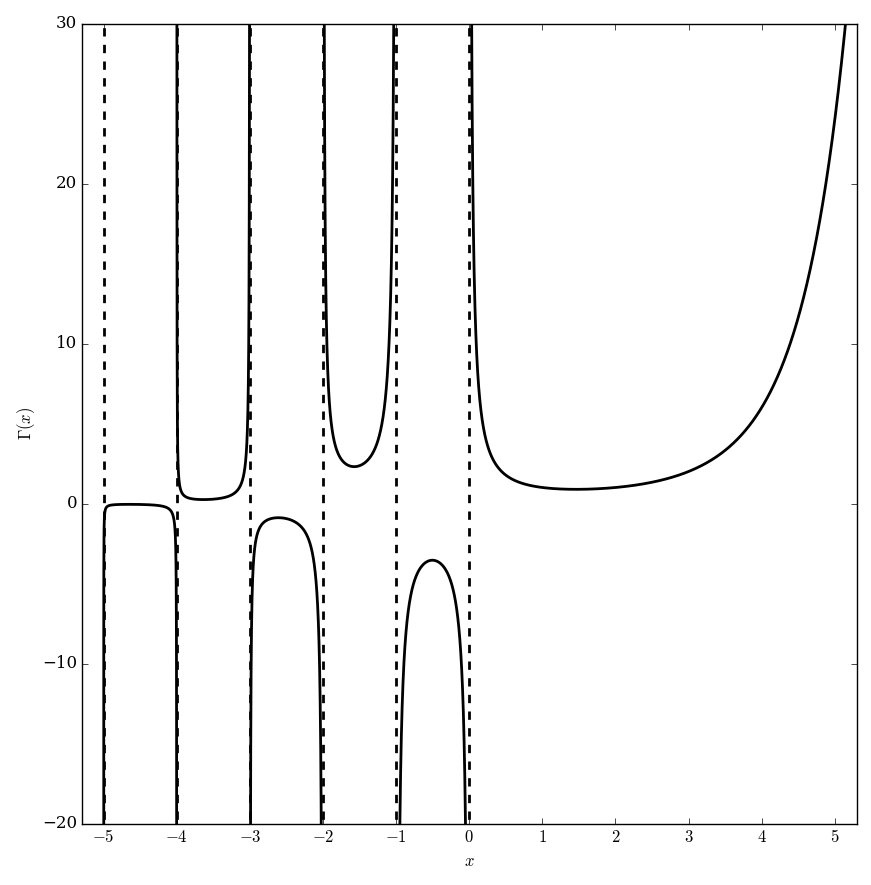
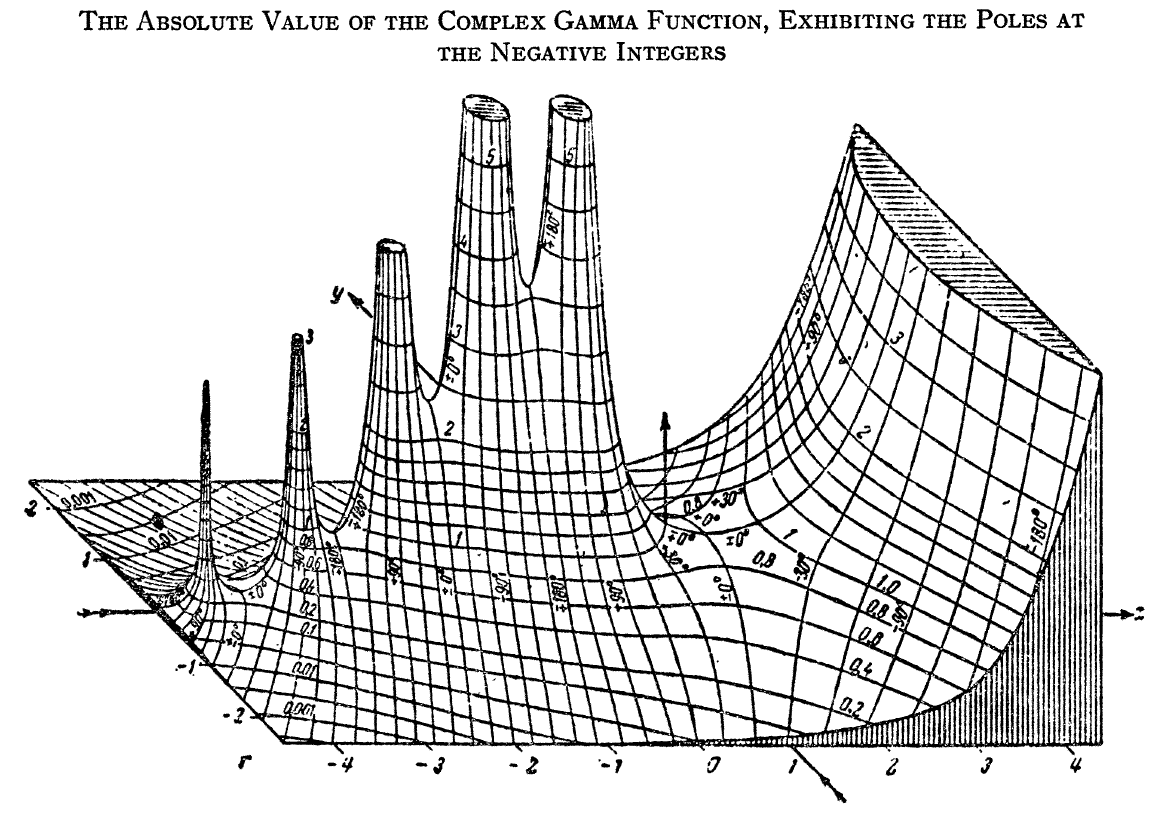
The gamma function $\Gamma \colon \mathbb{C} \setminus \{0,-1,-2,\ldots\} \rightarrow \mathbb{C}$ is the function initially defined for $\mathrm{Re}(z)>0$ by the integral by the formula $$\Gamma(x)=\displaystyle\int_0^{\infty} \xi^{x-1}e^{-\xi} \mathrm{d}\xi.$$ The analytic continuation of $\Gamma$ leads to a meromorphic function with poles at the negative integers.

Properties

Gamma(z) as integral of a power of log(1/t) for Re(z) greater than 0
Value of Gamma(1)
Factorial property of gamma
Gamma at positive integers
Gamma function Weierstrass product
Relationship between Hurwitz zeta and gamma function
Gamma-Sine Relation
Bohr-Mollerup theorem

Videos

Gamma Function (playlist)
The Gamma Function: intro (5)
Gamma Integral Function - Introduction
Gamma function
Mod-04 Lec-09 Analytic continuation and the gamma function (Part I)
gamma function - Part 1
Beta Function, Gamma Function and their Properties
What's the Gamma Function?
euler gamma function
Thermodynamics 19 a : Gamma Function 1/2
The Gamma Function: why 0!=1 (5)
Gamma Function Of One-Half: Part 1
Gamma Function Of One-Half: Part 2
Gamma function at 1/2
Contour Integral Definition of the Gamma Function

See Also

Loggamma
Polygamma
Reciprocal gamma

References

• 1953: Harry Bateman: Higher Transcendental Functions Volume I ... (next): §1.1 (1)

The Gamma Function by Emil Artin
The sine product formula and the gamma function
Leonhard Euler's Integral: A Historical Profile of the Gamma Function