Difference between revisions of "Tanh"
From specialfunctionswiki
| Line 12: | Line 12: | ||
=Properties= | =Properties= | ||
| − | + | [[Derivative of tanh]]<br /> | |
| − | + | [[Antiderivative of tanh]]<br /> | |
| − | + | [[Relationship between tanh and tan]]<br /> | |
| − | + | [[Relationship between tan and tanh]]<br /> | |
| − | + | [[Relationship between sine, Gudermannian, and tanh]]<br /> | |
| − | + | [[Relationship between tanh, inverse Gudermannian, and sin]]<br /> | |
| − | + | [[Taylor series for Gudermannian]]<br /> | |
=See Also= | =See Also= | ||
Revision as of 07:51, 8 June 2016
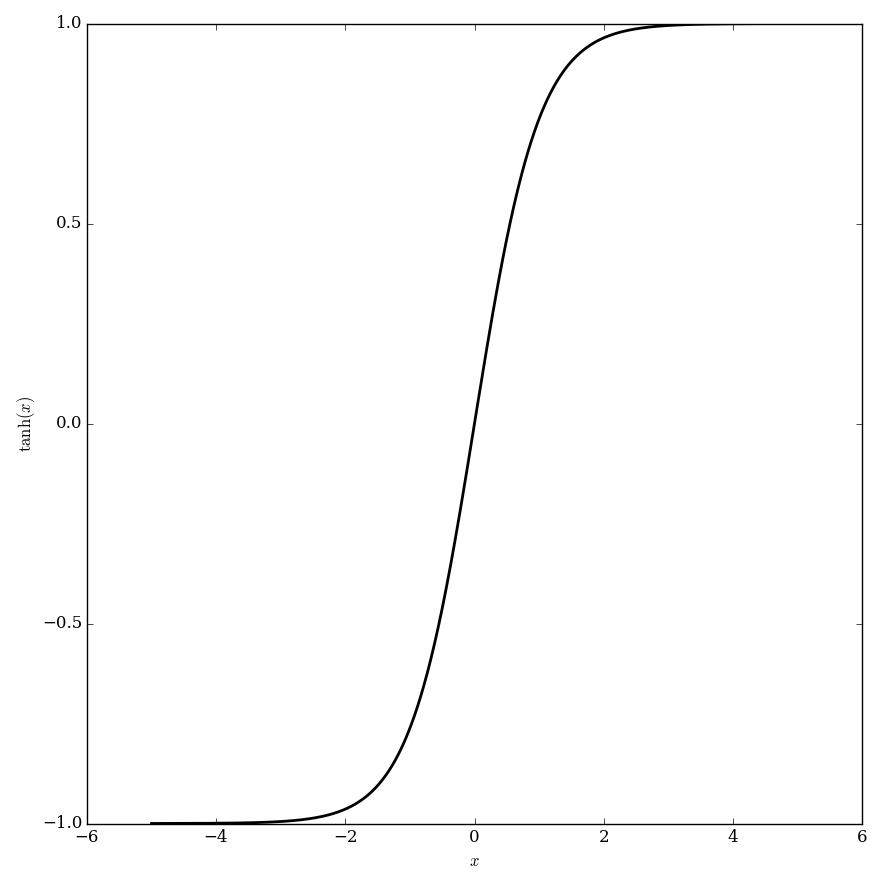
The hyperbolic tangent is defined by the formula $$\mathrm{tanh}(z)=\dfrac{\mathrm{sinh}(z)}{\mathrm{cosh}(z)},$$ where $\mathrm{sinh}$ is the hyperbolic sine and $\mathrm{cosh}$ is the hyperbolic cosine.
Domain coloring of $\tanh$.
Properties
Derivative of tanh
Antiderivative of tanh
Relationship between tanh and tan
Relationship between tan and tanh
Relationship between sine, Gudermannian, and tanh
Relationship between tanh, inverse Gudermannian, and sin
Taylor series for Gudermannian