Difference between revisions of "Prime counting"
From specialfunctionswiki
(→Properties) |
|||
| Line 9: | Line 9: | ||
=Properties= | =Properties= | ||
| − | [[Prime number theorem, pi and x/log(x)]] | + | [[Prime number theorem, pi and x/log(x)]]<br /> |
| − | [[Prime number theorem, logarithmic integral]] | + | [[Prime number theorem, logarithmic integral]]<br /> |
=References= | =References= | ||
Revision as of 19:37, 9 June 2016
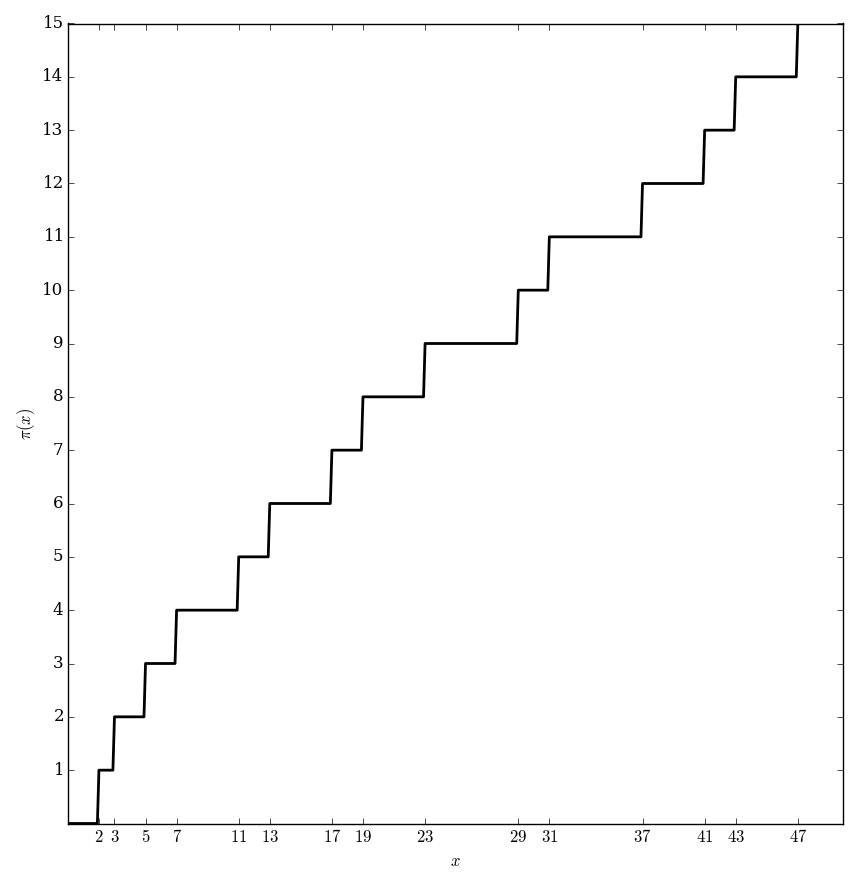
The prime counting function $\pi \colon \mathbb{R} \rightarrow \mathbb{Z}^+$ is defined by the formula $$\pi(x) = \{\mathrm{number \hspace{2pt} of \hspace{2pt} primes} \leq x \}.$$
Properties
Prime number theorem, pi and x/log(x)
Prime number theorem, logarithmic integral