Difference between revisions of "Reciprocal gamma"
From specialfunctionswiki
| Line 13: | Line 13: | ||
=Properties= | =Properties= | ||
| + | [[Reciprocal gamma is entire]]<br /> | ||
[[Reciprocal gamma written as an infinite product]]<br /> | [[Reciprocal gamma written as an infinite product]]<br /> | ||
[[Contour integral representation of reciprocal gamma]]<br /> | [[Contour integral representation of reciprocal gamma]]<br /> | ||
Latest revision as of 10:50, 11 January 2017
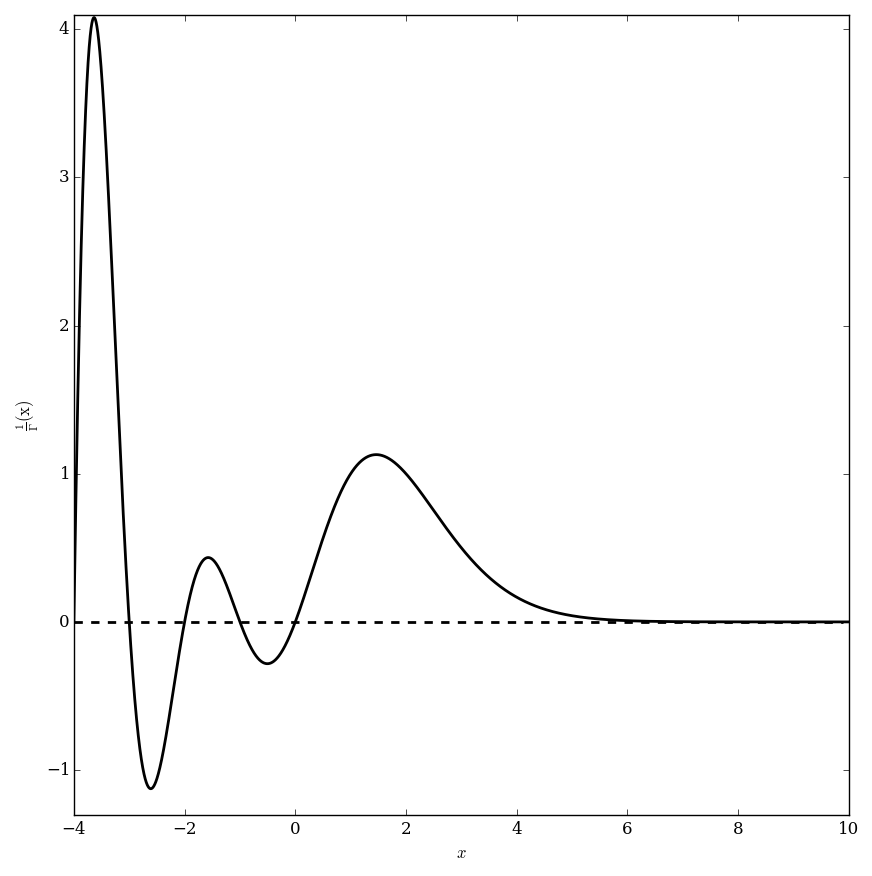
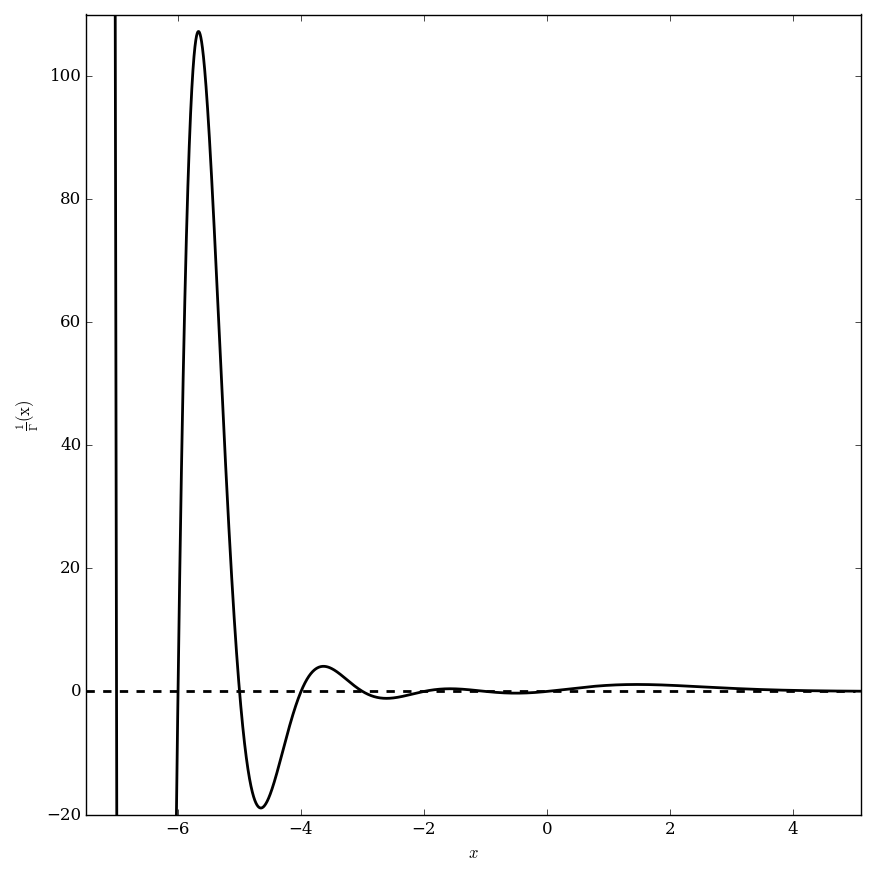
The reciprocal gamma function $\dfrac{1}{\Gamma}$ is defined by $$\left( \dfrac{1}{\Gamma} \right)(z) =\dfrac{1}{\Gamma(z)},$$ where $\Gamma$ denotes the gamma function.
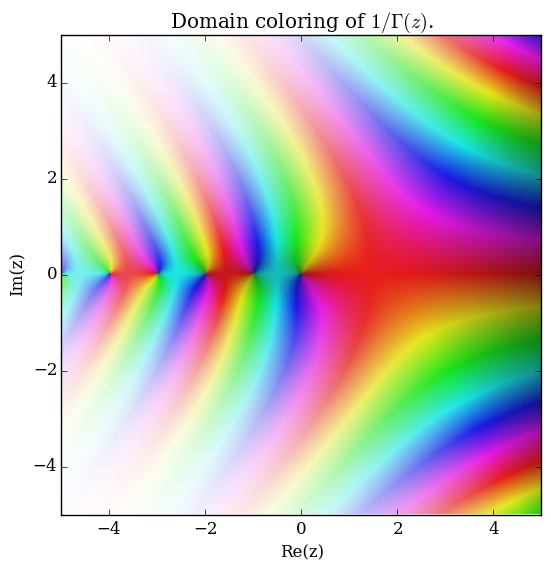
Domain coloring of $\dfrac{1}{\Gamma}$.
Plot of $\Gamma$ and $\dfrac{1}{\Gamma}$ from Abramowitz&Stegun.
Properties
Reciprocal gamma is entire
Reciprocal gamma written as an infinite product
Contour integral representation of reciprocal gamma