Difference between revisions of "Spherical Bessel y"
From specialfunctionswiki
(→Properties) |
|||
| Line 2: | Line 2: | ||
$$y_{\nu}(z)=\sqrt{\dfrac{\pi}{2z}} Y_{\nu+\frac{1}{2}}(z),$$ | $$y_{\nu}(z)=\sqrt{\dfrac{\pi}{2z}} Y_{\nu+\frac{1}{2}}(z),$$ | ||
where $Y_{\nu}$ denotes the [[Bessel Y sub nu|Bessel function of the second kind]]. | where $Y_{\nu}$ denotes the [[Bessel Y sub nu|Bessel function of the second kind]]. | ||
| + | |||
| + | <div align="center"> | ||
| + | <gallery> | ||
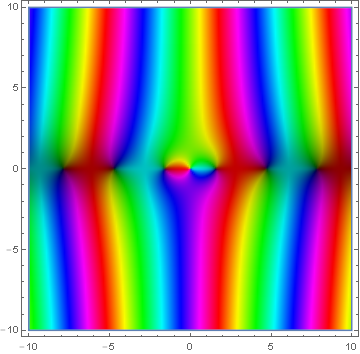
| + | File:Domcolsphericalbesselysub0.png|[[Domain coloring]] of $y_0$. | ||
| + | </gallery> | ||
| + | </div> | ||
=Properties= | =Properties= | ||
Revision as of 02:59, 21 August 2015
The spherical Bessel function of the second kind is $$y_{\nu}(z)=\sqrt{\dfrac{\pi}{2z}} Y_{\nu+\frac{1}{2}}(z),$$ where $Y_{\nu}$ denotes the Bessel function of the second kind.
Domain coloring of $y_0$.
Contents
Properties
Theorem
The following formula holds for non-negative integers $n$: $$y_n(z)=(-1)^{n+1}z^n \left( \dfrac{1}{z} \dfrac{d}{dz} \right)^n \left( \dfrac{\cos z}{z} \right),$$ where $y_n$ denotes the spherical Bessel function of the second kind and $\cos$ denotes the cosine function.
Proof
References
Spherical Bessel $y_{\nu}$