Difference between revisions of "Inverse Gudermannian"
(→Properties) |
|||
| Line 11: | Line 11: | ||
=Properties= | =Properties= | ||
| + | <div class="toccolours mw-collapsible mw-collapsed"> | ||
| + | <strong>Theorem:</strong> The following formula holds: | ||
| + | $$\sinh(\mathrm{gd}^{-1}(x))=\tan(x),$$ | ||
| + | where $\sinh$ is the [[sinh|hyperbolic sine]], $\mathrm{gd}^{-1}$ is the [[inverse Gudermannian]], and $\tan$ is the [[tangent]]. | ||
| + | <div class="mw-collapsible-content"> | ||
| + | <strong>Proof:</strong> █ | ||
| + | </div> | ||
| + | </div> | ||
| + | |||
| + | <div class="toccolours mw-collapsible mw-collapsed"> | ||
| + | <strong>Theorem:</strong> The following formula holds: | ||
| + | $$\cosh(\mathrm{gd}^{-1}(x))=\sec(x),$$ | ||
| + | where $\cosh$ is the [[cosh|hyperbolic cosine]], $\mathrm{gd}^{-1}$ is the [[inverse Gudermannian]], and $\sec$ is the [[secant]]. | ||
| + | <div class="mw-collapsible-content"> | ||
| + | <strong>Proof:</strong> █ | ||
| + | </div> | ||
| + | </div> | ||
| + | |||
| + | <div class="toccolours mw-collapsible mw-collapsed"> | ||
| + | <strong>Theorem:</strong> The following formula holds: | ||
| + | $$\mathrm{tanh}(\mathrm{gd}^{-1}(x))=\sin(x),$$ | ||
| + | where $\mathrm{tanh}$ is the [[tanh|hyperbolic tangent]], $\mathrm{gd}^{-1}$ is the [[inverse Gudermannian]], and $\sin$ is the [[sine]]. | ||
| + | <div class="mw-collapsible-content"> | ||
| + | <strong>Proof:</strong> █ | ||
| + | </div> | ||
| + | </div> | ||
| + | |||
| + | <div class="toccolours mw-collapsible mw-collapsed"> | ||
| + | <strong>Theorem:</strong> The following formula holds: | ||
| + | $$\mathrm{csch}(\mathrm{gd}^{-1}(x))=\cot(x),$$ | ||
| + | where $\mathrm{csch}$ is the [[csch|hyperbolic cosecant]], $\mathrm{gd}^{-1}$ is the [[inverse Gudermannian]], and $\cot$ is the [[cotangent]]. | ||
| + | <div class="mw-collapsible-content"> | ||
| + | <strong>Proof:</strong> █ | ||
| + | </div> | ||
| + | </div> | ||
| + | |||
| + | <div class="toccolours mw-collapsible mw-collapsed"> | ||
| + | <strong>Theorem:</strong> The following formula holds: | ||
| + | $$\mathrm{sech}(\mathrm{gd}^{-1}(x))=\cos(x),$$ | ||
| + | where $\mathrm{sech}$ is the [[sech|hyperbolic secant]], $\mathrm{gd}^{-1}$ is the [[inverse Gudermannian]], and $\cos$ is the [[cosine]]. | ||
| + | <div class="mw-collapsible-content"> | ||
| + | <strong>Proof:</strong> █ | ||
| + | </div> | ||
| + | </div> | ||
| + | |||
| + | <div class="toccolours mw-collapsible mw-collapsed"> | ||
| + | <strong>Theorem:</strong> The following formula holds: | ||
| + | $$\mathrm{coth}(\mathrm{gd}^{-1}(x))=\csc(x),$$ | ||
| + | where $\mathrm{coth}$ is the [[coth|hyperbolic cotangent]], $\mathrm{gd}^{-1}$ is the [[inverse Gudermannian]], and $\csc$ is the [[cosecant]]. | ||
| + | <div class="mw-collapsible-content"> | ||
| + | <strong>Proof:</strong> █ | ||
| + | </div> | ||
| + | </div> | ||
Revision as of 23:30, 25 August 2015
The inverse Gudermannian $\mathrm{gd}^{-1}$ is the inverse function of the Gudermannian function. It may be defined by the following formula for $x \in \mathbb{R}$: $$\mathrm{gd}^{-1}(x)=\displaystyle\int_0^x \dfrac{1}{\cosh(t)} dt,$$ where $\cosh$ denotes the hyperbolic cosine.
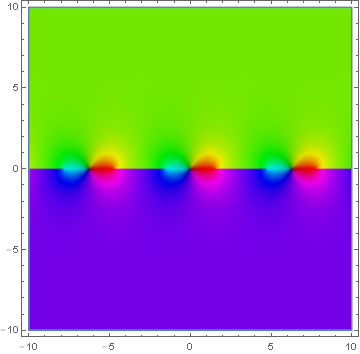
Domain coloring of $\mathrm{gd}^{-1}$.
Properties
Theorem: The following formula holds: $$\sinh(\mathrm{gd}^{-1}(x))=\tan(x),$$ where $\sinh$ is the hyperbolic sine, $\mathrm{gd}^{-1}$ is the inverse Gudermannian, and $\tan$ is the tangent.
Proof: █
Theorem: The following formula holds: $$\cosh(\mathrm{gd}^{-1}(x))=\sec(x),$$ where $\cosh$ is the hyperbolic cosine, $\mathrm{gd}^{-1}$ is the inverse Gudermannian, and $\sec$ is the secant.
Proof: █
Theorem: The following formula holds: $$\mathrm{tanh}(\mathrm{gd}^{-1}(x))=\sin(x),$$ where $\mathrm{tanh}$ is the hyperbolic tangent, $\mathrm{gd}^{-1}$ is the inverse Gudermannian, and $\sin$ is the sine.
Proof: █
Theorem: The following formula holds: $$\mathrm{csch}(\mathrm{gd}^{-1}(x))=\cot(x),$$ where $\mathrm{csch}$ is the hyperbolic cosecant, $\mathrm{gd}^{-1}$ is the inverse Gudermannian, and $\cot$ is the cotangent.
Proof: █
Theorem: The following formula holds: $$\mathrm{sech}(\mathrm{gd}^{-1}(x))=\cos(x),$$ where $\mathrm{sech}$ is the hyperbolic secant, $\mathrm{gd}^{-1}$ is the inverse Gudermannian, and $\cos$ is the cosine.
Proof: █
Theorem: The following formula holds: $$\mathrm{coth}(\mathrm{gd}^{-1}(x))=\csc(x),$$ where $\mathrm{coth}$ is the hyperbolic cotangent, $\mathrm{gd}^{-1}$ is the inverse Gudermannian, and $\csc$ is the cosecant.
Proof: █