Difference between revisions of "Fresnel C"
From specialfunctionswiki
| Line 1: | Line 1: | ||
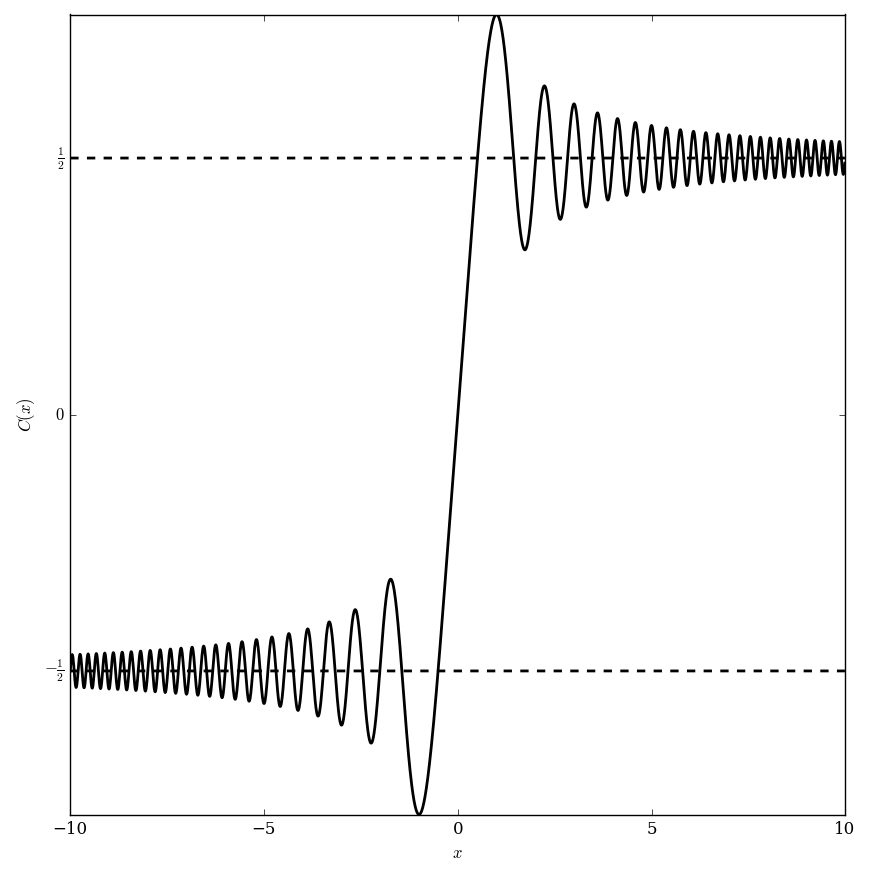
The Fresnel C function is defined by the formula | The Fresnel C function is defined by the formula | ||
$$C(x)=\int_0^x \cos(t^2) \mathrm{d}t.$$ | $$C(x)=\int_0^x \cos(t^2) \mathrm{d}t.$$ | ||
| − | (Note in Abramowitz&Stegun it [http:// | + | (Note in Abramowitz&Stegun it [http://specialfunctionswiki.org/mirror/abramowitz_and_stegun-1.03/page_300.htm is defined] differently.) |
<div align="center"> | <div align="center"> | ||
<gallery> | <gallery> | ||
Revision as of 08:06, 8 June 2016
The Fresnel C function is defined by the formula $$C(x)=\int_0^x \cos(t^2) \mathrm{d}t.$$ (Note in Abramowitz&Stegun it is defined differently.)
Domain coloring of Fresnel $C$.
See Also
Videos
How to integrate cos(x^2) - The Fresnel Integral C(x)