Difference between revisions of "Cosh"
From specialfunctionswiki
| Line 6: | Line 6: | ||
<div align="center"> | <div align="center"> | ||
<gallery> | <gallery> | ||
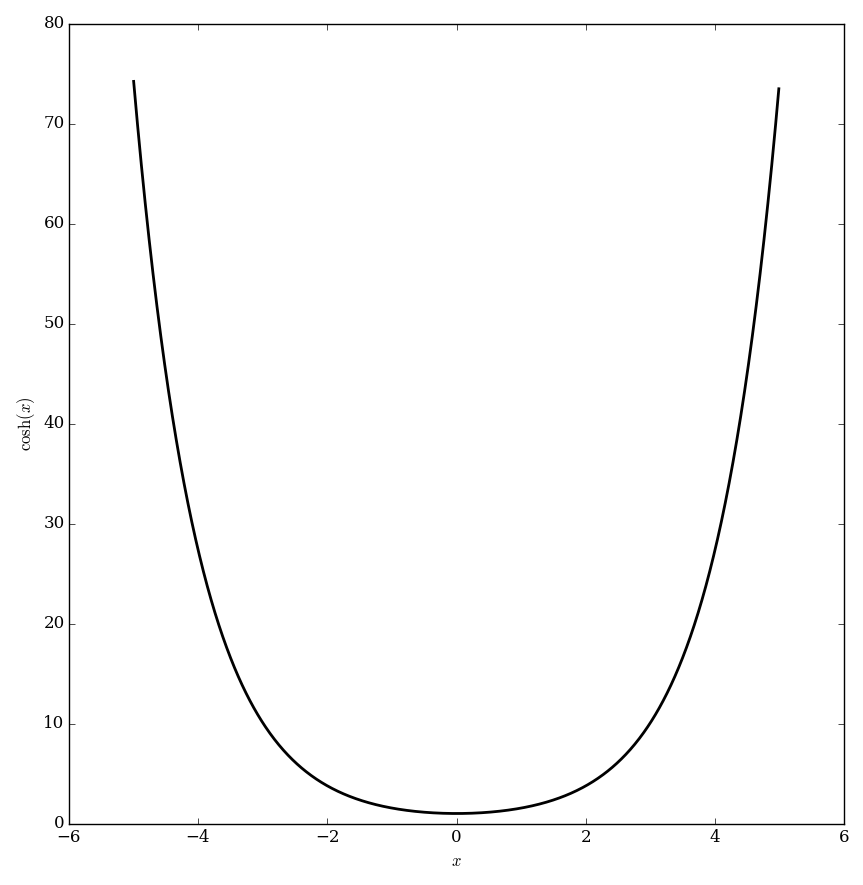
| − | File:Coshplot.png| | + | File:Coshplot.png|Graph of $\cosh$. |
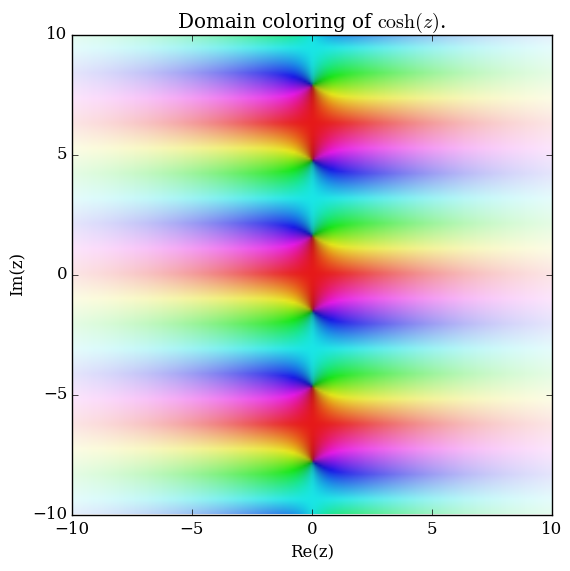
File:Complexcoshplot.png|[[Domain coloring]] of [[analytic continuation]] of $\cosh$. | File:Complexcoshplot.png|[[Domain coloring]] of [[analytic continuation]] of $\cosh$. | ||
</gallery> | </gallery> | ||
Revision as of 06:51, 9 June 2016
The hyperbolic cosine function $\cosh \colon \mathbb{C} \rightarrow \mathbb{C}$ is defined by
$$\mathrm{cosh}(z)=\dfrac{e^z + e^{-z}}{2}$$
Domain coloring of analytic continuation of $\cosh$.
Properties
Derivative of cosh
Pythagorean identity for sinh and cosh
Weierstrass factorization of cosh
Relationship between cosh and hypergeometric 0F1
Relationship between Bessel I sub 1/2 and cosh
Relationship between cosh and cos
Relationship between cos and cosh
Relationship between secant, Gudermannian, and cosh
Relationship between cosh, inverse Gudermannian, and sec