Difference between revisions of "Modified Bessel K"
From specialfunctionswiki
| Line 12: | Line 12: | ||
=Properties= | =Properties= | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
[[Relationship between Airy Ai and modified Bessel K]] | [[Relationship between Airy Ai and modified Bessel K]] | ||
| − | |||
| − | |||
=References= | =References= | ||
| Line 28: | Line 18: | ||
[[Category:SpecialFunction]] | [[Category:SpecialFunction]] | ||
| + | |||
| + | {{:Bessel functions footer}} | ||
Revision as of 19:15, 10 June 2016
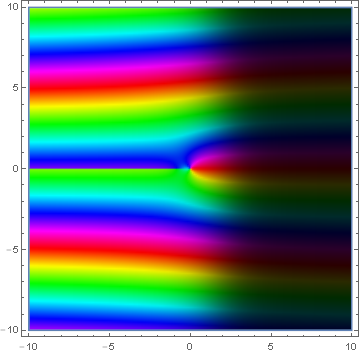
The modified Bessel function of the second kind is defined by $$K_{\nu}(z)=\dfrac{\pi}{2} \dfrac{I_{-\nu}(z)-I_{\nu}(z)}{\sin(\nu \pi)},$$ where $I_{\nu}$ is the modified Bessel function of the first kind.
Domain coloring of $K_1(z)$.
Modified Bessel functions from Abramowitz&Stegun.
.jpg)
Properties
Relationship between Airy Ai and modified Bessel K
