Difference between revisions of "Exponential integral E"
From specialfunctionswiki
| Line 1: | Line 1: | ||
__NOTOC__ | __NOTOC__ | ||
| − | The exponential integral functions $E_n$ are defined by | + | The exponential integral functions $E_n$ are defined for $\left|\mathrm{arg \hspace{2pt}}z\right|<\pi$ by |
| − | $$E_1(z) = \displaystyle\int_1^{\infty} \dfrac{e^{-t}}{t} \mathrm{d}t | + | $$E_1(z) = \displaystyle\int_1^{\infty} \dfrac{e^{-t}}{t} \mathrm{d}t,$$ |
and | and | ||
$$E_n(z)=\displaystyle\int_1^{\infty} \dfrac{e^{-zt}}{t^n} \mathrm{d}t.$$ | $$E_n(z)=\displaystyle\int_1^{\infty} \dfrac{e^{-zt}}{t^n} \mathrm{d}t.$$ | ||
Revision as of 00:22, 19 June 2016
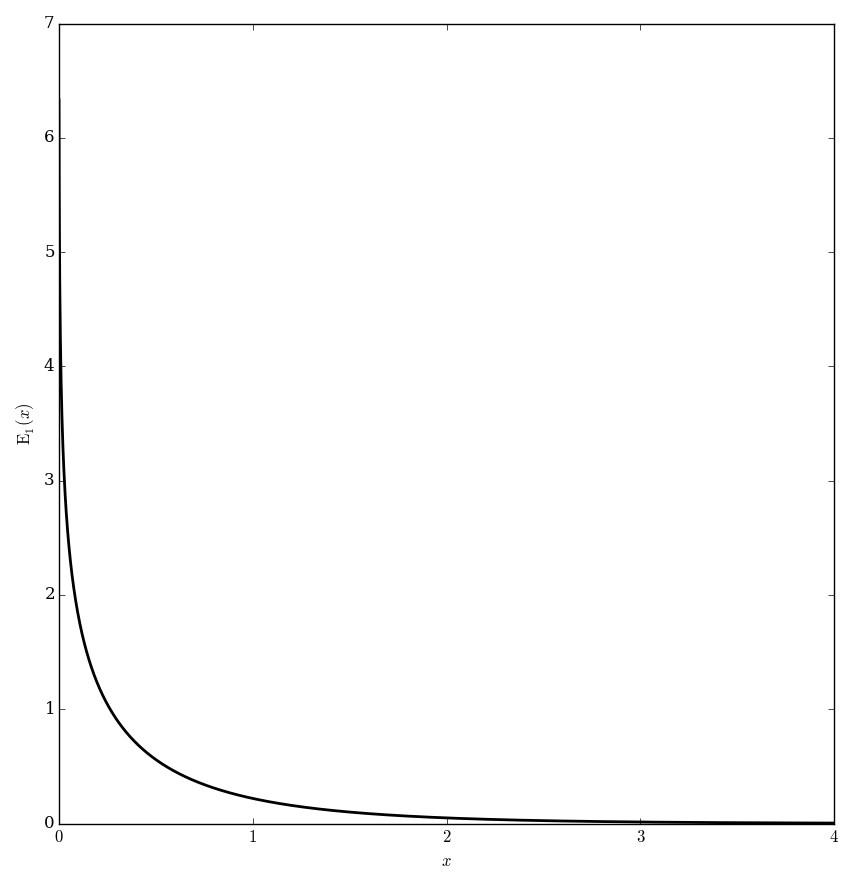
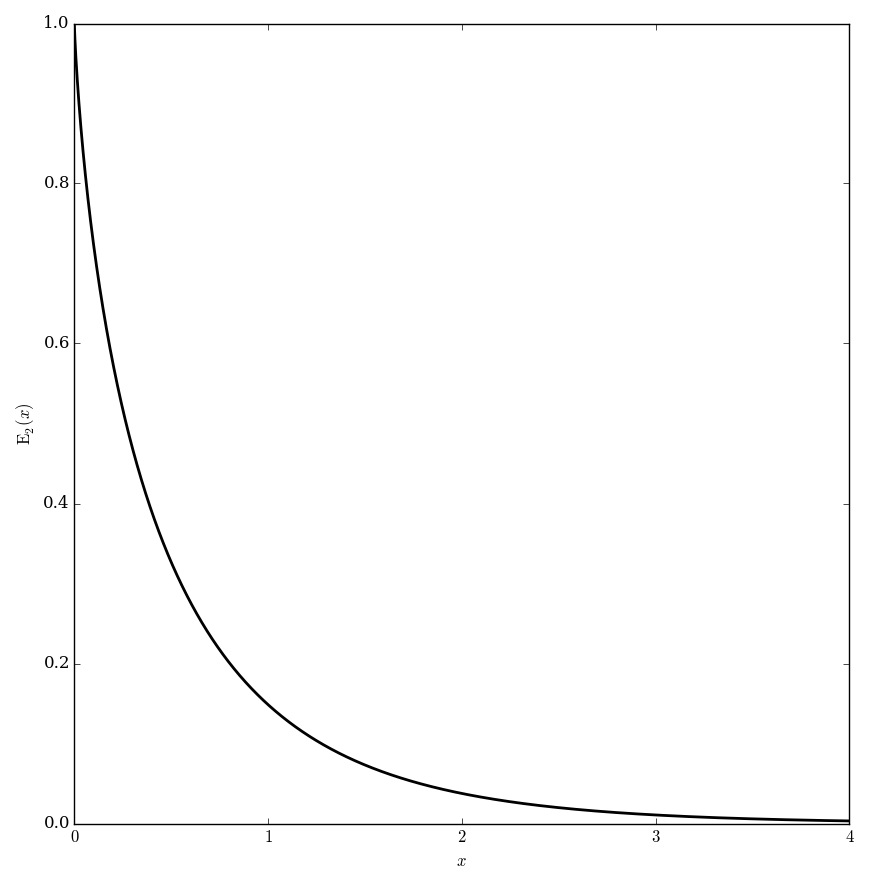
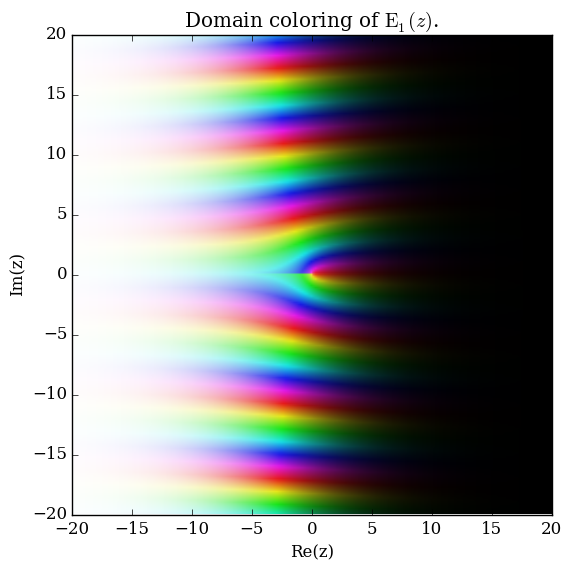
The exponential integral functions $E_n$ are defined for $\left|\mathrm{arg \hspace{2pt}}z\right|<\pi$ by $$E_1(z) = \displaystyle\int_1^{\infty} \dfrac{e^{-t}}{t} \mathrm{d}t,$$ and $$E_n(z)=\displaystyle\int_1^{\infty} \dfrac{e^{-zt}}{t^n} \mathrm{d}t.$$
Graph of $\mathrm{E}_1$.
Graph of $\mathrm{E}_2$.
Graph of $\mathrm{E}_3$.
Domain coloring of $\mathrm{E}_1$.
Domain coloring of $\mathrm{E}_2$.
Properties
Relationship between the exponential integral and upper incomplete gamma function
Videos
Laplace transform of exponential integral
References
Exponential Integral and Related Functions
