Difference between revisions of "Airy Ai"
(→Videos) |
(→References) |
||
| Line 27: | Line 27: | ||
[http://www.ams.org/journals/mcom/1979-33-145/S0025-5718-1979-0514831-8/S0025-5718-1979-0514831-8.pdf Tables of Weyl Fractional Integrals for the Airy Function]<br /> | [http://www.ams.org/journals/mcom/1979-33-145/S0025-5718-1979-0514831-8/S0025-5718-1979-0514831-8.pdf Tables of Weyl Fractional Integrals for the Airy Function]<br /> | ||
[http://www.amazon.com/Special-Functions-Introduction-Classical-Mathematical/dp/0471113131 Special Functions: An Introduction to the Classical Functions of Mathematical Physics]<br /> | [http://www.amazon.com/Special-Functions-Introduction-Classical-Mathematical/dp/0471113131 Special Functions: An Introduction to the Classical Functions of Mathematical Physics]<br /> | ||
| − | |||
=See Also= | =See Also= | ||
Latest revision as of 02:00, 18 December 2016
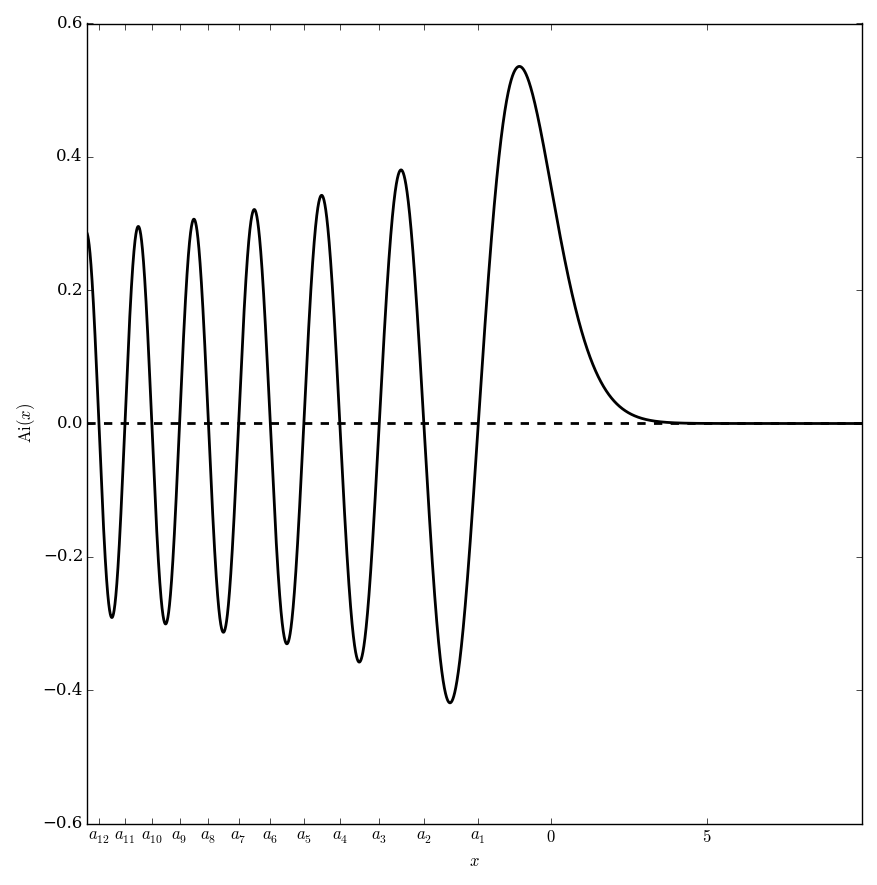
The Airy function $\mathrm{Ai}$ is a solution of the Airy differential equation linearly independent from the Airy Bi function.
Graph of the Airy $\mathrm{Ai}$ function.
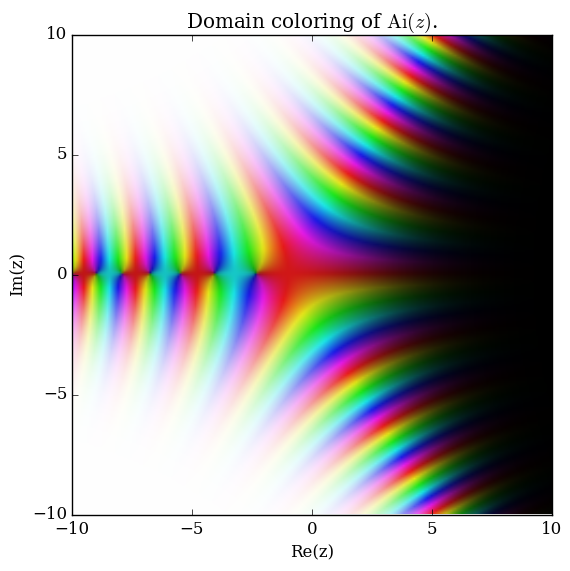
Domain coloring of Airy $\mathrm{Ai}$.
Properties
Integral representation of Airy Ai
Value of Ai(0)
Value of Ai'(0)
Relationship between Airy Ai and modified Bessel K
Relationship between Scorer Gi and Airy functions
Relationship between Scorer Hi and Airy functions
Videos
Leading Tsunami wave reaching the shore (27 November 2009)
Series solution of ode: Airy's equation (3 November 2010)
Airy differential equation (26 November 2013)
References
The mathematics of rainbows
Tables of Weyl Fractional Integrals for the Airy Function
Special Functions: An Introduction to the Classical Functions of Mathematical Physics
