Difference between revisions of "Arcsin"
From specialfunctionswiki
(→Properties) |
(→Videos) |
||
| Line 19: | Line 19: | ||
=Videos= | =Videos= | ||
| − | [https://www.youtube.com/watch?v=JGU74wbZMLg Inverse Trig Functions: Arcsin]<br /> | + | [https://www.youtube.com/watch?v=JGU74wbZMLg Inverse Trig Functions: Arcsin (1 October 2009)]<br /> |
| − | [https://www.youtube.com/watch?v= | + | [https://www.youtube.com/watch?v=JZ9Ku1TTeA4 What is arcsin(x)? (18 August 2011)]<br /> |
| − | [https://www.youtube.com/watch?v= | + | [https://www.youtube.com/watch?v=KmHD7CsOw5Y Integrate x*arcsin(x) (25 February 2013)]<br /> |
| − | [https://www.youtube.com/watch?v=4CY7RIUhs2s What is the inverse of arcsin(ln(x))?]<br /> | + | [https://www.youtube.com/watch?v=4CY7RIUhs2s What is the inverse of arcsin(ln(x))? (28 April 2014)]<br /> |
=See Also= | =See Also= | ||
Latest revision as of 23:45, 22 December 2016
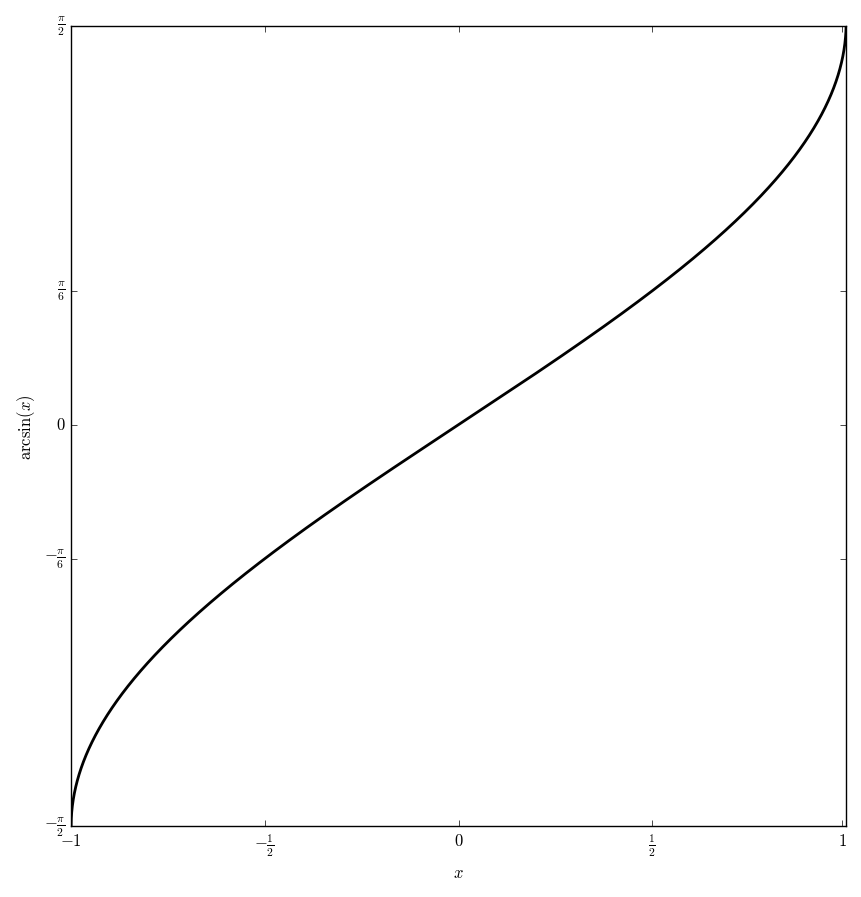
The function $\mathrm{arcsin} \colon \mathbb{C} \setminus \left\{ (-\infty,-1) \bigcup (1,\infty) \right\} \rightarrow \mathbb{C}$ is defined by
$$\rm{arcsin}(z)=-i \log \left( iz + \sqrt{1-z^2} \right),$$
where $i$ denotes the imaginary number and $\log$ denotes the logarithm.
Graph of $\mathrm{arcsin}$ on $[-1,1]$.
Domain coloring of $\mathrm{arcsin}$.
Properties
Arcsin as inverse sine
Derivative of arcsin
Antiderivative of arcsin
Relationship between arcsin and arccsc
2F1(1/2,1/2;3/2;z^2)=arcsin(z)/z
Videos
Inverse Trig Functions: Arcsin (1 October 2009)
What is arcsin(x)? (18 August 2011)
Integrate x*arcsin(x) (25 February 2013)
What is the inverse of arcsin(ln(x))? (28 April 2014)
See Also
References
On the function arc sin(x+iy)-Cayley
