Difference between revisions of "Lambert W"
From specialfunctionswiki
(→Videos) |
|||
| Line 1: | Line 1: | ||
| + | __NOTOC__ | ||
The Lambert $W$ function is the (multi-valued) inverse of the function $f(x)=xe^{x}$. | The Lambert $W$ function is the (multi-valued) inverse of the function $f(x)=xe^{x}$. | ||
| Line 8: | Line 9: | ||
</gallery> | </gallery> | ||
</div> | </div> | ||
| + | |||
| + | =Properties= | ||
| + | |||
| + | =Videos= | ||
| + | [https://www.youtube.com/watch?v=AJD8kh3DSAM 6: Recursion, Infinite Tetrations and the Lambert W Function (4 August 2014)] | ||
=References= | =References= | ||
[http://arxiv.org/pdf/1003.1628.pdf Having fun with the Lambert $W(x)$ function] | [http://arxiv.org/pdf/1003.1628.pdf Having fun with the Lambert $W(x)$ function] | ||
| − | + | ||
| − | |||
[[Category:SpecialFunction]] | [[Category:SpecialFunction]] | ||
Revision as of 00:46, 23 December 2016
The Lambert $W$ function is the (multi-valued) inverse of the function $f(x)=xe^{x}$.
Plot of the principal branch $W_0$.
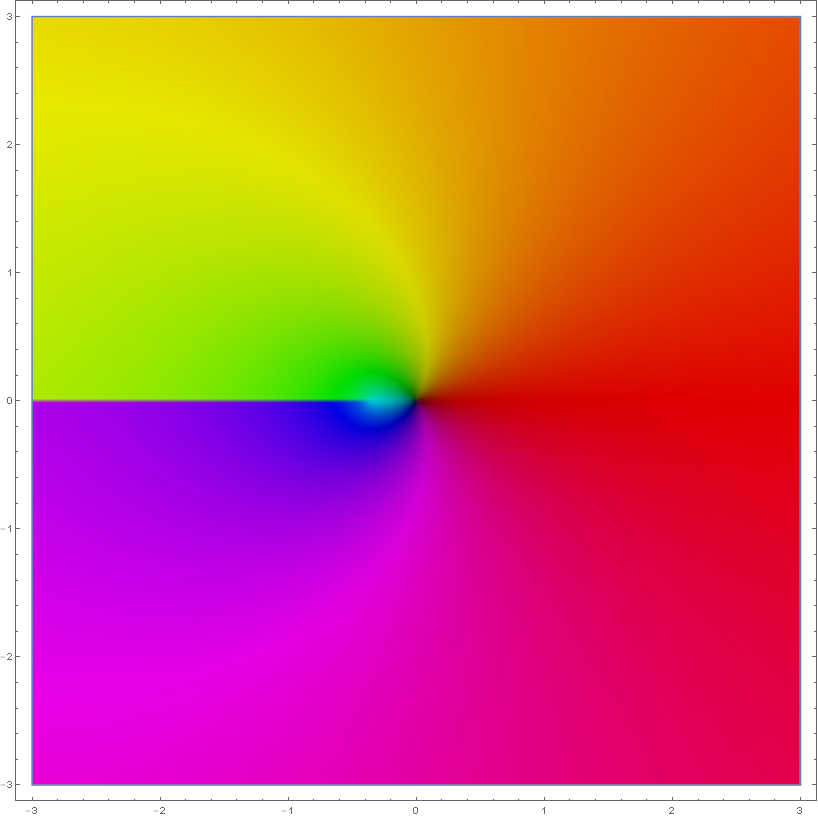
Domain coloring of analytic continuation of branch $W_0(x)$ to $\mathbb{C}$.
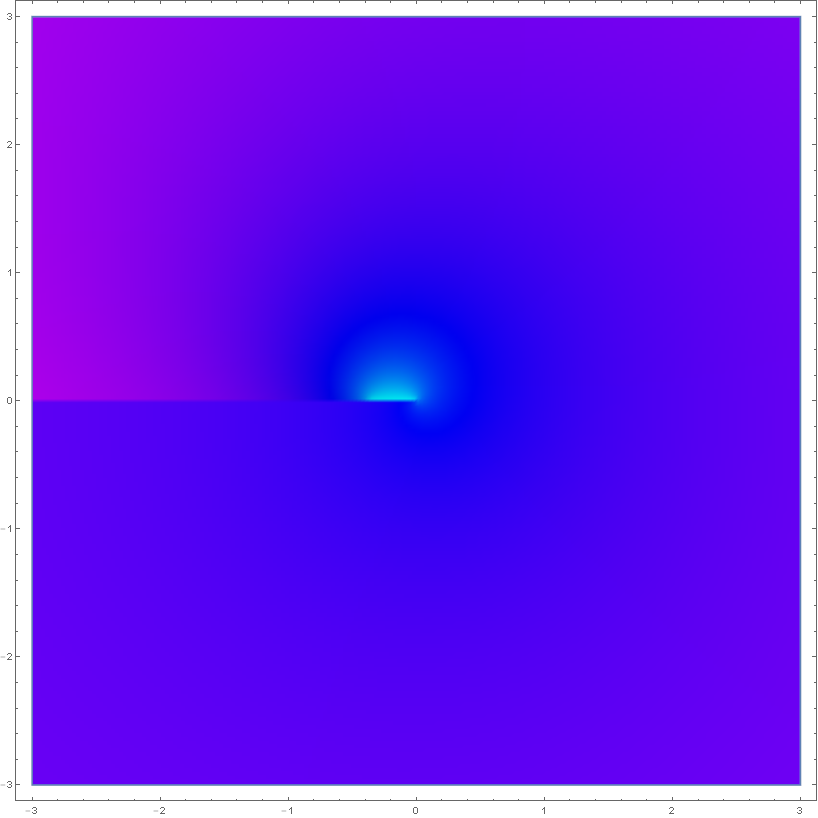
Domain coloring of analytic continuation of branch $W_{-1}(x)$ to $\mathbb{C}$.
Properties
Videos
6: Recursion, Infinite Tetrations and the Lambert W Function (4 August 2014)
