The sine integral is defined by $$\mathrm{Si}(z) = \displaystyle\int_0^z \mathrm{sinc}(t) \mathrm{d}t; |\mathrm{arg} z|<\pi,$$ where $\mathrm{sinc}$ denotes the Sinc function.

Si.png

Graph of $\mathrm{Si}$.
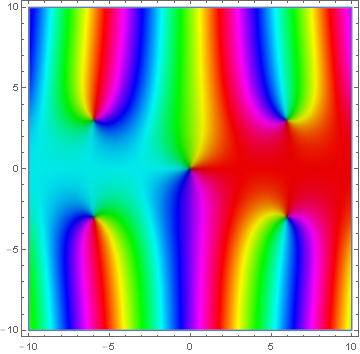
Domain coloring of analytic continuation of $\mathrm{Si}$.

Relationship to other functions

Theorem

The following formula holds: $$\mathrm{Ei}(ix)=\mathrm{Ci}(x)+i\mathrm{Si}(x),$$ where $\mathrm{Ei}$ denotes the exponential integral Ei, $\mathrm{Ci}$ denotes the cosine integral, and $\mathrm{Si}$ denotes the sine integral.