Sech
From specialfunctionswiki
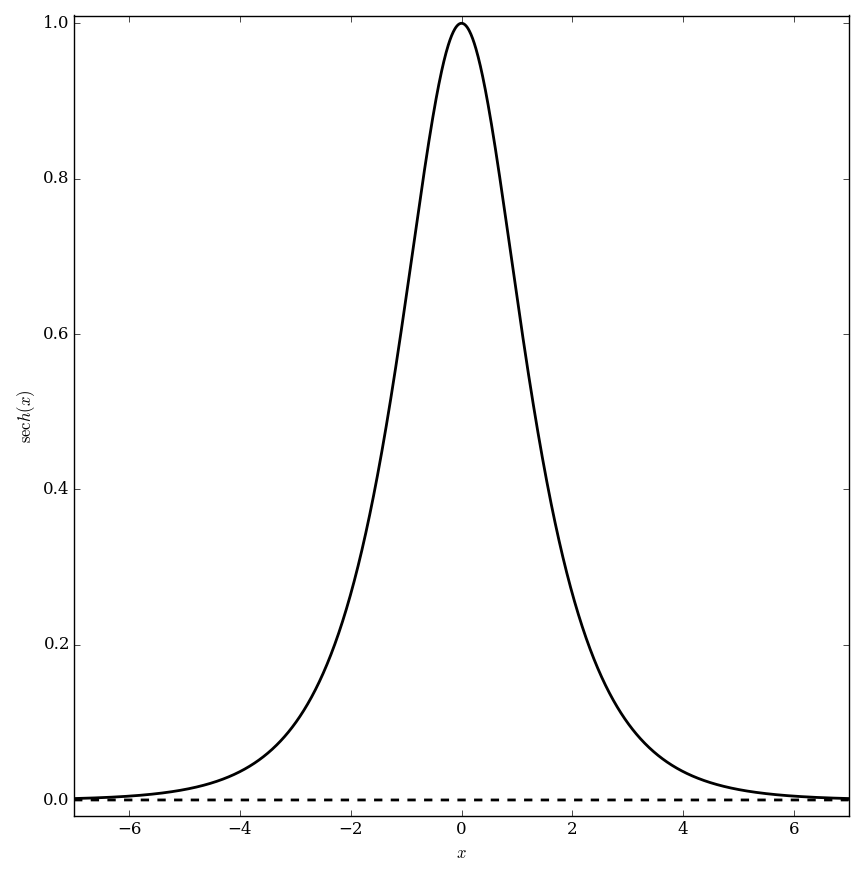
The hyperbolic secant function $\mathrm{sech} \colon \mathbb{R} \rightarrow (0,1]$ is defined by $$\mathrm{sech}(z)=\dfrac{1}{\cosh(z)}.$$ Since this function is not one-to-one, we define the inverse hyperbolic secant function as the inverse function of $\mathrm{sech}$ restricted to $[0,\infty)$.
Graph of $\mathrm{sech}$ on $[-5,5]$.
Domain coloring of analytic continuation of $\mathrm{sech}$.
Properties
Derivative of sech
Antiderivative of sech
Relationship between cosine, Gudermannian, and sech
Relationship between sech, inverse Gudermannian, and cos
