Darboux function

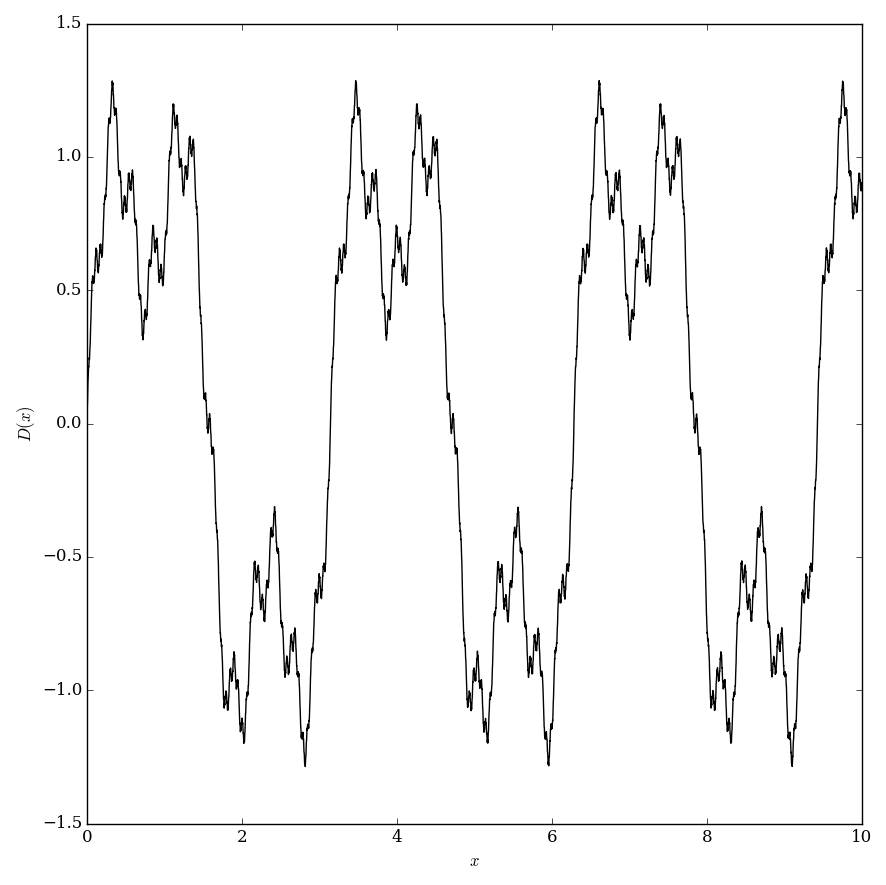
The Darboux function is defined by $$D(x)=\displaystyle\sum_{k=1}^{\infty} \dfrac{\sin\left((k+1)!x\right)}{k!},$$ where $\sin$ denotes the sine function.

• 

  Plot of $D(x)$ on $[0,5]$.

Properties

Theorem: The Darboux function is continuous on $\mathbb{R}$.

Proof: █

Theorem: The Darboux function is nowhere differentiable on $\mathbb{R}$.

Proof: █

References

[1]