Exponential integral Ei
From specialfunctionswiki
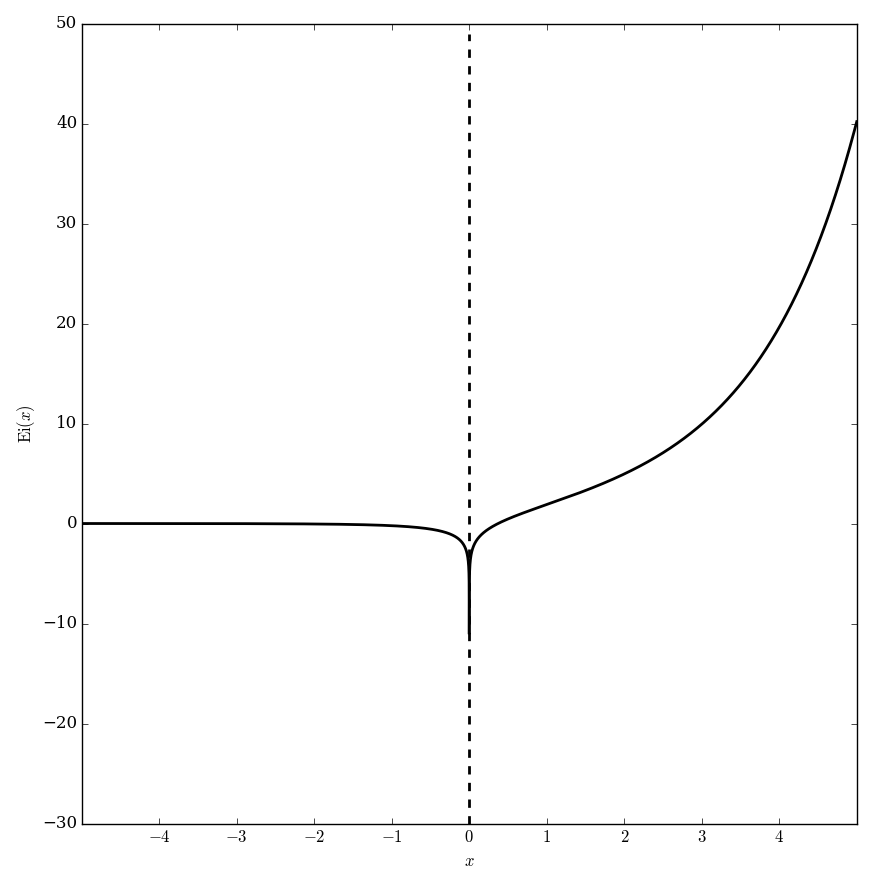
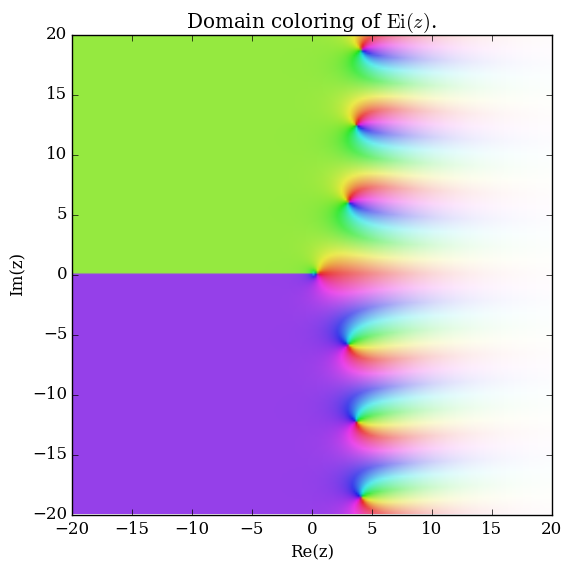
The exponential integral $\mathrm{Ei}$ is defined by $$\mathrm{Ei}(z) = \int_{-\infty}^x \dfrac{e^t}{t} \mathrm{d}t, \quad \left|\mathrm{arg}(-z) \right|<\pi.$$
Properties
Relationship between logarithmic integral and exponential integral
Exponential integral Ei series
Relationship between exponential integral Ei, cosine integral, and sine integral
See Also
References
- 1964: Milton Abramowitz and Irene A. Stegun: Handbook of mathematical functions ... (previous) ... (next): $5.1.2$
On certain definite integrals involving the exponential-integral - J.W.L. Glaisher