Dirichlet eta
From specialfunctionswiki
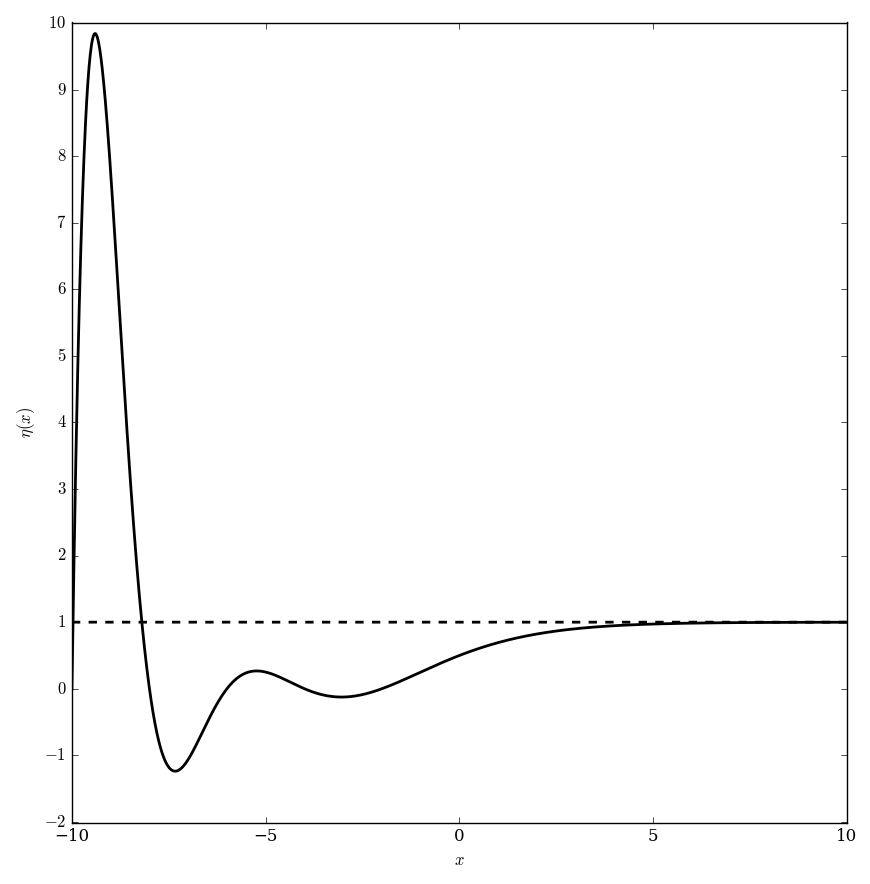
Let $\mathrm{Re} \hspace{2pt} z > 0$, then define $$\eta(z) = \displaystyle\sum_{n=1}^{\infty} \dfrac{(-1)^{n-1}}{n^s}.$$ This series is clearly the Riemann zeta function with alternating terms.
Graph of $\eta$.
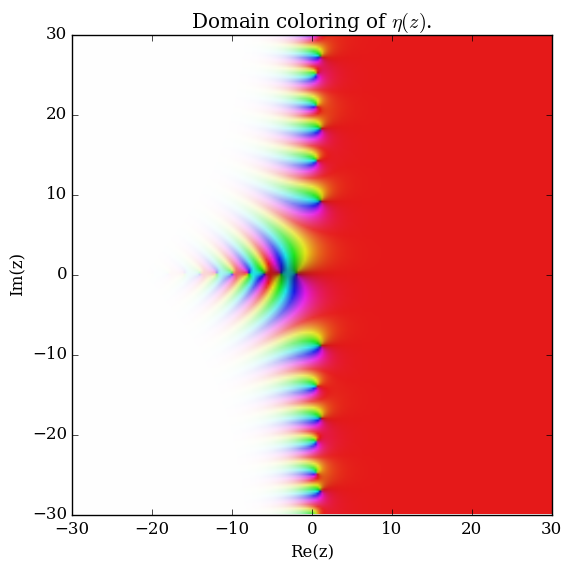
Domain coloring of $\eta$.
