Difference between revisions of "Polygamma"
From specialfunctionswiki
| Line 1: | Line 1: | ||
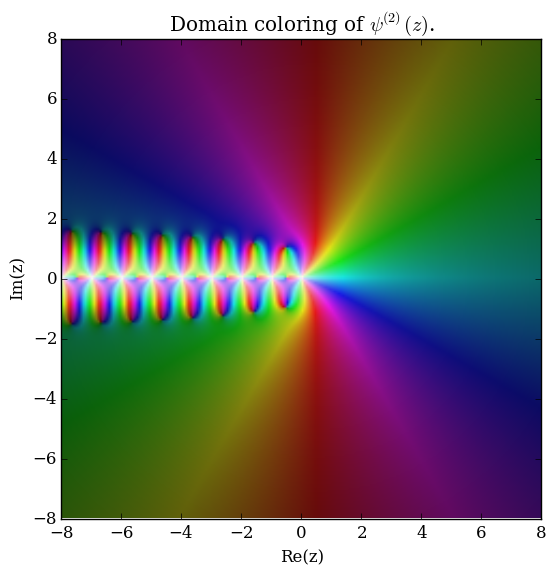
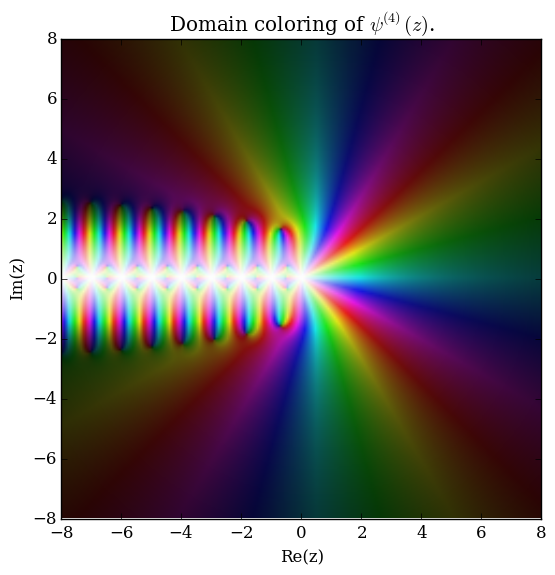
| − | The polygamma function of order $m$, $\psi^{(m)}(z) | + | The polygamma function of order $m$, $\psi^{(m)}(z)$, is defined by the formula |
$$\psi^{(m)}(z) = \dfrac{\mathrm{d}^{m+1}}{\mathrm{d}z^{m+1}} \log \Gamma(z),$$ | $$\psi^{(m)}(z) = \dfrac{\mathrm{d}^{m+1}}{\mathrm{d}z^{m+1}} \log \Gamma(z),$$ | ||
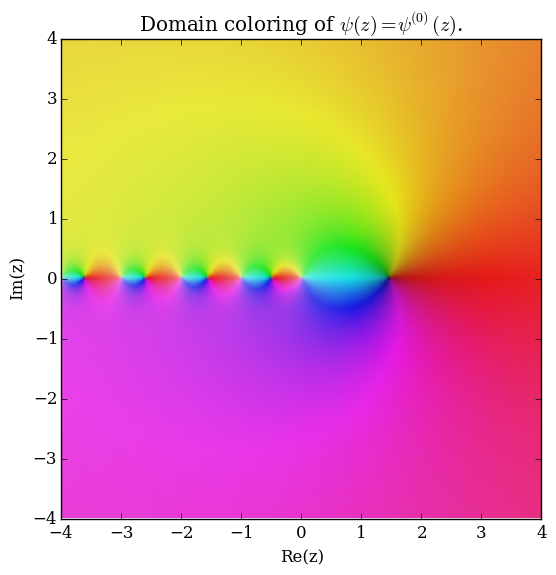
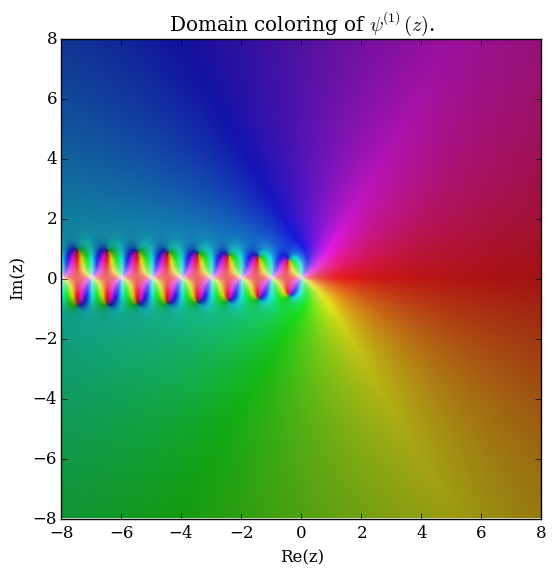
where $\log \Gamma$ denotes the [[loggamma]] function. The [[digamma]] function $\psi$ is the function $\psi^{(0)}(z)$ and the [[trigamma]] function is $\psi^{(1)}(z)$. | where $\log \Gamma$ denotes the [[loggamma]] function. The [[digamma]] function $\psi$ is the function $\psi^{(0)}(z)$ and the [[trigamma]] function is $\psi^{(1)}(z)$. | ||
| Line 27: | Line 27: | ||
=References= | =References= | ||
| − | * {{BookReference|Handbook of mathematical functions|1964|Milton Abramowitz|author2=Irene A. Stegun|prev=findme|next=Integral | + | * {{BookReference|Handbook of mathematical functions|1964|Milton Abramowitz|author2=Irene A. Stegun|prev=findme|next=Integral representaiton of polygamma for Re(z) greater than 0}}: 6.4.1 |
[[Category:SpecialFunction]] | [[Category:SpecialFunction]] | ||
Revision as of 08:04, 11 June 2016
The polygamma function of order $m$, $\psi^{(m)}(z)$, is defined by the formula $$\psi^{(m)}(z) = \dfrac{\mathrm{d}^{m+1}}{\mathrm{d}z^{m+1}} \log \Gamma(z),$$ where $\log \Gamma$ denotes the loggamma function. The digamma function $\psi$ is the function $\psi^{(0)}(z)$ and the trigamma function is $\psi^{(1)}(z)$.
Domain coloring of $\psi^{(0)}(z)$.
Domain coloring of $\psi^{(1)}(z)$.
Domain coloring of $\psi^{(2)}(z)$.
Domain coloring of $\psi^{(3)}(z)$.
Domain coloring of $\psi^{(4)}(z)$.
Domain coloring of $\psi^{(5)}(z)$.
Properties
Integral representation of polygamma
Integral representation of polygamma 2
Polygamma recurrence relation
Polygamma reflection relation
Polygamma series representation
Relation between polygamma and Hurwitz zeta
See Also
References
- 1964: Milton Abramowitz and Irene A. Stegun: Handbook of mathematical functions ... (previous) ... (next): 6.4.1
