Difference between revisions of "Möbius"
m (Tom moved page Möbius function to Möbius) |
(→Videos) |
||
| (16 intermediate revisions by the same user not shown) | |||
| Line 1: | Line 1: | ||
| + | __NOTOC__ | ||
The Möbius function is the function $\mu$ defined by the formula | The Möbius function is the function $\mu$ defined by the formula | ||
$$\mu(n) = \left\{ \begin{array}{ll} | $$\mu(n) = \left\{ \begin{array}{ll} | ||
| Line 5: | Line 6: | ||
0 &; n\mathrm{\hspace{2pt}has\hspace{2pt}a\hspace{2pt}square\hspace{2pt}divisor}. | 0 &; n\mathrm{\hspace{2pt}has\hspace{2pt}a\hspace{2pt}square\hspace{2pt}divisor}. | ||
\end{array} \right.$$ | \end{array} \right.$$ | ||
| + | |||
| + | <div align="center"> | ||
| + | <gallery> | ||
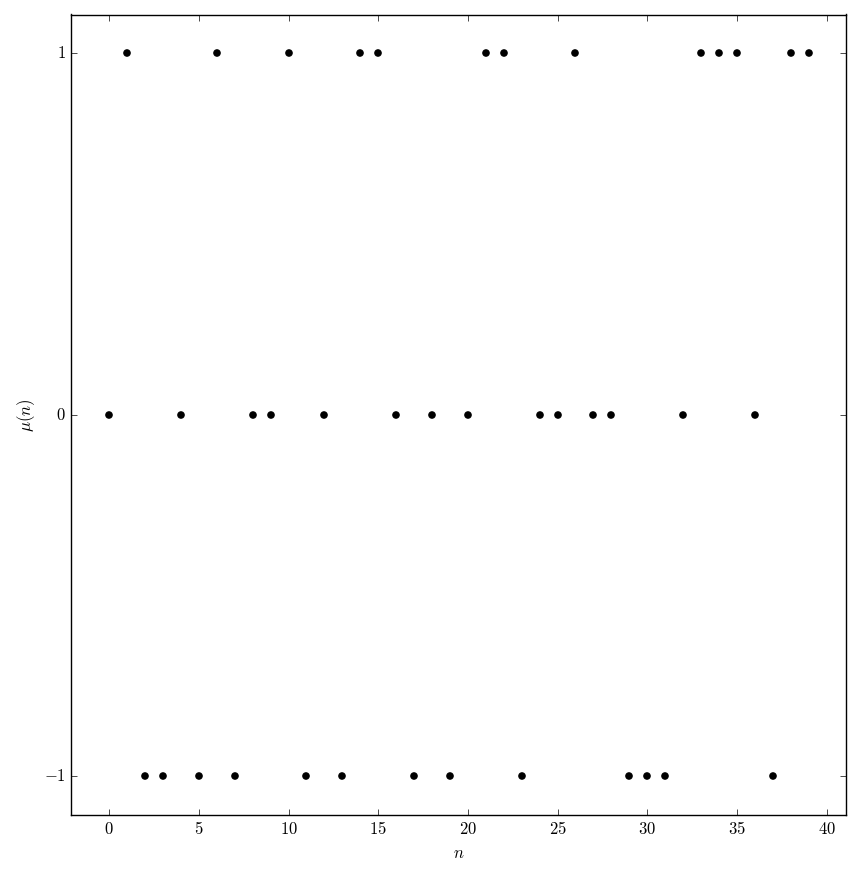
| + | File:Mobiusplot,on0to40.png|Graph of $\mu$ on $[0,40]$. | ||
| + | File:Mobiusplot,on0to100.png|Graph of $\mu$ on $[0,100]$. | ||
| + | </gallery> | ||
| + | </div> | ||
| + | |||
=Properties= | =Properties= | ||
| − | < | + | [[Reciprocal of Riemann zeta as a sum of Möbius function for Re(z) greater than 1]]<br /> |
| − | < | + | [[Relationship between prime zeta, Möbius function, logarithm, and Riemann zeta]]<br /> |
| − | + | ||
| − | + | =Videos= | |
| − | + | [https://youtu.be/zlRm1Lnz6fg?t=10 Möbius Function - Introduction (4 September 2007)]<br /> | |
| − | + | [https://www.youtube.com/watch?v=yiyuu9HiXUI Möbius Function - Merten's function (4 September 2007)]<br /> | |
| − | + | [https://www.youtube.com/watch?v=9Y5xokbMBSM Mobius Function Example (17 November 2012)]<br /> | |
| − | + | [https://www.youtube.com/watch?v=LyyLE5ROPXA Number Theory 27: Mobius function is multiplicative (8 January 2015)]<br /> | |
| + | [https://www.youtube.com/watch?v=Vsib1v5vfkc Möbius Inversion of $\zeta(s)$ (3 July 2016)]<br /> | ||
| + | |||
| + | =References= | ||
| + | * {{BookReference|Handbook of mathematical functions|1964|Milton Abramowitz|author2=Irene A. Stegun|prev=findme|next=Reciprocal of Riemann zeta as a sum of Möbius function for Re(z) greater than 1}}: $24.3.1 \mathrm{I}.A.$ | ||
| + | |||
| + | {{:Number theory functions footer}} | ||
| + | |||
| + | [[Category:SpecialFunction]] | ||
Latest revision as of 23:55, 8 December 2016
The Möbius function is the function $\mu$ defined by the formula $$\mu(n) = \left\{ \begin{array}{ll} 1 &; n \mathrm{\hspace{2pt}is\hspace{2pt}a\hspace{2pt}squarefree\hspace{2pt}positive\hspace{2pt}integer\hspace{2pt}with\hspace{2pt}even\hspace{2pt}number\hspace{2pt}of\hspace{2pt}prime\hspace{2pt}factors} \\ -1 &; n \mathrm{\hspace{2pt}is\hspace{2pt}a\hspace{2pt}squarefree\hspace{2pt}positive\hspace{2pt}integer\hspace{2pt}with\hspace{2pt}odd\hspace{2pt}number\hspace{2pt}of\hspace{2pt}prime\hspace{2pt}factors} \\ 0 &; n\mathrm{\hspace{2pt}has\hspace{2pt}a\hspace{2pt}square\hspace{2pt}divisor}. \end{array} \right.$$
Properties
Reciprocal of Riemann zeta as a sum of Möbius function for Re(z) greater than 1
Relationship between prime zeta, Möbius function, logarithm, and Riemann zeta
Videos
Möbius Function - Introduction (4 September 2007)
Möbius Function - Merten's function (4 September 2007)
Mobius Function Example (17 November 2012)
Number Theory 27: Mobius function is multiplicative (8 January 2015)
Möbius Inversion of $\zeta(s)$ (3 July 2016)
References
- 1964: Milton Abramowitz and Irene A. Stegun: Handbook of mathematical functions ... (previous) ... (next): $24.3.1 \mathrm{I}.A.$