Difference between revisions of "Cosecant"
From specialfunctionswiki
(→Properties) |
|||
| Line 13: | Line 13: | ||
=Properties= | =Properties= | ||
[[Derivative of cosecant]] <br /> | [[Derivative of cosecant]] <br /> | ||
| + | [[Derivative of cotangent]]<br /> | ||
[[Relationship between csch and csc]]<br /> | [[Relationship between csch and csc]]<br /> | ||
[[Relationship between csc, Gudermannian, and coth]] <br /> | [[Relationship between csc, Gudermannian, and coth]] <br /> | ||
[[Relationship between coth, inverse Gudermannian, and csc]]<br /> | [[Relationship between coth, inverse Gudermannian, and csc]]<br /> | ||
| + | [[Derivative of Bessel Y with respect to its order]]<br /> | ||
| + | [[Hankel H (1) in terms of csc and Bessel J]]<br /> | ||
| + | [[Hankel H (2) in terms of csc and Bessel J]]<br /> | ||
=See Also= | =See Also= | ||
Latest revision as of 15:39, 10 July 2017
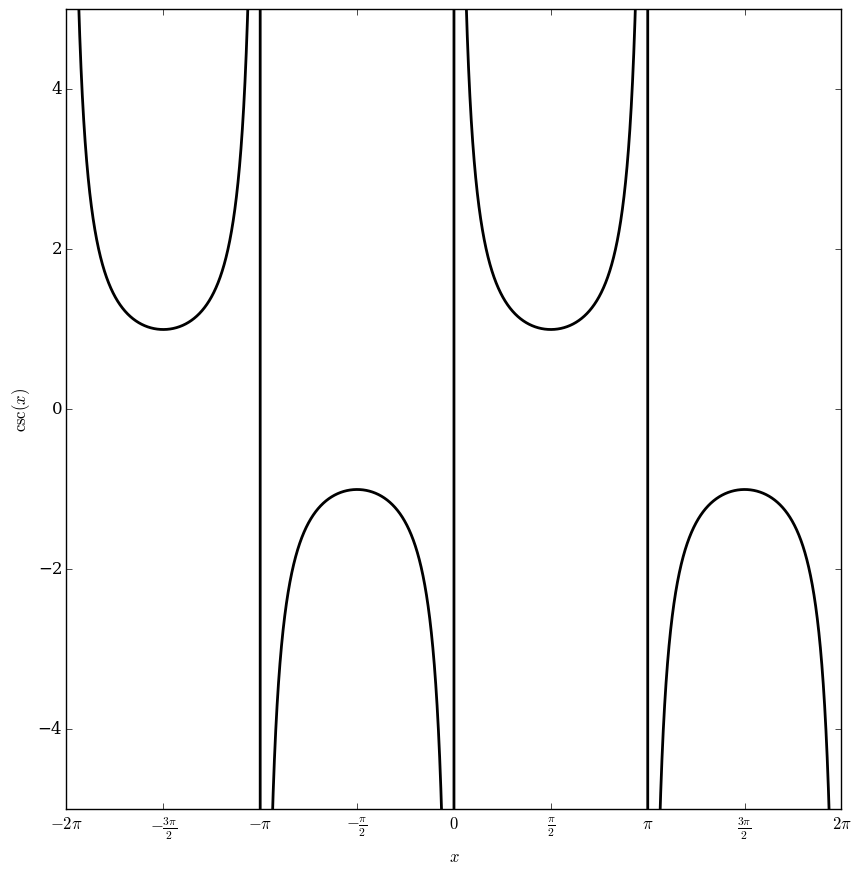
The cosecant function is defined by $$\csc(z)=\dfrac{1}{\sin(z)},$$ where $\sin$ denotes the sine function.
Graph of $\csc$ on $[-2\pi,2\pi]$.
Domain coloring of $\csc$.
Trig functions diagram using the unit circle.
Properties
Derivative of cosecant
Derivative of cotangent
Relationship between csch and csc
Relationship between csc, Gudermannian, and coth
Relationship between coth, inverse Gudermannian, and csc
Derivative of Bessel Y with respect to its order
Hankel H (1) in terms of csc and Bessel J
Hankel H (2) in terms of csc and Bessel J
See Also
References
- 1964: Milton Abramowitz and Irene A. Stegun: Handbook of mathematical functions ... (previous) ... (next): 4.3.4
