Latest revision as of 15:39, 10 July 2017

The cosecant function is defined by $$\csc(z)=\dfrac{1}{\sin(z)},$$ where $\sin$ denotes the sine function.

Properties

Trigonometric functions

Sine

Cosecant

Secant

@@ Line 1: / Line 1: @@
 The cosecant function is defined by
-$$\csc(z)=\dfrac{1}{\sin(z)}.$$
+$$\csc(z)=\dfrac{1}{\sin(z)},$$
+where $\sin$ denotes the [[sine]] function.
 <div align="center">
 <gallery>
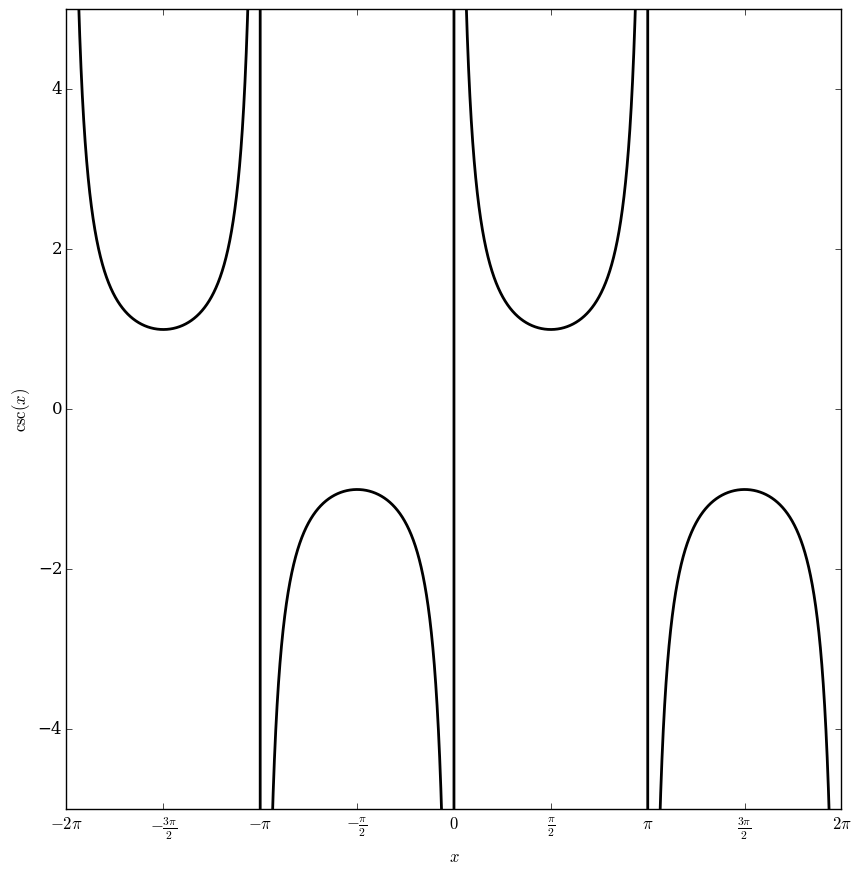
-File:Cosecant.png|Graph of $\csc$ on $\mathbb{R}$.
+File:Cosecantplot.png|Graph of $\csc$ on $[-2\pi,2\pi]$.
-File:Complex Csc.jpg|[[Domain coloring]] of [[analytic continuation]] of $\csc$.
+File:Complexcosecantplot.png|[[Domain coloring]] of $\csc$.
+File:Trig Functions Diagram.svg|Trig functions diagram using the unit circle.
 </gallery>
 </div>
 =Properties=
-{{:Derivative of cosecant}}
+[[Derivative of cosecant]] <br />
+[[Derivative of cotangent]]<br />
+[[Relationship between csch and csc]]<br />
+[[Relationship between csc, Gudermannian, and coth]] <br />
+[[Relationship between coth, inverse Gudermannian, and csc]]<br />
+[[Derivative of Bessel Y with respect to its order]]<br />
+[[Hankel H (1) in terms of csc and Bessel J]]<br />
+[[Hankel H (2) in terms of csc and Bessel J]]<br />
-<center>{{:Trigonometric functions footer}}</center>
+=See Also=
+[[Arccsc]]<br />
+[[Arccsch]] <br />
+[[Csch]] <br />
+[[Sine]]<br />
+=References=
+* {{BookReference|Handbook of mathematical functions|1964|Milton Abramowitz|author2=Irene A. Stegun|prev=Tangent|next=Secant}}: 4.3.4
+{{:Trigonometric functions footer}}
+[[Category:SpecialFunction]]
+[[Category:Definition]]