Difference between revisions of "Laguerre L"
From specialfunctionswiki
(→Properties) |
|||
| (4 intermediate revisions by the same user not shown) | |||
| Line 20: | Line 20: | ||
=Properties= | =Properties= | ||
[[Generating function for Laguerre L]]<br /> | [[Generating function for Laguerre L]]<br /> | ||
| − | + | [[L n(x)=(e^x/n!)d^n/dx^n(x^n e^(-x))]]<br /> | |
| − | + | [[L n(0)=1]]<br /> | |
| − | + | [[L n'(0)=-n]]<br /> | |
| − | + | [[Orthogonality of Laguerre L]]<br /> | |
| − | + | [[(n+1)L (n+1)(x) = (2n+1-x)L n(x)-nL (n-1)(x)]]<br /> | |
| − | + | [[xL n'(x)=nL n(x)-n L (n-1)(x)]]<br /> | |
| − | + | [[L n'(x)=-Sum L k(x)]]<br /> | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | </ | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | </ | ||
=See also= | =See also= | ||
| Line 66: | Line 32: | ||
=References= | =References= | ||
| − | * {{BookReference|Special Functions for Scientists and Engineers|1968|W.W. Bell|prev=findme|next= | + | * {{BookReference|Special Functions for Scientists and Engineers|1968|W.W. Bell|prev=findme|next=Generating function for Laguerre L}}: $(6.3)$ |
{{:Orthogonal polynomials footer}} | {{:Orthogonal polynomials footer}} | ||
[[Category:SpecialFunction]] | [[Category:SpecialFunction]] | ||
Latest revision as of 14:37, 15 March 2018
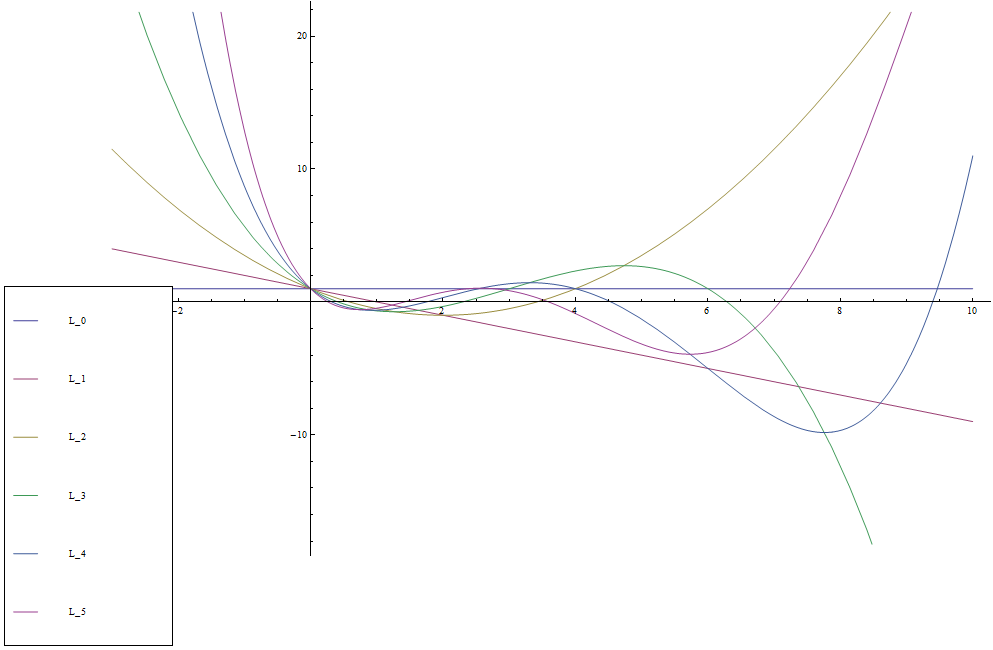
The Laguerre polynomial of order $n$, $L_n$, is given by $$L_n(x) = \displaystyle\sum_{k=0}^n \dfrac{(-1)^kn!}{(n-k)!(k!)^2}x^k.$$
The first few Laguerre polynomials are given by $$\begin{array}{ll} L_0(x) &= 1 \\ L_1(x) &= -x+1 \\ L_2(x) &= \dfrac{1}{2}(x^2-4x+2) \\ L_3(x) &= \dfrac{1}{6}(-x^3+9x^2-18x+6) \\ L_4(x) &= \dfrac{1}{24}(x^4-16x^3+72x^2-96x+24)\\ \vdots \end{array}$$
Graph of $L_n$ for $n=0,1,2,\ldots,5$ on $[-3,10]$.
Properties
Generating function for Laguerre L
L n(x)=(e^x/n!)d^n/dx^n(x^n e^(-x))
L n(0)=1
L n'(0)=-n
Orthogonality of Laguerre L
(n+1)L (n+1)(x) = (2n+1-x)L n(x)-nL (n-1)(x)
xL n'(x)=nL n(x)-n L (n-1)(x)
L n'(x)=-Sum L k(x)
See also
References
- 1968: W.W. Bell: Special Functions for Scientists and Engineers ... (previous) ... (next): $(6.3)$
