Difference between revisions of "Kelvin bei"
From specialfunctionswiki
| Line 5: | Line 5: | ||
<div align="center"> | <div align="center"> | ||
<gallery> | <gallery> | ||
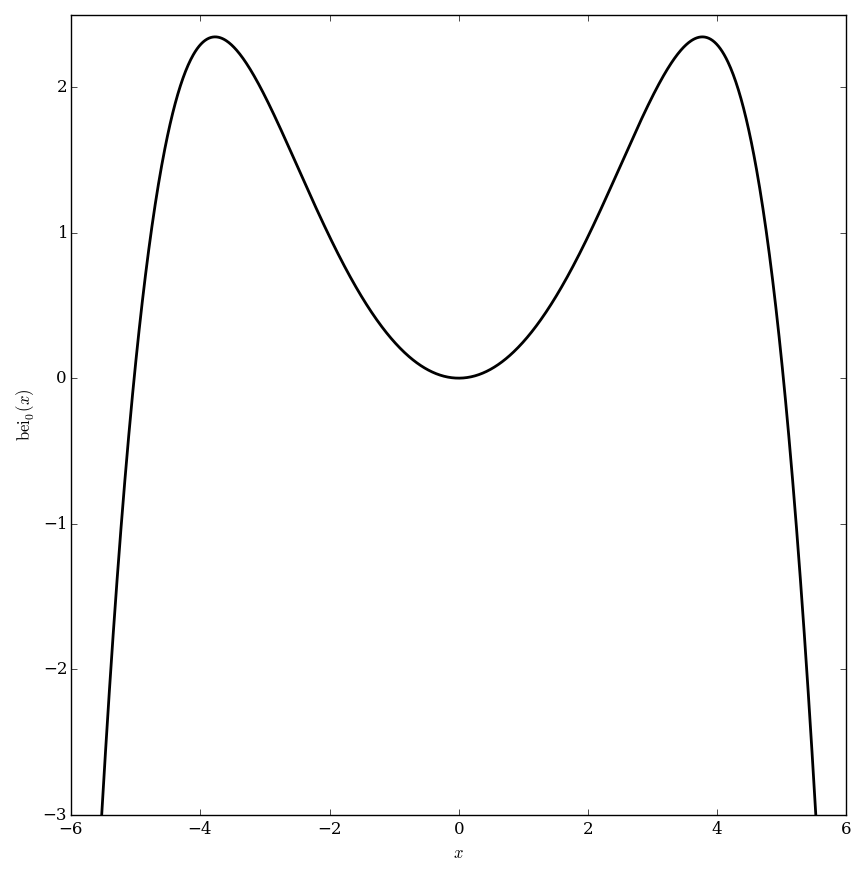
| + | File:Kelvinbei,n=0plot.png|Graph of $\mathrm{bei}_0$. | ||
File:Domcolkelvinbeisub0.png|[[Domain coloring]] of $\mathrm{bei}_0$. | File:Domcolkelvinbeisub0.png|[[Domain coloring]] of $\mathrm{bei}_0$. | ||
</gallery> | </gallery> | ||
Revision as of 00:27, 11 June 2016
The $\mathrm{bei}_{\nu}$ function is defined as $$\mathrm{bei}_{\nu}(z)=\mathrm{Im} \hspace{2pt} J_{\nu} \left( z e^{\frac{3\pi i}{4}} \right),$$ where $\mathrm{Im}$ denotes the imaginary part of a complex number and $J_{\nu}$ denotes the Bessel function of the first kind.
Graph of $\mathrm{bei}_0$.
Domain coloring of $\mathrm{bei}_0$.
