Difference between revisions of "Riemann zeta"
From specialfunctionswiki
| Line 22: | Line 22: | ||
*[http://www.claymath.org/sites/default/files/ezeta.pdf English translation of Riemann's paper "On the number of prime numbers less than a given quantity"] | *[http://www.claymath.org/sites/default/files/ezeta.pdf English translation of Riemann's paper "On the number of prime numbers less than a given quantity"] | ||
*[http://www.uam.es/personal_pdi/ciencias/cillerue/Curso/zeta2.pdf Evaluating $\zeta(2)$] | *[http://www.uam.es/personal_pdi/ciencias/cillerue/Curso/zeta2.pdf Evaluating $\zeta(2)$] | ||
| − | |||
*[http://empslocal.ex.ac.uk/people/staff/mrwatkin//zeta/riemannhyp.htm The Riemann Hypothesis: FAQ and resources] | *[http://empslocal.ex.ac.uk/people/staff/mrwatkin//zeta/riemannhyp.htm The Riemann Hypothesis: FAQ and resources] | ||
*[http://empslocal.ex.ac.uk/people/staff/mrwatkin//zeta/devlin.pdf How Euler discovered the zeta function] | *[http://empslocal.ex.ac.uk/people/staff/mrwatkin//zeta/devlin.pdf How Euler discovered the zeta function] | ||
Revision as of 06:36, 22 June 2016
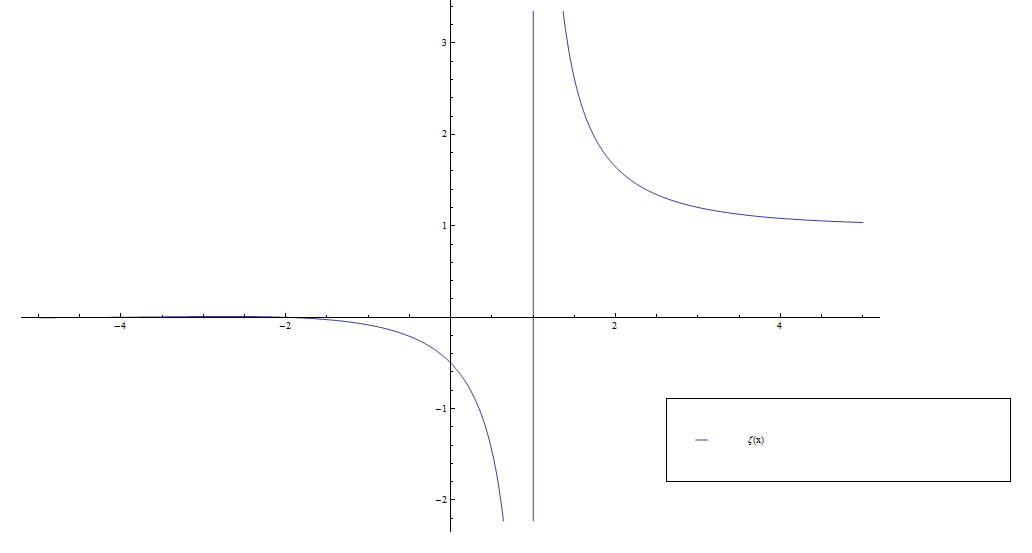
Consider the function $\zeta$ defined by the series $$\zeta(z) = \displaystyle\sum_{n=1}^{\infty} \dfrac{1}{n^z},$$ which is valid for $\mathrm{Re}(z)>1$.
Domain coloring of $\zeta$.
Properties
Euler product for Riemann zeta
Laurent series of the Riemann zeta function
Relationship between prime zeta, Möbius function, logarithm, and Riemann zeta
Videos
Riemann Zeta function playlist
External links
- 15 Videos about the Riemann $\zeta$ function
- English translation of Riemann's paper "On the number of prime numbers less than a given quantity"
- Evaluating $\zeta(2)$
- The Riemann Hypothesis: FAQ and resources
- How Euler discovered the zeta function
- Andrew Odlyzko: Tables of zeros of the Riemann zeta function
References
- 1930: Edward Charles Titchmarsh: The Zeta-Function of Riemann ... (next): § Introduction (1)