Difference between revisions of "Cosine"
From specialfunctionswiki
| Line 7: | Line 7: | ||
File:Cosineplot.png|Graph of $\cos$ on $[-2\pi,2\pi]$. | File:Cosineplot.png|Graph of $\cos$ on $[-2\pi,2\pi]$. | ||
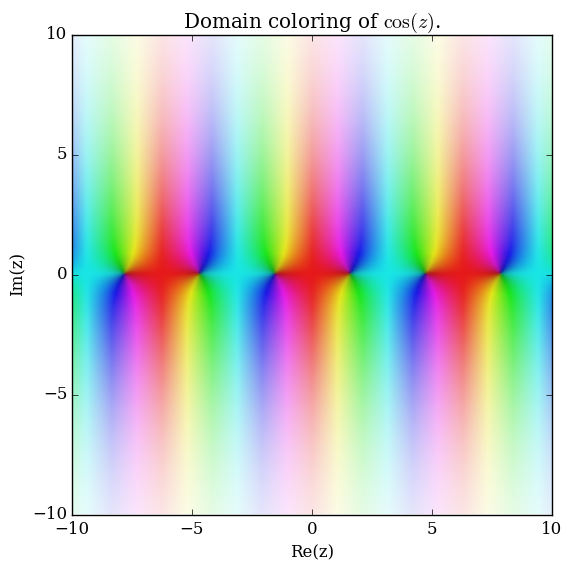
File:Complexcosineplot.png|[[Domain coloring]] of $\cos$. | File:Complexcosineplot.png|[[Domain coloring]] of $\cos$. | ||
| + | File:Trig Functions Diagram.svg|Trig functions diagram using the unit circle. | ||
</gallery> | </gallery> | ||
</div> | </div> | ||
Revision as of 06:14, 6 June 2016
The cosine function, $\cos \colon \mathbb{C} \rightarrow \mathbb{C}$ is defined by the formula $$\cos(z)=\dfrac{e^{iz}+e^{-iz}}{2},$$ where $e^z$ is the exponential function.
Domain coloring of $\cos$.
Properties
Derivative of cosine
Taylor series of cosine
Weierstrass factorization of cosine
Beta in terms of sine and cosine
Relationship between cosine and hypergeometric 0F1
Relationship between spherical Bessel y sub nu and cosine
Relationship between cosh and cos
Relationship between cos and cosh
Relationship between cosine, Gudermannian, and sech
Relationship between sech, inverse Gudermannian, and cos
See Also
References
- 1964: Milton Abramowitz and Irene A. Stegun: Handbook of mathematical functions ... (previous) ... (next): 4.3.147