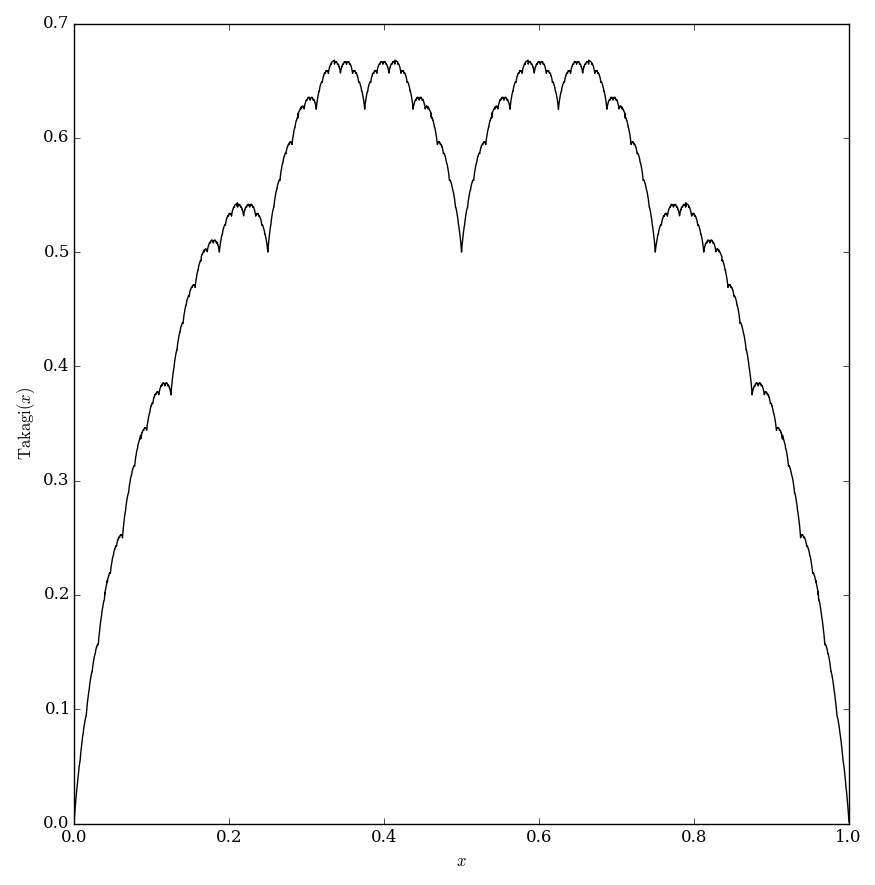
Takagi function

The Takagi function (also called the blancmange function) is defined by $$\mathrm{takagi}(x)=\displaystyle\sum_{k=0}^{\infty} \dfrac{\mathrm{dist}_{\mathbb{Z}}(2^n x)}{2^n},$$ where $\mathrm{dist}_{\mathbb{Z}}$ denotes the distance to integers function.

• 

  Graph of $\mathrm{takagi}$ on $[0,1]$.

Properties

Takagi function is continuous
Takagi function is nowhere differentiable

See Also

van der Waerden function

References

[1]
[2]

Continuous nowhere differentiable functions

Darboux function

Faber F1

Faber F2

Takagi

van der Waerden