Difference between revisions of "Kelvin ker"
From specialfunctionswiki
| Line 11: | Line 11: | ||
=References= | =References= | ||
[http://mathworld.wolfram.com/Ker.html] <br/> | [http://mathworld.wolfram.com/Ker.html] <br/> | ||
| + | |||
| + | <center>{{:Kelvin functions footer}}</center> | ||
Revision as of 03:23, 21 August 2015
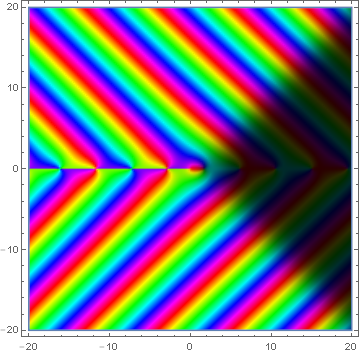
The $\mathrm{ker}_{\nu}$ function is defined as $$\mathrm{ber}(z)=\mathrm{Re} \left[ e^{-\frac{\nu \pi i}{2}} K_{\nu} \left( z e^{\frac{\pi i}{4}} \right) \right],$$ where $\mathrm{Re}$ denotes the real part of a complex number and $K_{\nu}$ denotes the modified Bessel function $K_{\nu}$.
Domain coloring of $\mathrm{ker}_0$.