Difference between revisions of "Gamma"
(→Other formulas) |
|||
| (72 intermediate revisions by the same user not shown) | |||
| Line 1: | Line 1: | ||
| − | The gamma function is the function defined by the integral | + | __NOTOC__ |
| − | $$\Gamma( | + | The gamma function $\Gamma \colon \mathbb{C} \setminus \{0,-1,-2,\ldots\} \rightarrow \mathbb{C}$ is the function initially defined for $\mathrm{Re}(z)>0$ by the integral by the formula |
| + | $$\Gamma(z)=\displaystyle\int_0^{\infty} \xi^{z-1}e^{-\xi} \mathrm{d}\xi.$$ | ||
The [[analytic continuation]] of $\Gamma$ leads to a [[meromorphic function]] with [[pole | poles]] at the negative integers. | The [[analytic continuation]] of $\Gamma$ leads to a [[meromorphic function]] with [[pole | poles]] at the negative integers. | ||
<div align="center"> | <div align="center"> | ||
<gallery> | <gallery> | ||
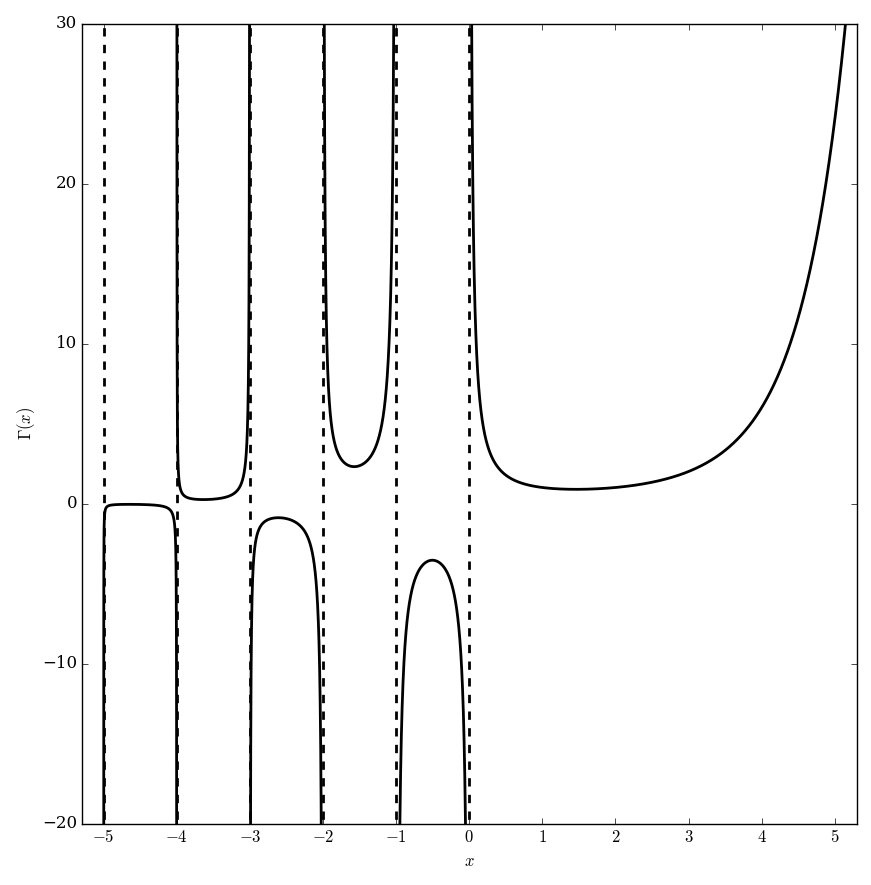
| − | File: | + | File:Gammaplot.png|Graph of $\Gamma$. |
| − | File: | + | File:Complexgammaplot.png|[[Domain coloring]] of $\Gamma$. |
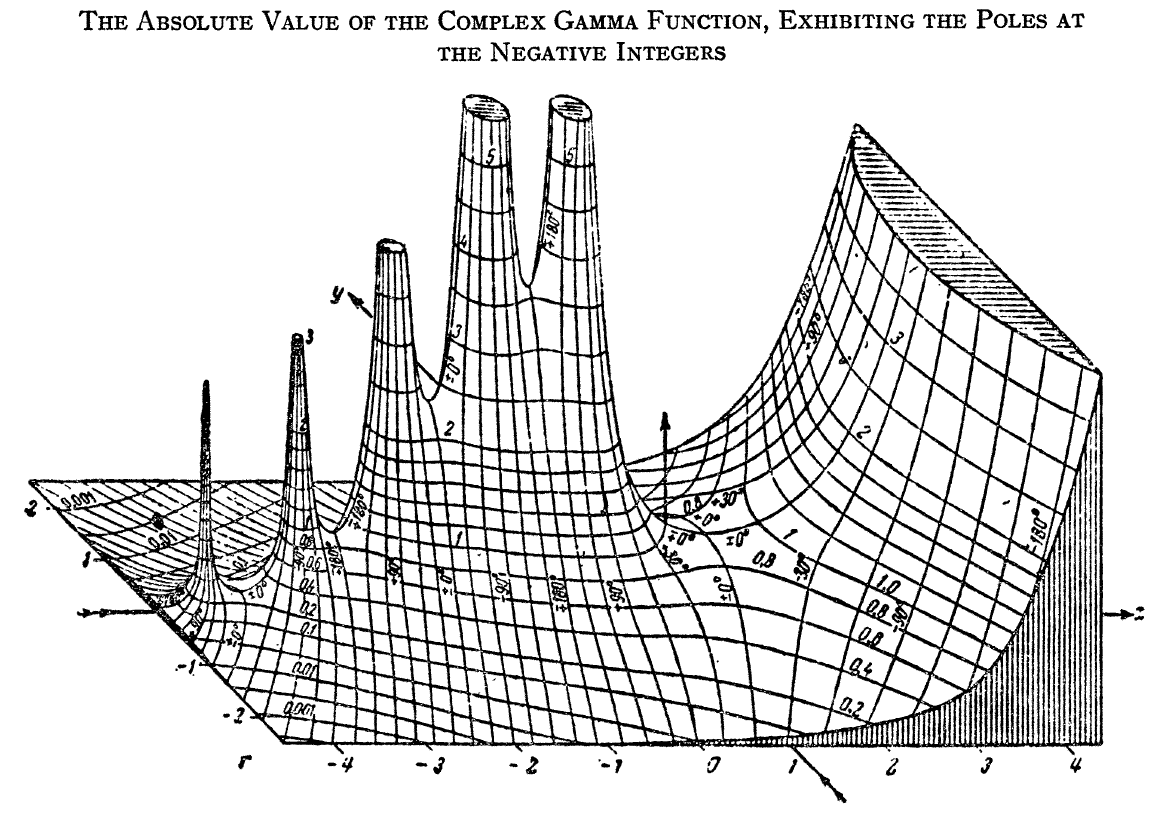
File:Absvalgamma.png|Plot of $z=|\Gamma(x+iy)|$ (1948). | File:Absvalgamma.png|Plot of $z=|\Gamma(x+iy)|$ (1948). | ||
| + | File:Gamma and reciprocal gamma (abramowitzandstegun).png|Plot of $\Gamma$ and [[Reciprocal gamma|$\dfrac{1}{\Gamma}$]] from Abramowitz&Stegun. | ||
</gallery> | </gallery> | ||
</div> | </div> | ||
| − | = | + | =Properties= |
| − | < | + | [[Gamma(z) as integral of a power of log(1/t) for Re(z) greater than 0]]<br /> |
| − | < | + | [[Gamma function written as a limit of a factorial, exponential, and a rising factorial]]<br /> |
| − | < | + | [[Gamma function written as infinite product]]<br /> |
| − | < | + | [[Gamma(1)=1]]<br /> |
| − | + | [[Gamma(z+1)=zGamma(z)]]<br /> | |
| − | + | [[Gamma(n+1)=n!]]<br /> | |
| − | + | [[Relationship between Hurwitz zeta and gamma function]]<br /> | |
| − | + | [[Gamma(z)Gamma(1-z)=pi/sin(pi z)]]<br /> | |
| − | + | [[Bohr-Mollerup theorem]]<br /> | |
| − | + | [[Gamma'(z)/Gamma(z)=-gamma-1/z+Sum z/(k(z+k))]]<br /> | |
| − | |||
| − | </ | ||
| − | + | =Videos= | |
| − | < | + | [https://www.youtube.com/watch?v=Yor2Q584o6A What's the Gamma Function? (16 September 2008)]<br /> |
| − | + | [https://www.youtube.com/watch?v=AIPgWKnBFgc Gamma Function Of One-Half: Part 1 (10 August 2010)]<br /> | |
| − | < | + | [https://www.youtube.com/watch?v=KAn0jKFkmrI Gamma Function Of One-Half: Part 2 (10 August 2010)]<br /> |
| − | + | [https://www.youtube.com/watch?v=vSekqP29PjA Gamma Integral Function - Introduction (5 December 2011)]<br /> | |
| − | + | [https://www.youtube.com/watch?v=Uos_gY--5GI gamma function - Part 1 (9 February 2012)]<br /> | |
| − | + | [https://www.youtube.com/watch?v=2iBNo4j3vRo&list=PL3E4136E122545FBE&index=1 Gamma Function (playlist) (26 February 2012)]<br /> | |
| − | + | [https://www.youtube.com/watch?v=yu9k2iPta-k Gamma function (20 October 2012)]<br /> | |
| − | + | [https://www.youtube.com/watch?v=Vc8dIykQRhY Beta Function, Gamma Function and their Properties (17 August 2013)]<br /> | |
| − | </ | + | [https://www.youtube.com/watch?v=6kSe2PnDEvM Thermodynamics 19 a : Gamma Function 1/2 (31 August 2013)]<br /> |
| − | </ | + | [https://www.youtube.com/watch?v=O45LOf2NfNI euler gamma function (14 September 2013)]<br /> |
| + | [https://www.youtube.com/watch?v=XZIVrkkYBRI The Gamma Function: intro (5) (13 February 2014)]<br /> | ||
| + | [https://www.youtube.com/watch?v=Q1rHWCP_40s The Gamma Function: why 0!=1 (5) (13 February 2014)]<br /> | ||
| + | [https://www.youtube.com/watch?v=C3PmT6oNEew Mod-04 Lec-09 Analytic continuation and the gamma function (Part I) (3 June 2014)]<br /> | ||
| + | [https://www.youtube.com/watch?v=XAoe4th0F1k Gamma function at 1/2 (3 January 2015)]<br /> | ||
| + | [https://www.youtube.com/watch?v=l7LoSBv6o2k Contour Integral Definition of the Gamma Function (18 January 2015)]<br /> | ||
| − | + | =External links= | |
| − | + | [http://ocw.mit.edu/courses/mathematics/18-104-seminar-in-analysis-applications-to-number-theory-fall-2006/projects/chan.pdf The sine product formula and the gamma function]<br /> | |
| − | + | [http://www.jstor.org/discover/10.2307/2309786?sid=21105065140641&uid=3739256&uid=2129&uid=70&uid=3739744&uid=4&uid=2 Leonhard Euler's Integral: A Historical Profile of the Gamma Function]<br /> | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | < | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | </ | ||
| − | + | =See Also= | |
| − | + | [[Loggamma]]<br /> | |
| − | + | [[Polygamma]]<br /> | |
| − | + | [[Reciprocal gamma]] <br /> | |
| − | < | ||
| − | </ | ||
| − | </ | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
=References= | =References= | ||
| − | + | * {{BookReference|Einleitung in die Theorie der Gammafunktion und der Euler'schen Integrale|1895|Johann Heinrich Graf|prev=findme|next=findme}}: $\S 3 (15_a)$ | |
| − | + | * {{BookReference|A course of modern analysis|1920|Edmund Taylor Whittaker|author2=George Neville Watson|edpage=Third edition|prev=findme|next=Euler-Mascheroni constant}}: $\S 12 \cdot 1$ | |
| − | + | * {{BookReference|Higher Transcendental Functions Volume I|1953|Arthur Erdélyi|author2=Wilhelm Magnus|author3=Fritz Oberhettinger|author4=Francesco G. Tricomi|next=Gamma(z) as integral of a power of log(1/t) for Re(z) greater than 0}}: $\S 1.1 (1)$ | |
| + | * {{BookReference|Special Functions of Mathematical Physics and Chemistry|1956|Ian N. Sneddon|prev=findme|next=Beta}}: $\S 5 (5.1)$ | ||
| + | * {{BookReference|Special Functions|1960|Earl David Rainville|prev=findme|next=findme}}: $15.(1)$ | ||
| + | * {{BookReference|Handbook of mathematical functions|1964|Milton Abramowitz|author2=Irene A. Stegun|prev=findme|next=Gauss' formula for gamma function}}: $6.1.1$ | ||
| + | * {{BookReference|Special Functions for Scientists and Engineers|1968|W.W. Bell|prev=findme|next=Beta}}: $(2.1)$ | ||
| + | * {{BookReference|The Special Functions And Their Approximations, Volume I|1969|Yudell L. Luke|prev=findme|next=findme}} $2.1 (1)$ | ||
| + | * {{BookReference|Hypergeometric Orthogonal Polynomials and Their q-Analogues|2010|Roelof Koekoek|author2=Peter A. Lesky|author3=René F. Swarttouw|prev=findme|next=Gamma(z+1)=zGamma(z)}}: $(1.2.1)$ | ||
| + | * {{BookReference|Special functions, a graduate text|2010|Richard Beals|author2=Roderick Wong|prev=findme|next=Gamma(z+1)=zGamma(z)}}: $(2.1.1)$ | ||
| + | [[Category:SpecialFunction]] | ||
Latest revision as of 18:12, 16 June 2018
The gamma function $\Gamma \colon \mathbb{C} \setminus \{0,-1,-2,\ldots\} \rightarrow \mathbb{C}$ is the function initially defined for $\mathrm{Re}(z)>0$ by the integral by the formula $$\Gamma(z)=\displaystyle\int_0^{\infty} \xi^{z-1}e^{-\xi} \mathrm{d}\xi.$$ The analytic continuation of $\Gamma$ leads to a meromorphic function with poles at the negative integers.
Domain coloring of $\Gamma$.
Plot of $\Gamma$ and $\dfrac{1}{\Gamma}$ from Abramowitz&Stegun.
Properties
Gamma(z) as integral of a power of log(1/t) for Re(z) greater than 0
Gamma function written as a limit of a factorial, exponential, and a rising factorial
Gamma function written as infinite product
Gamma(1)=1
Gamma(z+1)=zGamma(z)
Gamma(n+1)=n!
Relationship between Hurwitz zeta and gamma function
Gamma(z)Gamma(1-z)=pi/sin(pi z)
Bohr-Mollerup theorem
Gamma'(z)/Gamma(z)=-gamma-1/z+Sum z/(k(z+k))
Videos
What's the Gamma Function? (16 September 2008)
Gamma Function Of One-Half: Part 1 (10 August 2010)
Gamma Function Of One-Half: Part 2 (10 August 2010)
Gamma Integral Function - Introduction (5 December 2011)
gamma function - Part 1 (9 February 2012)
Gamma Function (playlist) (26 February 2012)
Gamma function (20 October 2012)
Beta Function, Gamma Function and their Properties (17 August 2013)
Thermodynamics 19 a : Gamma Function 1/2 (31 August 2013)
euler gamma function (14 September 2013)
The Gamma Function: intro (5) (13 February 2014)
The Gamma Function: why 0!=1 (5) (13 February 2014)
Mod-04 Lec-09 Analytic continuation and the gamma function (Part I) (3 June 2014)
Gamma function at 1/2 (3 January 2015)
Contour Integral Definition of the Gamma Function (18 January 2015)
External links
The sine product formula and the gamma function
Leonhard Euler's Integral: A Historical Profile of the Gamma Function
See Also
Loggamma
Polygamma
Reciprocal gamma
References
- 1895: Johann Heinrich Graf: Einleitung in die Theorie der Gammafunktion und der Euler'schen Integrale ... (previous) ... (next): $\S 3 (15_a)$
- 1920: Edmund Taylor Whittaker and George Neville Watson: A course of modern analysis ... (previous) ... (next): $\S 12 \cdot 1$
- 1953: Arthur Erdélyi, Wilhelm Magnus, Fritz Oberhettinger and Francesco G. Tricomi: Higher Transcendental Functions Volume I ... (next): $\S 1.1 (1)$
- 1956: Ian N. Sneddon: Special Functions of Mathematical Physics and Chemistry ... (previous) ... (next): $\S 5 (5.1)$
- 1960: Earl David Rainville: Special Functions ... (previous) ... (next): $15.(1)$
- 1964: Milton Abramowitz and Irene A. Stegun: Handbook of mathematical functions ... (previous) ... (next): $6.1.1$
- 1968: W.W. Bell: Special Functions for Scientists and Engineers ... (previous) ... (next): $(2.1)$
- 1969: Yudell L. Luke: The Special Functions And Their Approximations, Volume I ... (previous) ... (next) $2.1 (1)$
- 2010: Roelof Koekoek, Peter A. Lesky and René F. Swarttouw: Hypergeometric Orthogonal Polynomials and Their q-Analogues ... (previous) ... (next): $(1.2.1)$
- 2010: Richard Beals and Roderick Wong: Special functions, a graduate text ... (previous) ... (next): $(2.1.1)$